A hemispherical portion of radius R is removed from the bottom of a cylinder of radius R. The volume of the remaining cylinder is V and its mass M. It is suspended by a string in a liquid of density ρ where it stays vertical. The upper surface of the cylinder is at a depth h below the liquid surface. The force on the bottom of the cylinder by the liquid is
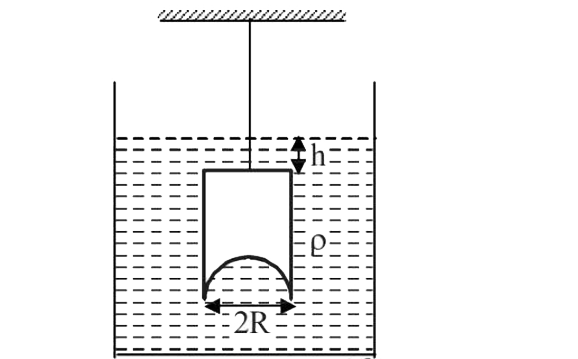
Mg
Mg−vρg
Mg+πR2hρg
ρg(V+πR2h)
The correct Answer is:D
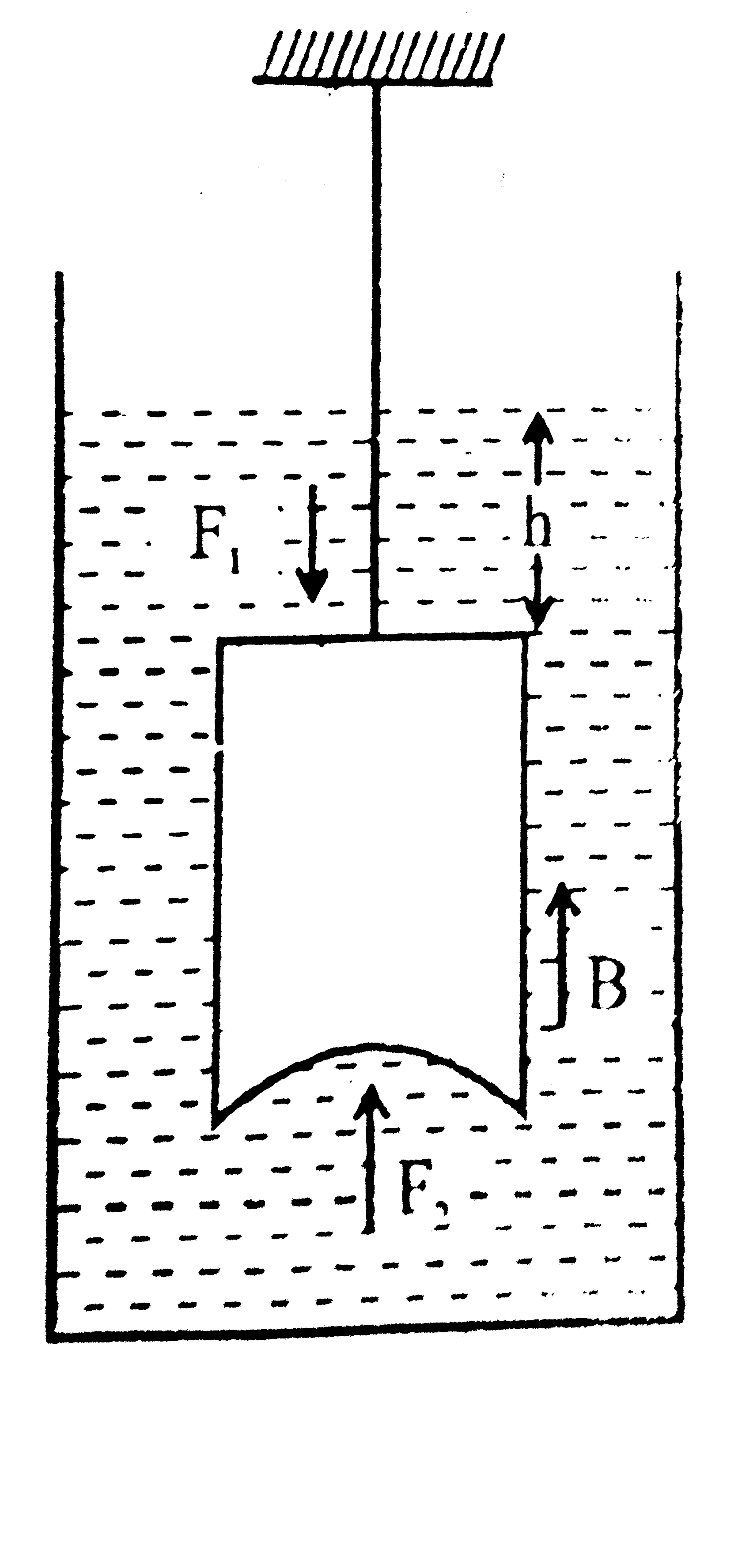
B=F2−F1
F2=B+F1
F2=Vρg+(hρg×πR2)
F2=ρg(V+πR2h)
Topper's Solved these Questions
- FLUID MECHANICSBook:BANSALChapter:FLUID MECHANICSExercise:MCQ & Solution
Explore 86 Videos
- FLUID MECHANICSBook:BANSALChapter:FLUID MECHANICSExercise:PYQS AIEEE
Explore 10 Videos
- FLUID MECHANICSBook:BANSALChapter:FLUID MECHANICSExercise:EXERCISE # II
Explore 18 Videos
- MASTER PRACTICE PROBLEMBook:BANSALChapter:MASTER PRACTICE PROBLEMExercise:Additional topic
Explore 70 Videos
Similar Questions
Knowledge Check
A hemispherical portion of radius R is removed from the bottom of a cylinder of radius R. The volume of the remaining cylinder is V and its mass is M. It suspended by a string in a liquid of density ρ where it stays vertical. The upper surface of cylinder is at a depth h below the liquid surface. The force on the bottom of the cylinder bby the liquid is
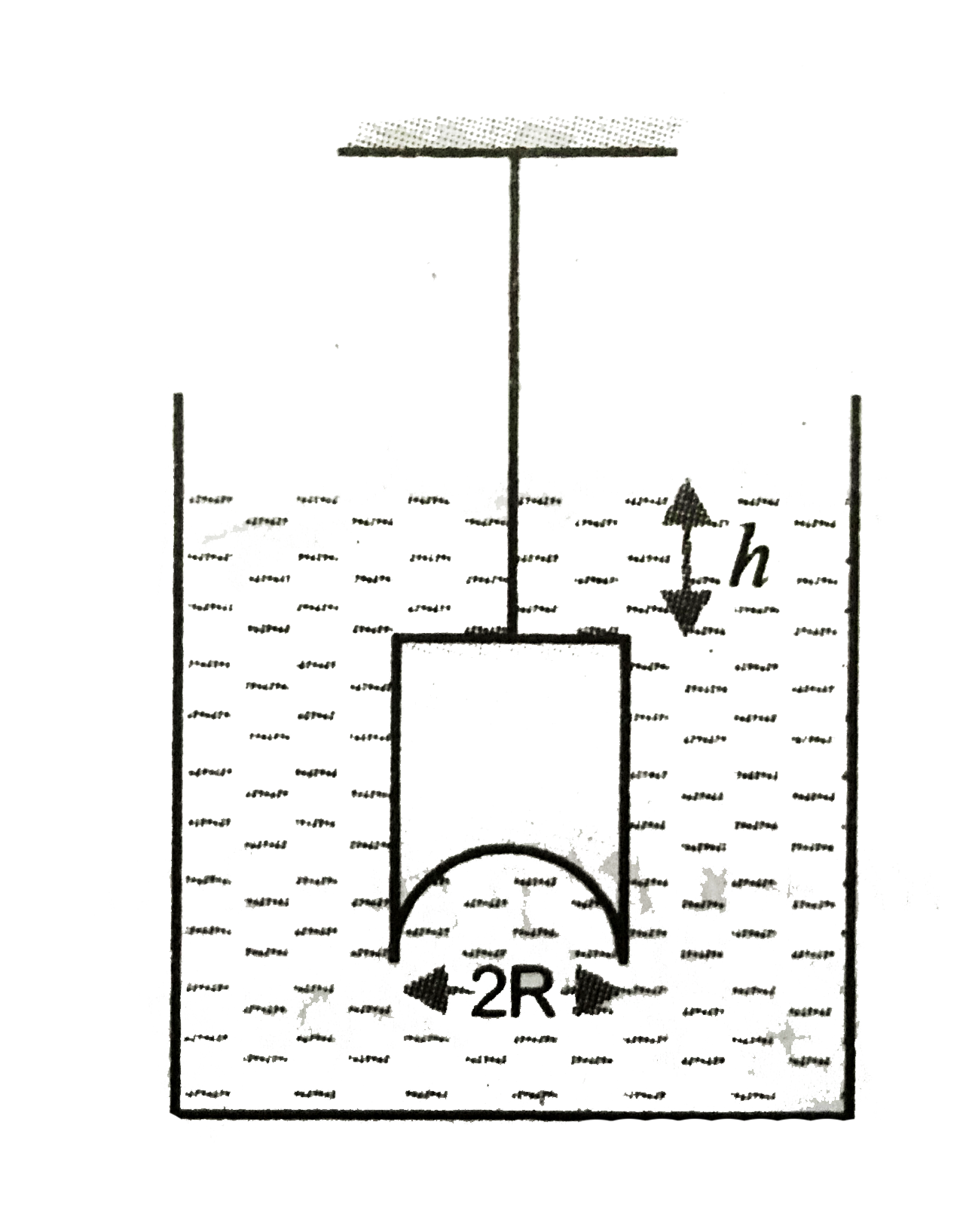 AMgBMg - h ρ gCMg+πR2hρgDρg(V+πR2h)
AMgBMg - h ρ gCMg+πR2hρgDρg(V+πR2h)A hemispherical portion of radius R is removed from the bottom of a cylinder of radius R. The volume of the remaining cylinder is V and its mass M. It is suspended by a string in a liquid of density ρ where it stays vertical. The upper surface of the cylinder is at a depth h below the liquid surface. The force on the bottom of the cylinder by the liquid is
 AMgBMg-VdgCMg+πR2hdgDdg(V+πR2h)
AMgBMg-VdgCMg+πR2hdgDdg(V+πR2h)A conical portion of radius R and height H is removed from the bottom of a cylinder of radius R. The volume of the remaining cylinder is V and its mass is M. It is suspended by a string in a liquid of density ρ where it stays vertical . The upper surface of the cylinder is at depth h below the liquid surface . The force on the bottom of the cylinder by the liquid is
 AMgBMg−VρgCMg+πR2hρgDρg(V+πR2h)
AMgBMg−VρgCMg+πR2hρgDρg(V+πR2h)
Curved surface area of a cylinder of radius h and height r is _______.
View SolutionThe sum of radius of the base and height of a cylinder is 37m. If the total surface area of the cylinder is 1628m2, find the curved surface area and volume of the cylinder
View SolutionA cylinder is inscribed in a sphere of radius r
What is the radius of the cylinder of maximum volume ?
View SolutionThe volumes of a sphere and a right circular cylinder having the same radius are equal. The ratio of the diameter of the sphere to the height of the cylinder is
View SolutionA sphere is placed inside a right circular cylinder so as to touch the top, base and lateral surface of the cylinder. If the radius of the sphere is r, then the volume of the cylinder is 4π r3 (b) 83π r3 (c) 2π r3 (d) 8π r3
View Solution