A 3.6m long vertical pipe resonates with a source of frequency 212.5Hz when water level is at certain height in the pipe. Find the height of water level (from the bottom of the pipe) at which resonance occurs. Neglect end correction. Now , the pipe is filled to a height H(≈3.6m). A small hole is drilled very close to its bottom and water is allowed to leak. Obtain an expression for the rate of fall of water level in the pipe as a function of H. If the radii of the pipe and the hole are 2×10−2m and 1×10−3m respectively, Calculate the time interval between the occurance of first two resonances. Speed of sound in air 340m/s and g=10m/s2.

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:h=3.2,2.4,1.6,0.8,0;dHdt=5×10−3√5H;Δt=80(4−2√3)

First resonance length
v=nλ
340=212.5×l1
l1=0.4m
x1=3.6−0.4
x1=3.2m
Second resonance length
V=nλ2⇒340=212.5×4l23
l2=1.2m
x2=3.6−1.2
x2=2.4m Third resonance length
v=nλ⇒340=212.5×45l3
l3=2m⇒x3=3.6−2⇒x3=1.6m
forth resonance length
v=n⇒340=212.5×4l47
l4=2.8m⇒x4=3.6−2.8⇒x4=0.8m
Given that at any stage height of liquid above hole is H.
First we have to get time taken
velocity of efflux v=√2gH
volume comingout per/sec. dVdt=A0√2gH
−AdHdt=A0√2gH
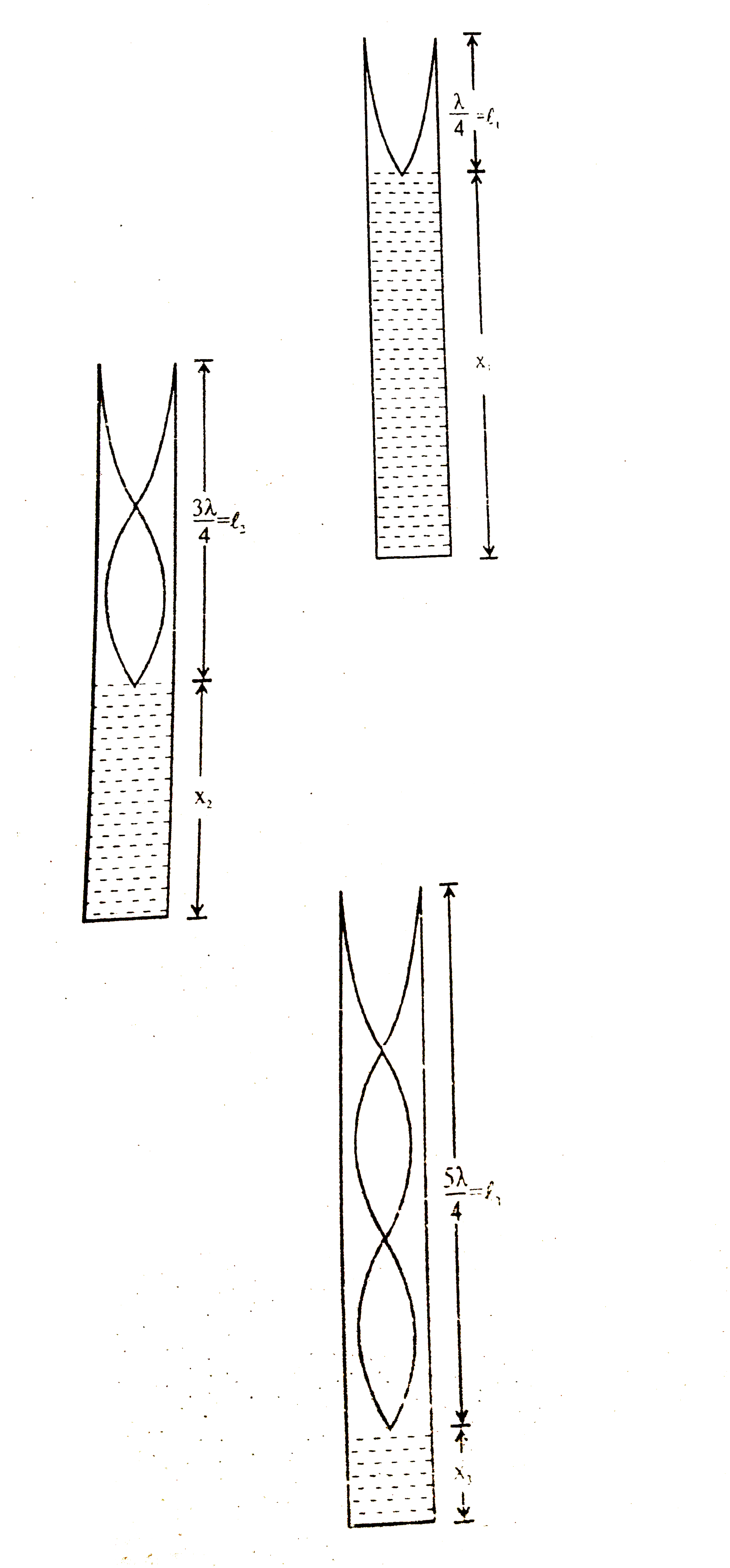
ocr_image
dHdt=A0A√2gH ⇒dHdt=1×10−32×10−2√2×10×H
dHdt=π×(1×10−3)2π×(2×10−2)2√2×10×H ⇒dHdt=5×10−3√5H
For time interval
dVdt=A0√2gH
−AdHdt=A0√2gH ⇒−AdH=A0√2gHdt
−AA0x∫Hdx√x=√2gt∫0dt ⇒−AA0[√2x]xH=√2gt
AA0√2g⌊√x−√H⌋=t
t=AA0√2g⌊√H−√x⌋
for first resonance
t1=AA0√2g⌊√H−√H1⌋
for second resonance
t2=AA0√2g⌊√H−√H2⌋ so,Δt=AA0√2g⌊√H1−√H2⌋
Δt=π(2×10−2)2π(1×10−3)2√210(√3.2−√2.4)
Δt=400√15(√165−√125)
Δt=80(4−2√3)sec.

Updated on:21/07/2023
|
Class 12PHYSICSFLUID MECHANICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • An air column in a resonace tube of length 1.5 m resonates with a source of frequency 125 Hz, when the water level in it at a certain height from the bottom of the tube. What is the height of water level corresponding to the fundamental frequency ?
    ( Neglect the end correction and speed of sound in air is 330 m/s)

    A1.2 m
    B0.5 m
    C0.84 m
    D0.42 m

  • An open pipe resonates to a frequency f1 and a closed pipe resonates to a frequency f2. If they are joined together to form a longer tube, then it will resonate to a frequency of (neglect end corrections)

    Af1f22f2+f1
    Bf1f2f2+2f1
    C2f1f2f2+f1
    Df1+2f2f1f2

  • A closed pipe resonates at its fundmental frequency of 300Hz. Which one of the following statements is wrong ?

    Aif the temperature rises, the fundamental frequency increases.
    Bif the pressure rises, the fundamental frequency increases.
    CThe first overtone is of frequency 900Hz.
    DAn open pipe with the same fundamental frequency has twice the length.