Resistance R,2R,4R,8R ...... ∞ are connected in parallel. What is their resutlant resistance will be
To find the resultant resistance of resistors R, 2R, 4R, 8R, ..., connected in parallel, we can follow these steps:
1. Identify the Resistors: The resistors are R, 2R, 4R, 8R, ..., and they are connected in parallel.
2. Use the Formula for Parallel Resistance: The formula for the total or resultant resistance Rnet in parallel is given by:
1Rnet=1R1+1R2+1R3+…
In this case, the resistors can be expressed as:
R1=R,R2=2R,R3=4R,R4=8R,…
3. Express the Series: The series of resistances can be written as:
1Rnet=1R+12R+14R+18R+…
4. Factor Out 1R: We can factor out 1R from the equation:
1Rnet=1R(1+12+14+18+…)
5. Recognize the Series as a Geometric Series: The series inside the parentheses is a geometric series where the first term a=1 and the common ratio r=12. The sum of an infinite geometric series can be calculated using the formula:
S=a1−r
Substituting the values:
S=11−12=112=2
6. Substitute Back into the Equation: Now substituting back into the equation for 1Rnet:
1Rnet=1R⋅2=2R
7. Calculate Rnet: Finally, we take the reciprocal to find Rnet:
Rnet=R2
Final Result:
The resultant resistance Rnet of the resistors connected in parallel is:
Rnet=R2
1Rnet=1R1+1R2+1R3+…
In this case, the resistors can be expressed as:
R1=R,R2=2R,R3=4R,R4=8R,…
1Rnet=1R+12R+14R+18R+…
1Rnet=1R(1+12+14+18+…)
S=a1−r
Substituting the values:
S=11−12=112=2
1Rnet=1R⋅2=2R
Rnet=R2
The resultant resistance Rnet of the resistors connected in parallel is:
Rnet=R2
Topper's Solved these Questions
Similar Questions
Knowledge Check
Two resistors of resistance R1 and R2 having R1>R2 are connected in parallel. For equivalent resistance R, the correct statement is
AR>R1+R2BR1<R<R2CR2<R<(R1+R2)DR<R1A wire of resistance R1 is cur into five equal pieces. These five pieces of wire are then connected in parallel. If the resultant resistance of this combination be R2, then the ratio R1R2 is:
A125B15C5D25R is the equivalent resistance of n resistors of same resistance in series. If the connection is turned parallel then equivalent resistance will be:
An2RBnRCRnDRn2
The resultant resistance of n wires each of resistance r ohms is R, when they are connected in parallel. When these n resistances are connected in series, the resultant resistance will be
View Solution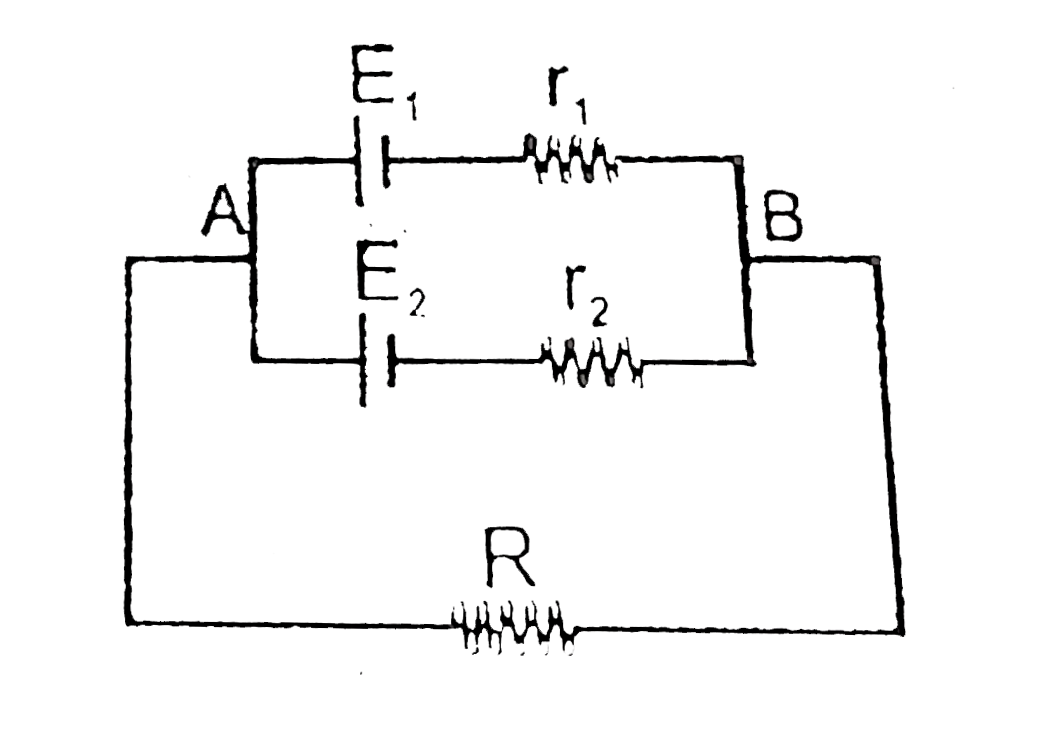
Two batteries of E1 internal resistance r1 & emf E2 internal resistance r2 are connected in parallel and makes a battery system. An external resistance R is connected across this system as shown in the figure.
View SolutionA student did the experiment to find the equivalent resistance of two given resistors, R1 and R2 first when they are connected in series and next when they are connected in parallel. The two values of the equivalent resistance obtained by him were Rs and Rp respectively. Compare Rs and Rp.
View SolutionA student did the experiment to find the equivalent resistance of two given resistors, R1 and R2 first when they are connected in series and next when they are connected in parallel. The two values of the equivalent resistance obtained by him were Rs and Rp respectively. Compare Rs and Rp.
View SolutionA resistance R2 is connected in parallel with a resistance R1 what resistance R3 must be connected in series with the combination of R1andR2 so that the equivalent resistance is equal to the resistance R1 -
View Solution