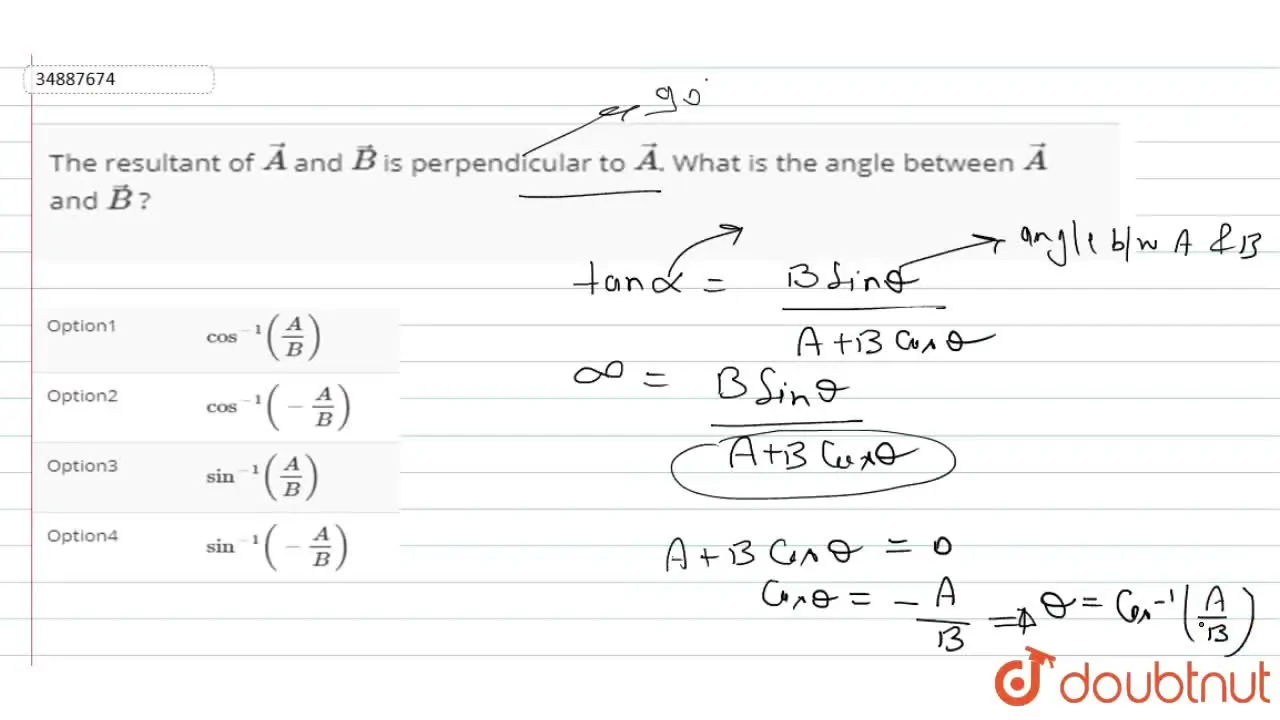
The resultant of →A and →B is perpendicular to →A. What is the angle between →A and →B ?
cos−1(AB)
cos−1(−AB)
sin−1(AB)
sin−1(−AB)
The correct Answer is:B
tanα=BsinθA+Bcosθ=tan90∘=∞
thus, A+Bcosθ=0⇒cosθ=−A/B
Related Playlists
- BOOK - ALLENCHAPTER - BASIC MATHSEXERCISE - EXERCISE-280videos
BASIC MATHS
- BOOK - ALLENCHAPTER - BASIC MATHSEXERCISE - EXERCISE-320videos
BASIC MATHS
- BOOK - ALLENCHAPTER - BASIC MATHSEXERCISE - Question1videos
BASIC MATHS
- BOOK - ALLENCHAPTER - AIIMS 2019EXERCISE - PHYSICS40videos
AIIMS 2019
- BOOK - ALLENCHAPTER - CIRCULAR MOTIONEXERCISE - EXERCISE (J-A)6videos
CIRCULAR MOTION
Similar Questions
What is the angle between A and B (vecA+vecB) = (vecA-vecB)?
View SolutionGiven that A=B. What is the angle between (→A+→B) and (→A−→B) ?
View SolutionGiven that A=B. What is the angle between (→A+→B)and(→A−→B) ?
View SolutionIf →A+→B=→R and 2→A+→B is perpendicular to →B then
View SolutionThe resultant of two vectors →A and →B is perpendicular to →A and equal to half of the magnitud of →B. Find angle between →B and →B?
View SolutionIf →a,→b are unit vectors such that the vector →a+3→b is peependicular to 7→a−→band→a−4→b is prependicular to 7→a−2→b then the angle between →aand→b is
View SolutionIf →a and →b are two unit vectors such that →a+2→b and 5→a−4→b are perpendicular to each other, then the angle between →a and →b is
View SolutionIf (→a+3→b) is perpendicular to (7→a−5→b) and (→a−4→b) is perpendicular to (7→a−2→b) , then the angle between →aand→b ( in degrees ) is ______
View SolutionLet →a,→band→c be three unit vectors such that →a is perpendicular to the plane of →b and →c. if the angle between →band→c is π3, then ∣∣→a×→b−→a×→c∣∣2 is equal to
View SolutionThe resultant of →Aand→B makes an angle alpha with →A and βand→B,
View Solution