A light spiral spring supports 200g weight at its lower end oscillates with a period of 1s. The weight that must be removed from the lower end to reduce the period to 0.5s is
100 g
50 g
150 g
200 g
The correct Answer is:C
T2T1=√m2m1⇒0.51=√200−m200
⇒m=150g must be removed
Topper's Solved these Questions
- OSCILLATIONSBook:NARAYNAChapter:OSCILLATIONSExercise:EXERCISE - II (C.W)
Explore 27 Videos
- OSCILLATIONSBook:NARAYNAChapter:OSCILLATIONSExercise:EXERCISE - II (H.W)
Explore 19 Videos
- OSCILLATIONSBook:NARAYNAChapter:OSCILLATIONSExercise:EXERCISE - I (C.W)
Explore 63 Videos
- NEWTONS LAWS OF MOTIONBook:NARAYNAChapter:NEWTONS LAWS OF MOTIONExercise:PASSAGE TYPE QUESTION
Explore 6 Videos
- PHYSICAL WORLDBook:NARAYNAChapter:PHYSICAL WORLDExercise:C.U.Q
Explore 10 Videos
Similar Questions
A srting of length l hangs freely from a rigid support under its own weight Calculate the time required by transverse waves to travel from the lower end to the upper end
View SolutionA flat spiral spring of force constant k is loaded with mass M and oscillate about vertical with a time period T. Then the mass suspended to the free end is
View Solution
Knowledge Check
A light sprial spring supports 200g weight at its lower end oscillates with a period of 1s. The weight that must be removed from the lower end to reduce the period to 0.5s is
A100gB50gC150gD200gA flat spiral spring of force constant K is loaded with mass M and oscillates vertically with a time period T. Then the mass suspended to the free end is
A4π2KT2BKT24π2CKT4π2DKT4πA mass suspended on a vertical spring oscillates with a period of 0.5s. When the mass is allowed to hang at rest, the spring is stretched by
A3.1cmB6.2cmC9.3cmD12.4cm
Three mass 0.1 kg ,0.3 kg and 0.4 kg are suspended at end of a spring. When is 0.4 kg mass is removed , the system oscillates with a period 2 s . When the 0.3 kg mass is also removed , the system will oscillates with a period
View SolutionThree masses of 500g, 300g and 100g are suspended at the end of a spring as shown and are in equilibrium. When the 500g mass is removed suddenly, the system oscillates with a period of 2s. When the 300g mass is also removed, it will oscillate with period T. Find the value of T.
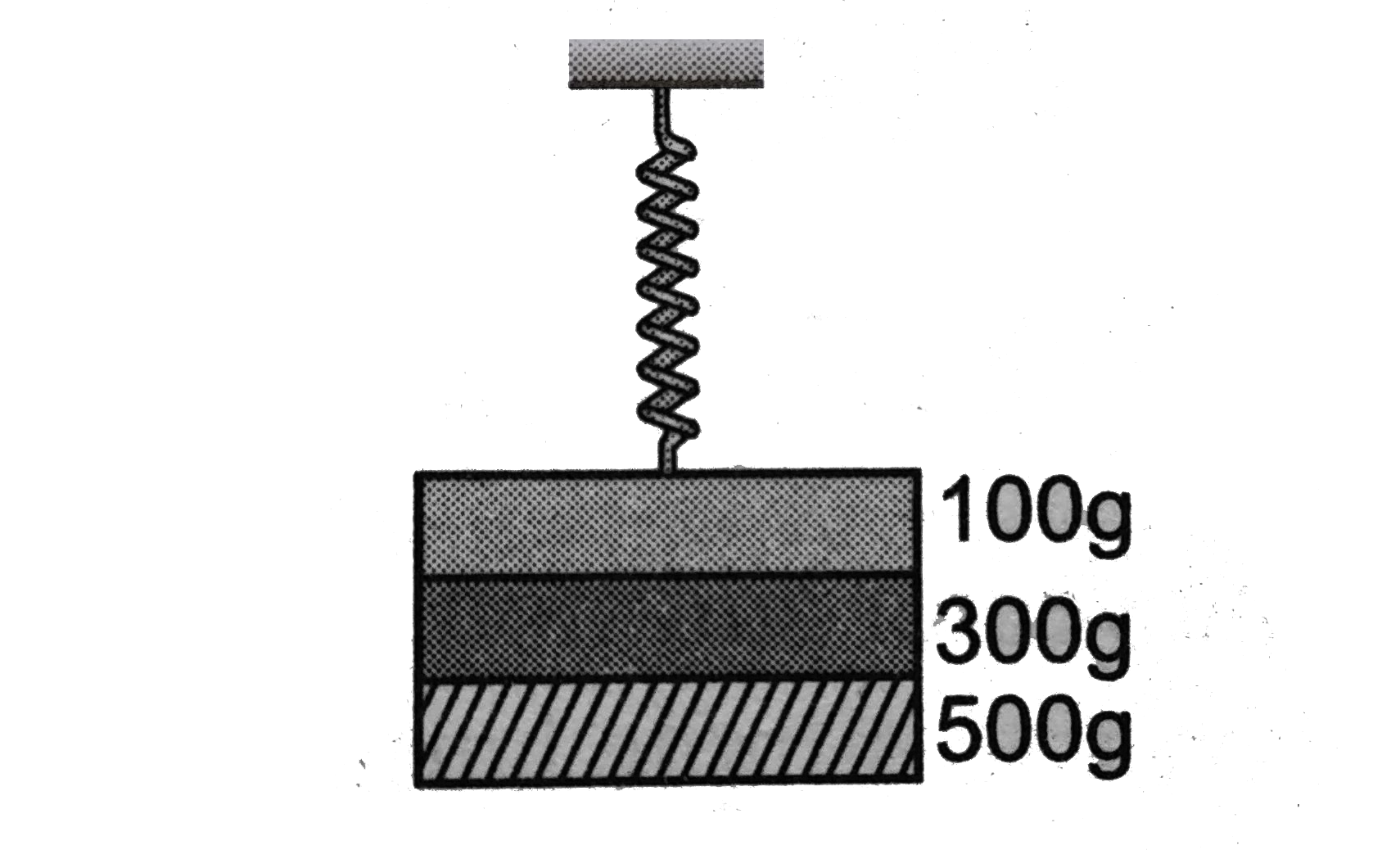
View Solution1 kg weight is suspended to a weightless spring and it has time period T. If now 4 kg weight is suspended from the same spring the time period will be
View SolutionA mass m attached to a light spring oscillates with a period of 2 s. If the mass is increased by 2 kg, the period increases by 1 s. Then the value of m is
View SolutionThree masses 700 g , 500 g , and 400 g are suspended at the end of a spring a shown and are in equilibrium. When the 700 g mass is removed, the system oscillates with a period of 3 seconds, when the 500 gm mass also removed. It will oscillate with a period of
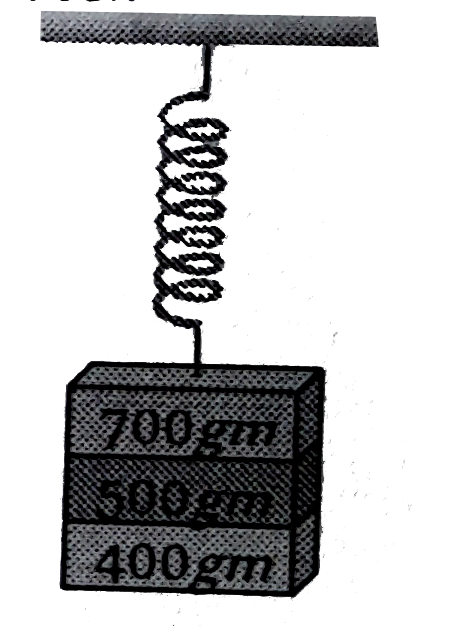
View Solution
