Two satellite of masses 40kg, 50kg are revolving around earth in different circular orbits of radii r1,r2 such that their kinetic energies are equal. The ratio of r1,r2 is
√5:√4
16:25
√45:√4
25:16
The correct Answer is:A
To solve the problem, we need to find the ratio of the radii r1 and r2 of the orbits of two satellites that have equal kinetic energies.
1. Understand the Kinetic Energy Formula:
The kinetic energy (KE) of a satellite in orbit is given by the formula:
KE=GMm2r
where G is the gravitational constant, M is the mass of the Earth, m is the mass of the satellite, and r is the radius of the orbit.
2. Set Up the Equation for Kinetic Energies:
For the two satellites with masses m1=40kg and m2=50kg, and their respective orbital radii r1 and r2, we can write:
KE1=GMm12r1
KE2=GMm22r2
Since the kinetic energies are equal, we have:
GMm12r1=GMm22r2
3. Cancel Common Terms:
We can cancel G and M from both sides of the equation:
m1r1=m2r2
4. Substitute the Masses:
Substitute m1=40kg and m2=50kg:
40r1=50r2
5. Cross Multiply:
Cross multiplying gives us:
40r2=50r1
6. Rearrange to Find the Ratio:
Rearranging the equation to find the ratio r1r2:
r1r2=4050=45
Final Answer:
The ratio of the radii r1 to r2 is:
r1r2=45
The kinetic energy (KE) of a satellite in orbit is given by the formula:
KE=GMm2r
where G is the gravitational constant, M is the mass of the Earth, m is the mass of the satellite, and r is the radius of the orbit.
For the two satellites with masses m1=40kg and m2=50kg, and their respective orbital radii r1 and r2, we can write:
KE1=GMm12r1
KE2=GMm22r2
Since the kinetic energies are equal, we have:
GMm12r1=GMm22r2
We can cancel G and M from both sides of the equation:
m1r1=m2r2
Substitute m1=40kg and m2=50kg:
40r1=50r2
Cross multiplying gives us:
40r2=50r1
Rearranging the equation to find the ratio r1r2:
r1r2=4050=45
The ratio of the radii r1 to r2 is:
r1r2=45
Topper's Solved these Questions
- GRAVITATIONBook:NARAYNAChapter:GRAVITATIONExercise:EXERCISE -II (C.W.)
Explore 38 Videos
- GRAVITATIONBook:NARAYNAChapter:GRAVITATIONExercise:EXERCISE -II (H.W.)
Explore 34 Videos
- GRAVITATIONBook:NARAYNAChapter:GRAVITATIONExercise:EXERCISE -I (C.W.)
Explore 50 Videos
- FRICTIONBook:NARAYNAChapter:FRICTIONExercise:Passage type of questions I
Explore 6 Videos
- KINETIC THEORY OF GASESBook:NARAYNAChapter:KINETIC THEORY OF GASESExercise:LEVEL-III(C.W)
Explore 52 Videos
Similar Questions
Two satellites of masses 50kg and 150kg revolve around the earth in circular orbits of radii 4R and 9Rrespectively where R is the radius of the earth. The speed of the two satellites will be in the ratio ?
View SolutionA satellite of mass , os revolving round the eartj at height of 10R, where R is the radius of earth. What is the kinetic energy of satellite.
View Solution
Knowledge Check
Two satellite of masses 400kg,500kg are revolving around earth in different circular orbits of radii r1,r2 such that their kinetic energies are equal. The ratio of r1 to r2 is
A4:5B16:25C5:4D25:16Two satellites of same mass are orbiting round the earth at heights of r1 and r2 from the centre of earth. Their kinetic energies are in the ratio of :
Ar2r1Br1r2Cr1(r1+r2)Dr2(r1+r2)Two satellite fo masses m and 4m orbit the earth in circular orbits of radii 4r and r respectively. The ratio of their orbital speed is
A1B12C1√2D1√5
Two satellites of masses 3 m and m orbit the earth in circular orbits of radii r and 3 r respectively. The ratio of the their speeds is
View SolutionTwo bodies of masses 8 kg and 4 kg are moving in concentric circular orbits of radii r1andr2 respectively . If their time periods are same , the ration of their centripetal accelerations is
View SolutionTwo satellites A and B are revolving around the earth circular orbits of radius r1 and r2 respectively with r1<r2. Plane of motion of the two are same. At position 1, A is given an impulse in the direction of velocity by firing a rocket so that it follows an elliptical path to meet B at position 2 as shown. A?t position 2, A is given another impluse so that velocities of A and B at 2 become equal and the move together.
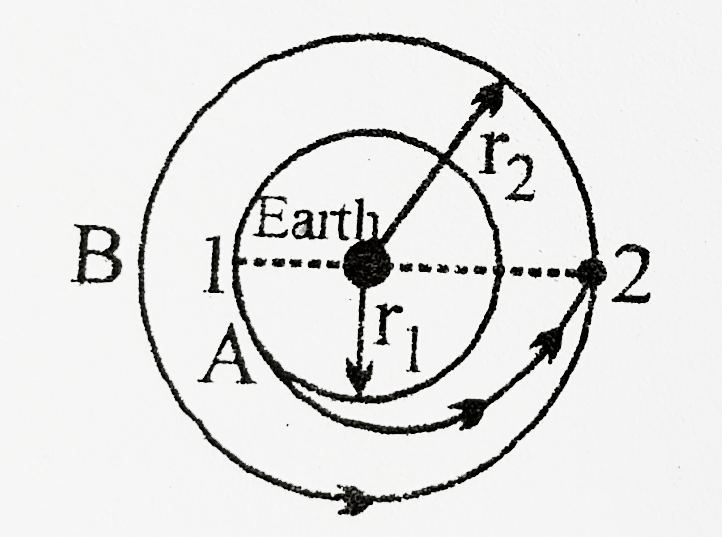
For any elliptical path of the satellite of the time period of revolution is given by Kepler's planetary law as T2αr3 where a is semi major axis of the ellipse which is r1+r22 in this case. Also angular mopmentum of any satellite revolving around the Earth will remain a constant about EArth's centre as force of gravity on the satellite which keeps it in elliptical path is along its position vector relative to the earth centre.
If the two have same mass
View SolutionTwo satellites of masses M and 4M are orbiting the earth in a circular orbit of radius r. Their frequencies of revolution are in the ratio of
View SolutionTwo satellites of masses 50 kg and 100 kg revolve around the earth in circular orbits of radii 9 R and 16 R respectively, wehre R is the radius of earth. The speeds of the two satellites will be in the ratio
View Solution
