If V,T,L,K and r denote speed, time period, angular momentum, kinetic energy and radius of satellite in circular orbit (a)Vαr−1,(b) Lαr1/2
(c)Tαr3/2,(d) Kαr−2
a,b are true
b,c are true
a,b,d are true
a,b,c are true
The correct Answer is:B
Step by step video, text & image solution for If V, T, L, K and r denote speed, time period, angular momentum, kinetic energy and radius of satellite in circular orbit (a)Valphar^(-1),(b) Lalphar^(1//2) (c) Talphar^(3//2),(d) Kalphar^(-2) by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- GRAVITATIONBOOK - NARAYNACHAPTER - GRAVITATIONEXERCISE - LEVEL I(C.W.)
51 Videos
- GRAVITATIONBOOK - NARAYNACHAPTER - GRAVITATIONEXERCISE - LEVEL II (C.W.)
38 Videos
- GRAVITATIONBOOK - NARAYNACHAPTER - GRAVITATIONEXERCISE - EXERCISE -IV
40 Videos
- FRICTIONBOOK - NARAYNACHAPTER - FRICTIONEXERCISE - Passage type of questions I
6 Videos
- KINETIC THEORY OF GASESBOOK - NARAYNACHAPTER - KINETIC THEORY OF GASESEXERCISE - LEVEL-III(C.W)
52 Videos
Similar Questions
A planet of mass M, has two natural satellites with masses m1 and m2. The radii of their circular orbits are R1 and R2 respectively. Ignore the gravitational force between the satellites. Define v1,L1,K1 and T1 to be, respectively, the orbital speed, angular momentum, kinetic energy and time period of revolution of satellite 1 , and v2,L2,K2 and T2 to be he corresponding quantities of satellite 2. Given m1/m2=2 and R1/R2=1/4, match the ratios in List-I to the numbers in List-II.
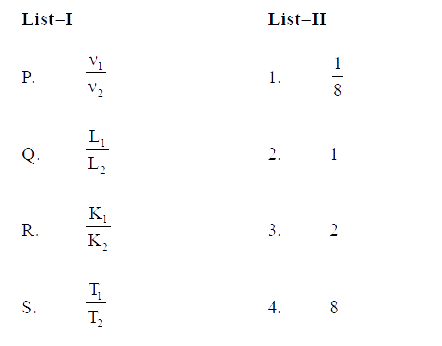
View SolutionA planet of mass , has two natural satellites with masses and . The radii of their circular orbits are and respectively. Ignore the gravitational force between the satellites. Define , and to be, respectively, the orbital speed, angular momentum, kinetic energy and time period of revolution of satellite 1, and , , and to be the corresponding quantities of satellite 2. Given/2and/1/4, match the ratios in List-I to the numbers in List-II.
View Solution
Knowledge Check
If L is the angular momentum of a satellite revolving around earth is a circular orbit of radius r with speed v , then
AL∝vBL∝rCL∝√rDL∝√vWhat are the values of the orbital angular momentum of an electron in the orbitals 1s,3s,3d and 2p:-
A0,0√6h,√2hB1,1√4h,√2hC0,1√6h,√3hD0,0√20h,√6What are the values of the orbital angular momentum of an electron in the orbitals 1s,3s,3d and 2p ?
A0,0,h2π√6,h2π√2B1,1,h2π√4,h2π√2C0,1,h2π√6,h2π√3D0,0,h2π√20,h2π√6
En= total energy , ln angular momentum
Kn=K.E.,Vn=P.E.,
Tn= time period , rn= radius of nth orbit
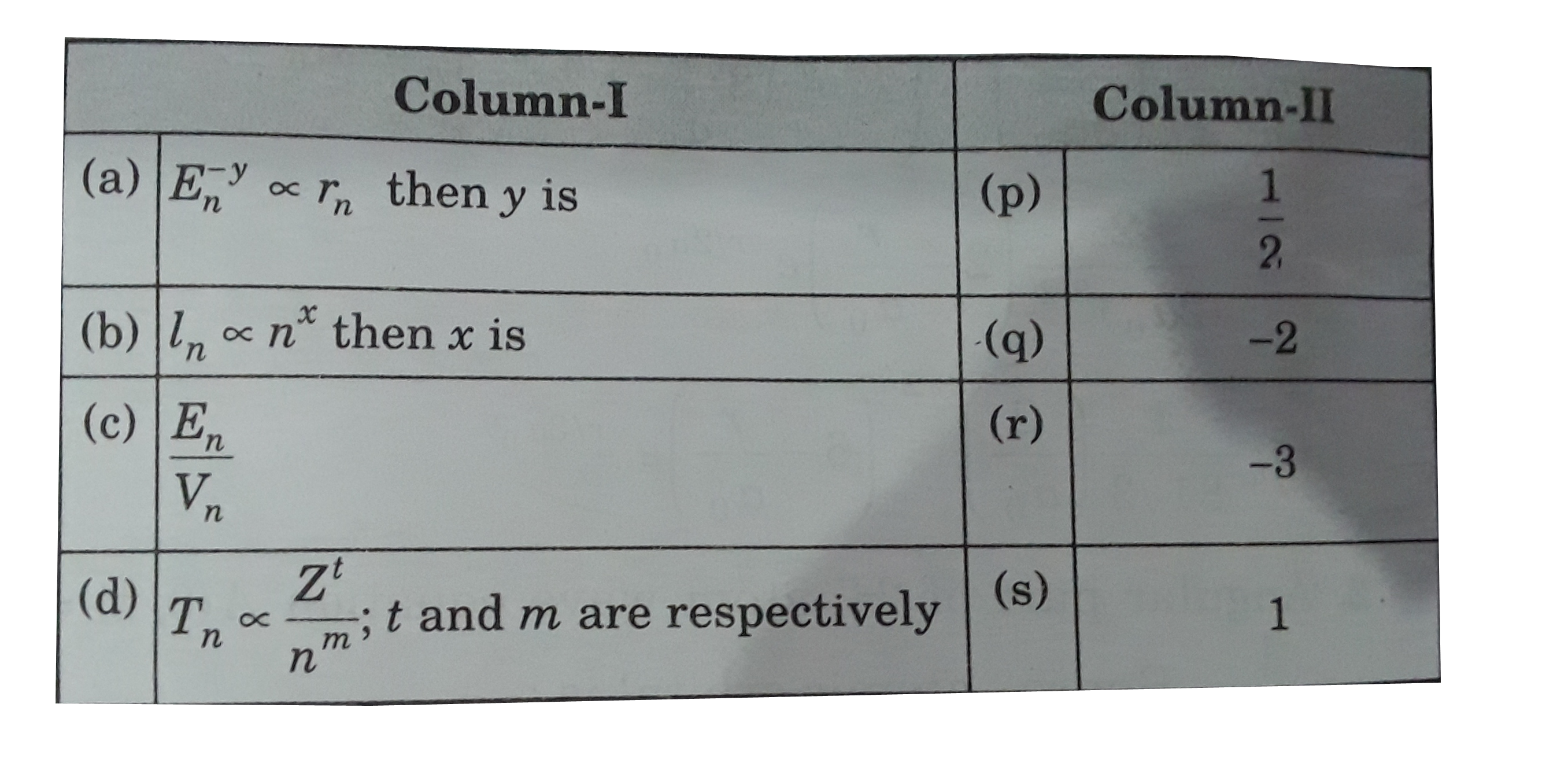
View SolutionSatellite 1 is in a certain circular orbit around a planet, while satellite 2 is in a larger circular orbit. Which satellite has (a) the longer period and (b) the greater speed ?
View SolutionA 400 kg satellite is in a circular orbite of radius 2R around the earth how much energy is required to transfer it to circular orbit of radius 4R
View SolutionIf g∝1R3 (instead of 1R2), then the relation between time period of a satellite near earth's surface and radius R will be
View SolutionThe energy required to take a satellite to a height ‘h’ above Earth surface (radius of Earth=6.4×103 km ) is E1 and kinetic energy required for the satellite to be in a circular orbit at this height is E2. The value of h for which E1 and E2 are equal, is:
View Solution
