The distance travelled by a particle in a straight line motion is directly proportional to t1/2, where t is the time elapsed.
increasing acceleration
decrease accelration
increasing retardation
decreasing reatardation
The correct Answer is:D
Given the xα√t
therefore dxdtα12√t or dxdtαt−1/2
Further , d2x)dt2α−12t−3/2
Or aα−t−3/2
∴ a is retardation and as t increase retadation decreases
Similar Questions
The distance travelled by a particle in a straight line motion is directly proportional to t^(1/2) ,where t = time elapsed.The nature of motion is
View SolutionAssertion : Distance-time graph of the motion of a body having uniformly accelerated motion is a straight line inclined to the time axis.
Reason : Distance travelled by a body having uniformly accelerated motion is directly proportional to the square of the time taken.
View Solution
Knowledge Check
The distance travelled by a particle in a straight line motion is directly poroportional to t1/2, where t is the time elapsed.
AIncreasing accelerationBDecreasing accelerationCIncreasing retardationDDecreasing retardationThe distance s travelled by a particle moving on a straight line in time t sec is given by s=2t3−9t2+12t+6 then the initial velocity of the particle is
A6B−9C12D11The displacement x of a particle in a straight line motion is given by x=1−t−t2. The correct representation of the motion is
A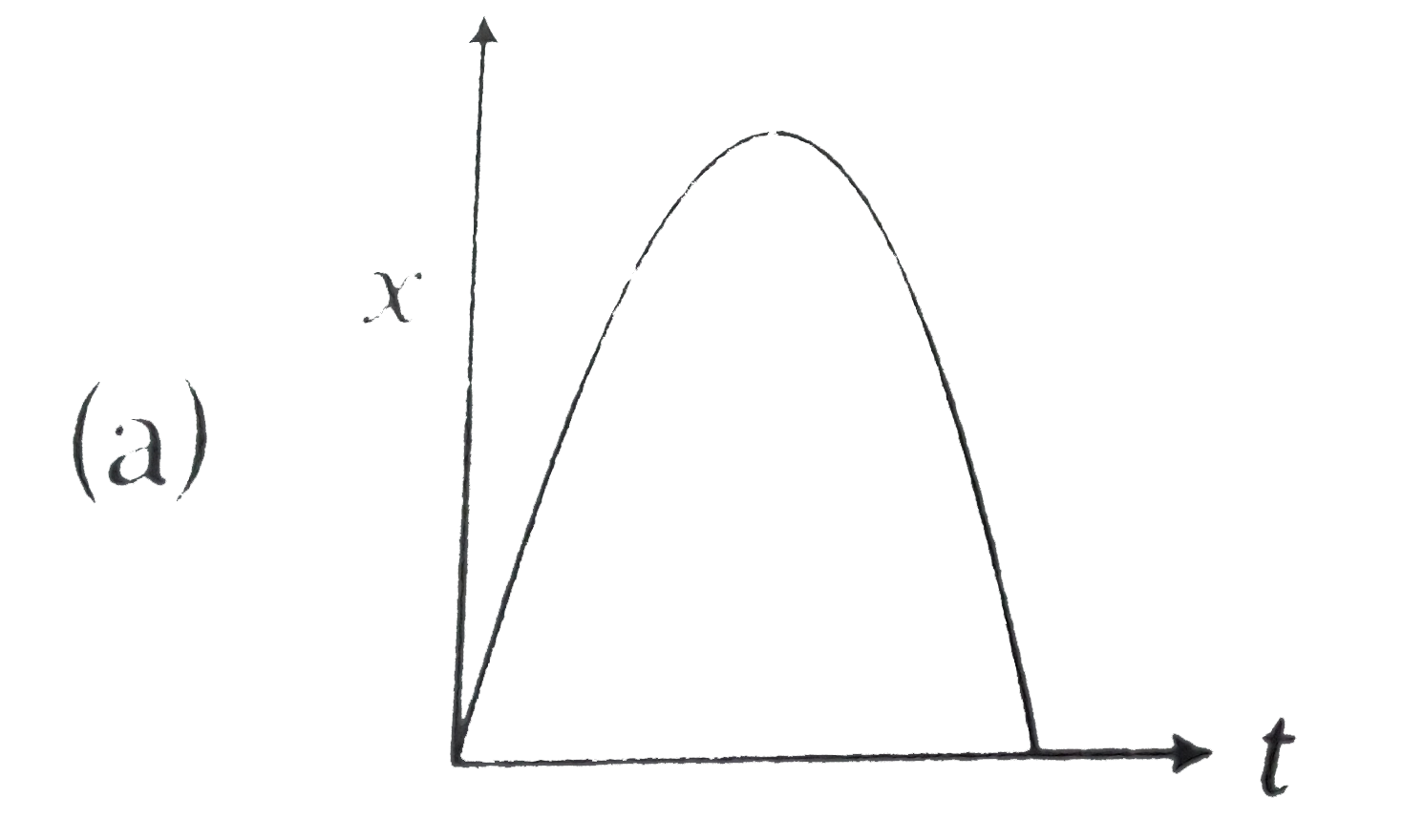 B
B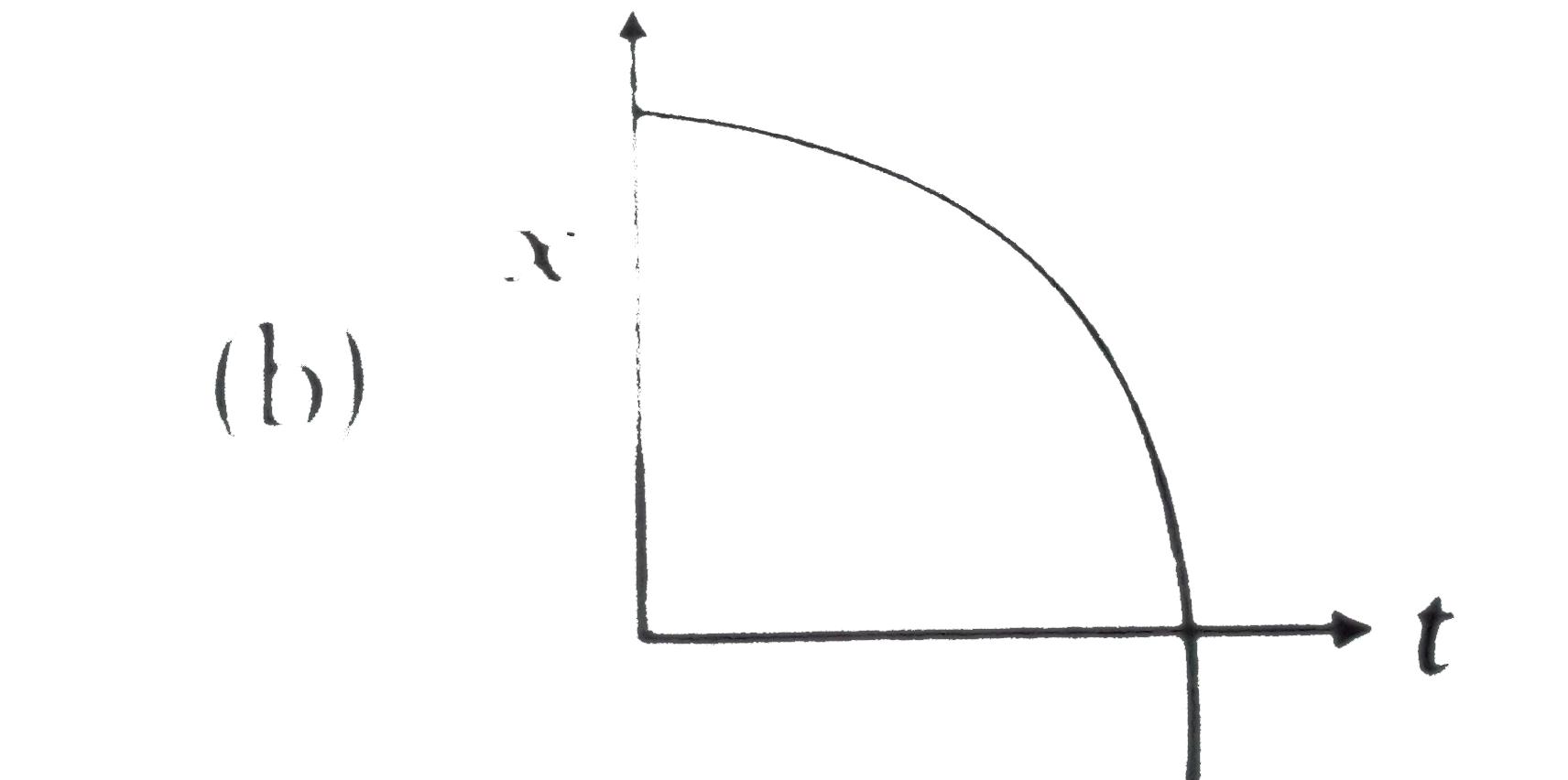 C
C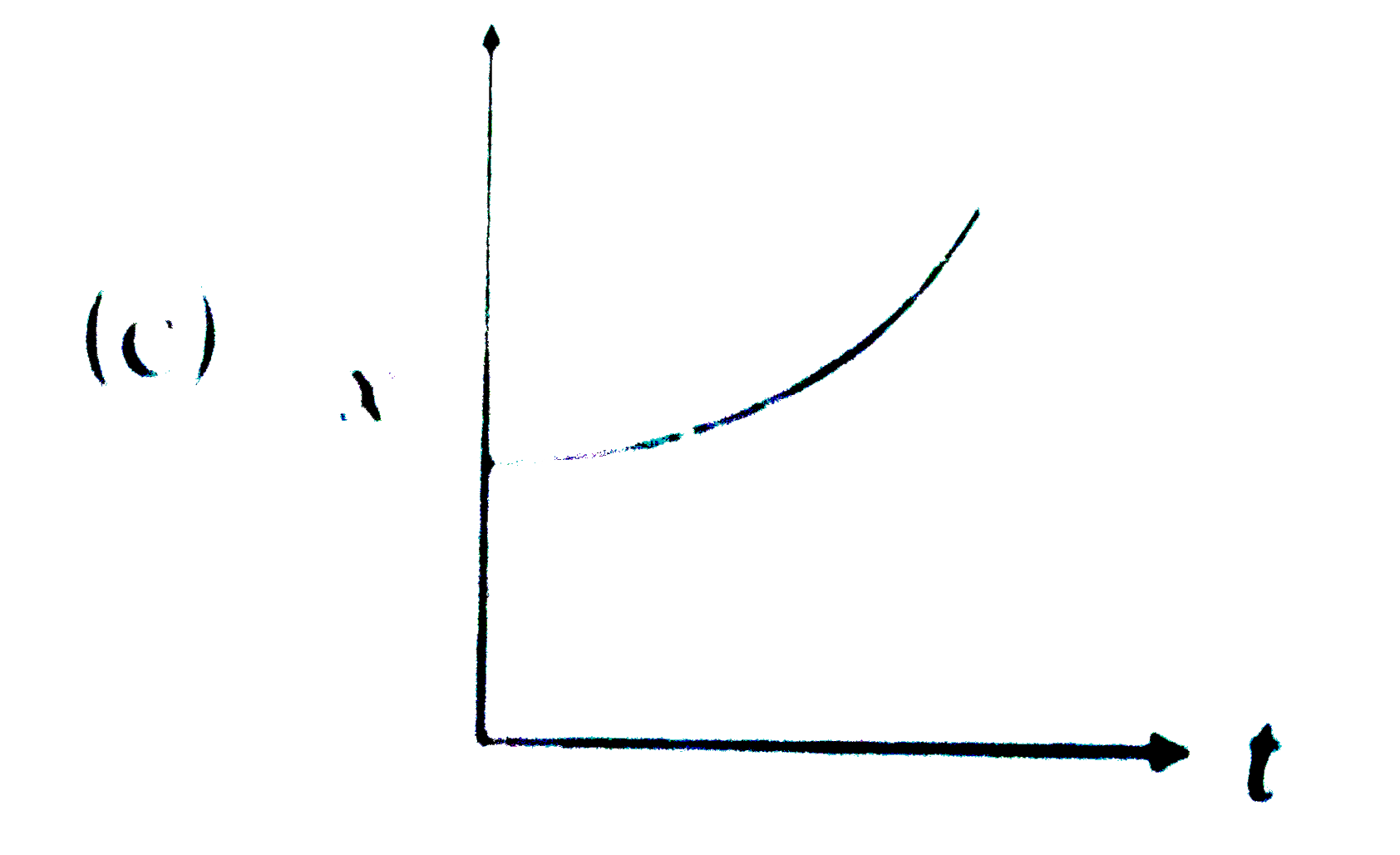 D
D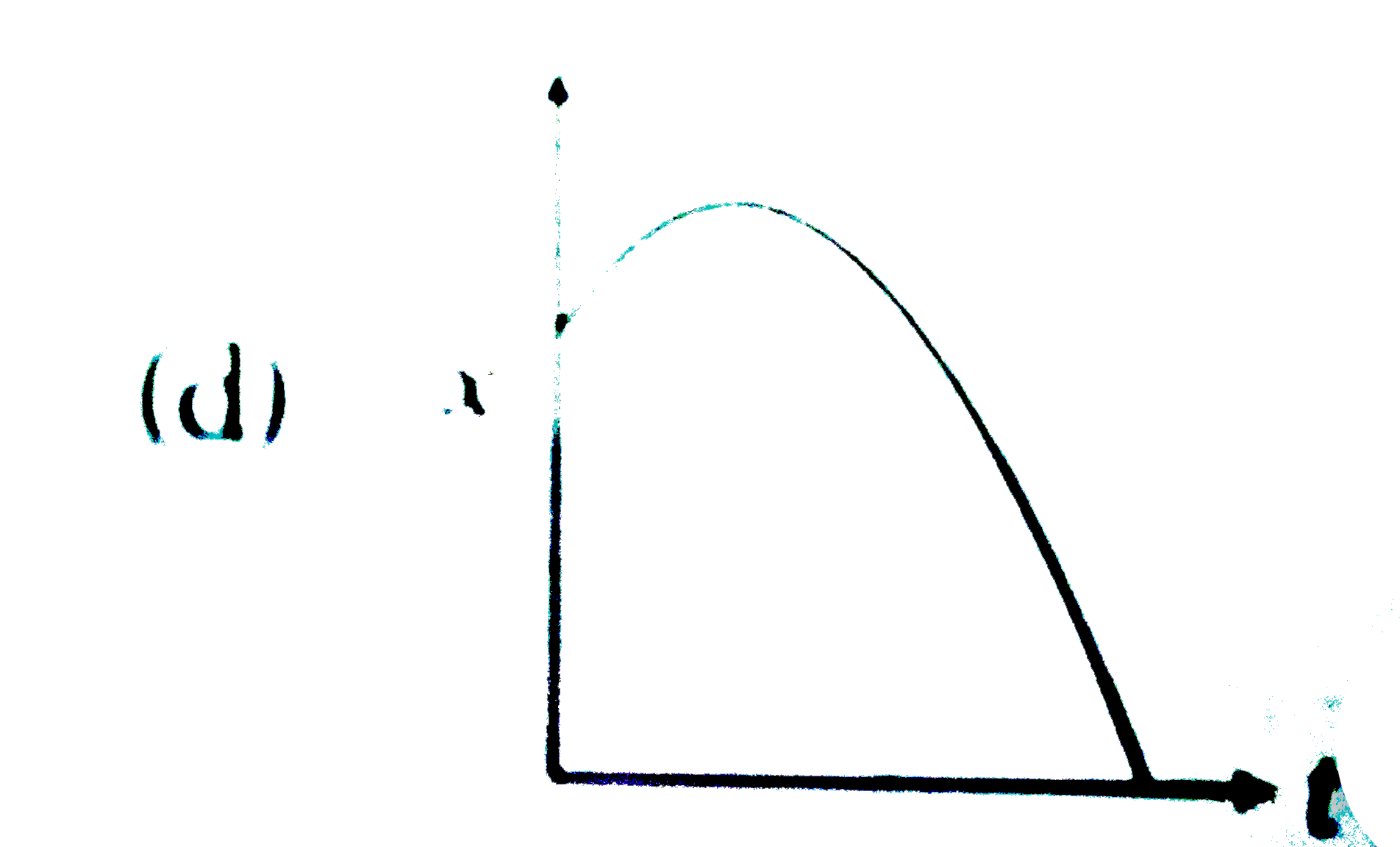
The velocity of a particle moving in a straight line is directly proportional to 3/4th power of time elapsed. How does its displacement and acceleration depend on time?
View SolutionThe position of a particle moving on a straight line is proportional to the cube of the time elapsed. How does the acceleration of the particle depend on time elapsed?
View SolutionThe distance travelled (in meters) by the particle from time t = 0 to t = t will be –
View SolutionThe distance travelled by an object along a straight line in time t is given by s=3−41+5t2 , the initial velocity of the objectis
View SolutionIf the displacement of a particle is proportional to the square of time, then-
View Solution
