The dimensionla formula for the product of two physical quantities P and Q is [ML2T−2]. The dimensional formula of PQ is [MT−2]. Then P and Q respectively are
Video Solution
Text Solution
Give P×Q has dimensions ML2T−2
P/Q has dimensions ML0T−2
Hence , (PQ) (PQ)=(ML2T−2)(ML0T−2)
P2=M2L2T−4
∴P=ML1T−2= Force ………….(1)
given PQ=M1L0T−2,ML1T−2Q=ML0T−2
Q=ML1T−2ML0T−2=L= Displacement .......... (2)
∴ P and Q are respectively the force and the displacement, hence their units are newton and metre.
|
Topper's Solved these Questions
- UNITS AND MEASUREMENTSBOOK - NARAYNACHAPTER - UNITS AND MEASUREMENTSEXERCISE - ILLUSTRATIONS
12 Videos
- UNITS AND MEASUREMENTSBOOK - NARAYNACHAPTER - UNITS AND MEASUREMENTSEXERCISE - EVALUATE YOURSELF - 1
10 Videos
- UNITS AND MEASUREMENTSBOOK - NARAYNACHAPTER - UNITS AND MEASUREMENTSEXERCISE - LEVEL-II (H.W)
20 Videos
- TRANSMISSION OF HEATBOOK - NARAYNACHAPTER - TRANSMISSION OF HEATEXERCISE - LEVEL-II(C.W)
27 Videos
- VECTORSBOOK - NARAYNACHAPTER - VECTORSEXERCISE - LEVEL-II (H.W)
14 Videos
Similar Questions
Knowledge Check
The demensional formula for the product of two physical quantites P and Q is [L2T−2] the dimensional formula of P/Q is [T2] the P and Q respectively are......
Adistance and velocityBdistance and accelerationCdisplacement and velocityDdisplacement and forceThe dimensional formula of product and quotient of two physical quantities A and B are given by [AB]=[ML2T−2],[AB]=[MT−2]. The quantities A and B respectively are
Aforce and velocityBForce and displacementCMomentum and displacementDWork and velocityIf the dimensional formula for the physical quantity is [M1L2T−2] then the physical quantity is ______ .
AtorqueBimpulseCforceDforce per unit area
Dimensional formula ML−1T2 does not represent the physical quantity
View Solution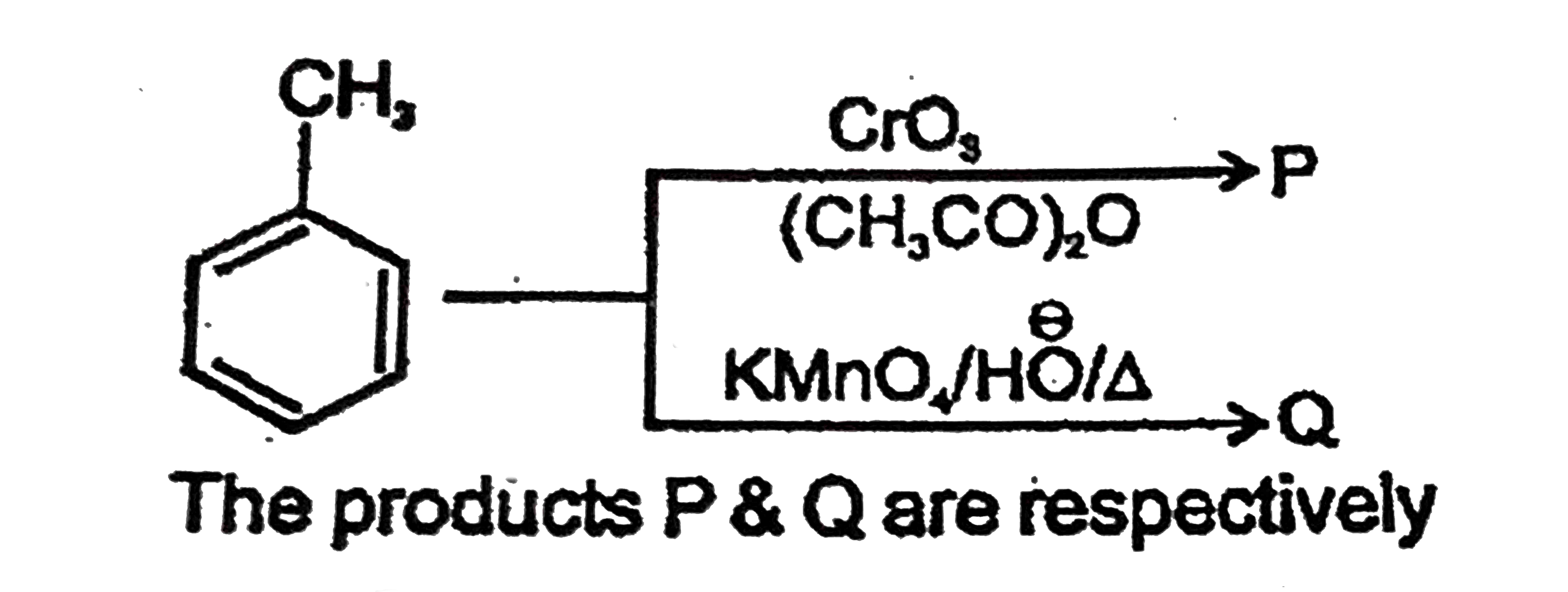
The products P & Q are respectively
View SolutionThe dimensional formula [ML−1T−2] is for the quantity
View SolutionThe SI unit of a physical quantity having the dimensional formula of [ML^(0)T^(-2) A^(-1)]
View SolutionTwo physical quantities are represented by P and Q. The dimensions of their product is [M2L−4T−4I−1] and dimensions of their ratio is [I−1]. Then P and Q respectively are
View Solution