A mark of the surface of sphere (μ=32) is viewed from a diametrically opposite position. It appears to be at a distance 15 cm from its actual position.Find the radius of sphere.
5 cm
10 cm
15 cm
25 cm
The correct Answer is:A
As the mark is viewed from the diametrically opposite position, refraction takes place at side II of the surface (the mark being on side I as shown)
here μ1=1.5,μ2=1,u=−2R
∴1v=0.5R−1.52R=−0.52R or v=-4R
Negative sign indicates that the image is formed to the left of refracting surface as shown in Figure. Further, it is given that the image of mark is at a distance 10 cm from the object. Hence: 4R = 2R + 10,∴ R = 5cm

Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRAUMENTS Book:NARAYNAChapter:RAY OPTICS AND OPTICAL INSTRAUMENTS Exercise:EXERCISE-2 (H.W)(LENSES & THEIR COMBINATIONS)
Explore 23 Videos
- RAY OPTICS AND OPTICAL INSTRAUMENTS Book:NARAYNAChapter:RAY OPTICS AND OPTICAL INSTRAUMENTS Exercise:EXERCISE-2 (H.W)(REFRACTION THROUGH A PRISM)
Explore 9 Videos
- RAY OPTICS AND OPTICAL INSTRAUMENTS Book:NARAYNAChapter:RAY OPTICS AND OPTICAL INSTRAUMENTS Exercise:EXERCISE-2 (H.W)(REFRACTION)
Explore 21 Videos
- NUCLEI Book:NARAYNAChapter:NUCLEI Exercise:ASSERTION & REASON
Explore 5 Videos
- SEMI CONDUCTOR DEVICESBook:NARAYNAChapter:SEMI CONDUCTOR DEVICESExercise:Level-II (H.W)
Explore 36 Videos
Similar Questions
A small object stuck on the surface of a glass sphere (μ=4/3) is viewed from the diametrically opposite position find transverse magnification.
View SolutionA small object stuck on the surface of a glass sphere (n=1.5) is viewed from the diametrically opposite position. Find transverse magnification.
View Solution
Knowledge Check
A sphere of radius 5 cm is cut by a plane at a distance of 4 cm from its centre. Find the radius of circular section hence formed.
A3 cmB5 cmC4 cmD6 cm
Find the surface area of sphere if radius is 9cm?
View SolutionA mark placed on the surface of glass sphere is viewed through glass from an oppositely directed position. If the diameter of the sphere is 30 cm, locate the image of the mark. For glass, μ=1.5
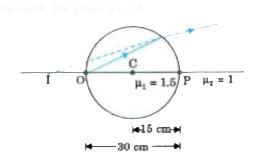
View SolutionA mark placed on the surface of a sphere is viewed through glass from a position directly opposite. If the diameter of the sphere is 10cm and refractive index of glass is 1.5, find the position of the image.
View SolutionFind the surface area of a sphere of radius 7cm.
View SolutionFind the surface area of a sphere of radius 7 cm.
View SolutionFind the surface area of a sphere of radius 14cm.
View SolutionFind the surface area of a sphere of radius 7 cm.
View Solution
