A rectangular wire of length 2.0 cm, breadth 1.5 cm is placed 25 cm in front of a concave mirror of focal length 10 cm with its centre on the axis of the mirror and its plane normal to the axis. The area enclosed by the image of the wire is
7.5cm2
6cm2
4cm2
1.33cm2
The correct Answer is:D
To solve the problem, we need to find the area enclosed by the image of a rectangular wire placed in front of a concave mirror. Let's break down the solution step by step.
Step 1: Identify the given data
- Length of the wire (object) Lo=2.0cm
- Breadth of the wire (object) Bo=1.5cm
- Distance of the object from the mirror u=−25cm (negative as per the sign convention)
- Focal length of the concave mirror f=−10cm (negative for concave mirrors)
Step 2: Use the mirror formula to find the image distance v
The mirror formula is given by:
1f=1v+1u
Rearranging gives:
1v=1f−1u
Substituting the values:
1v=1−10−1−25
Calculating the right side:
1v=−110+125=−550+250=−350
Thus,
v=−503cm≈−16.67cm
Step 3: Calculate the magnification m
The magnification m for mirrors is given by:
m=−vu
Substituting the values:
m=−(−503)÷(−25)=503×25=5075=23
Step 4: Find the dimensions of the image
Using the magnification to find the image dimensions:
- Image length Li=m⋅Lo=23⋅2.0cm=43cm≈1.33cm
- Image breadth Bi=m⋅Bo=23⋅1.5cm=1.0cm
Step 5: Calculate the area of the image
The area A of the image is given by:
A=Li⋅Bi=(43cm)⋅(1.0cm)=43cm2≈1.33cm2
Final Answer
The area enclosed by the image of the wire is approximately 43cm2 or 1.33cm2.
---
- Length of the wire (object) Lo=2.0cm
- Breadth of the wire (object) Bo=1.5cm
- Distance of the object from the mirror u=−25cm (negative as per the sign convention)
- Focal length of the concave mirror f=−10cm (negative for concave mirrors)
The mirror formula is given by:
1f=1v+1u
Rearranging gives:
1v=1f−1u
Substituting the values:
1v=1−10−1−25
Calculating the right side:
1v=−110+125=−550+250=−350
Thus,
v=−503cm≈−16.67cm
The magnification m for mirrors is given by:
m=−vu
Substituting the values:
m=−(−503)÷(−25)=503×25=5075=23
Using the magnification to find the image dimensions:
- Image length Li=m⋅Lo=23⋅2.0cm=43cm≈1.33cm
- Image breadth Bi=m⋅Bo=23⋅1.5cm=1.0cm
The area A of the image is given by:
A=Li⋅Bi=(43cm)⋅(1.0cm)=43cm2≈1.33cm2
The area enclosed by the image of the wire is approximately 43cm2 or 1.33cm2.
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRAUMENTS BOOK - NARAYNACHAPTER - RAY OPTICS AND OPTICAL INSTRAUMENTS EXERCISE - EXERCISE-2 (H.W)(REFRACTION)
21 Videos
- RAY OPTICS AND OPTICAL INSTRAUMENTS BOOK - NARAYNACHAPTER - RAY OPTICS AND OPTICAL INSTRAUMENTS EXERCISE - EXERCISE-2 (H.W)(REFRACTION THROUGH SPHERICAL SURFACES)
5 Videos
- RAY OPTICS AND OPTICAL INSTRAUMENTS BOOK - NARAYNACHAPTER - RAY OPTICS AND OPTICAL INSTRAUMENTS EXERCISE - EXERCISE-2 (C.W)( OPTICAL INSTRUMENTS (TELESCOPES) )
6 Videos
- NUCLEI BOOK - NARAYNACHAPTER - NUCLEI EXERCISE - ASSERTION & REASON
5 Videos
- SEMI CONDUCTOR DEVICESBOOK - NARAYNACHAPTER - SEMI CONDUCTOR DEVICESEXERCISE - Level-II (H.W)
36 Videos
Similar Questions
An object 4.0 cm in size, is placed 25.0 cm in front of a concave mirror of focal length 15.0 cm.
Find the size of the image.
View SolutionA rod AB of length 5 cm is placed in front of a concave mirror of focal length 10 cm as shown in figure. The length of the image of AB formed by the mirror is
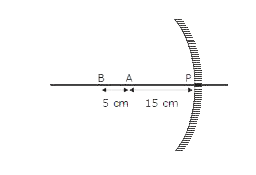
View Solution
Knowledge Check
An object is placed 10 cm in front of a convex mirror of focal length 20 cm . The distance of the image from the mirror is
A10/3 cmB20/3 cmC10 cmD40/3 cmIf an object is placed 10 cm in front of a concave mirror of focal length 20cm, the image will be
ADiminished, upright, virtualBEnlarged, upright, virtualCDiminished, inverted, realDEnlarged, upright, realAn object is placed at 50 cm in front of a concave mirror of focal length 25 cm. What is the nature of the image produced by the mirror ?
Areal and erect and of the same sizeBvirtual and inverted and bigger in sizeCreal, inverted and of the same sizeDreal, inverted and smaller in size
An object is kept 5 cm in front of a concave mirror of focal
length 15 cm. What will be the nature of the image?
View SolutionAn object is kept 5 cm in front of a concave mirror of focal length 15 cm . What will be the nature of the image
View SolutionObject is placed 15 cm from a concave mirror of focal length 10 cm, then the nature of the image formed will be
View SolutionA 2.0cm tall object is placed 15cm in front of concave mirrorr of focal length 10cm. The size and nature of the image will be
View SolutionAn object is placed 40 cm from a concave mirror of focal length 20 cm. The image formed is
View Solution
