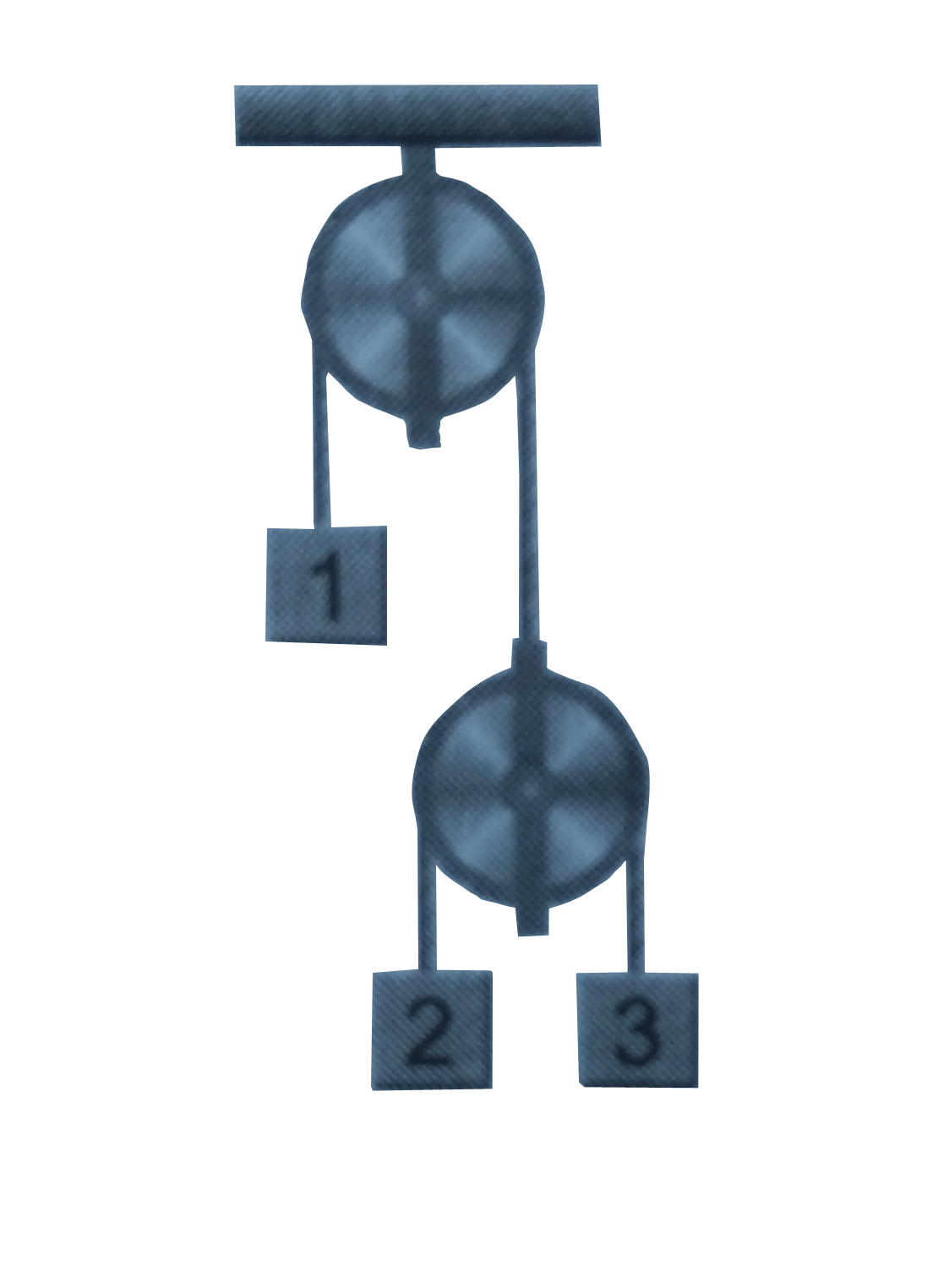
Find the relation between acceleration of blocks a1,a2 and a3.

v1+2x2+x3=l
V1+2V2+V3=0
a1+2a2+a3=0
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION & FRICTIONBOOK - ALLENCHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (S-1)
26 Videos
- NEWTON'S LAWS OF MOTION & FRICTIONBOOK - ALLENCHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (S-2)
9 Videos
- MOTION IN A PALNEBOOK - ALLENCHAPTER - MOTION IN A PALNEEXERCISE - SOLVED EXAMPLE
28 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
Similar Questions
Knowledge Check
Find the relation between acceleration a1 and a2
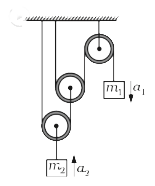 Aa1=2a2Ba1=4a2Ca1=a2Da1=3a2
Aa1=2a2Ba1=4a2Ca1=a2Da1=3a2Three blocks A, B, and C of masses 3M,2M, and M are suspended vertically with the help of spring PQ and TU, and a string RS as shown in fig. If the acceleration of blocks A, B and C is a1,a2 and a3 , respectively, then
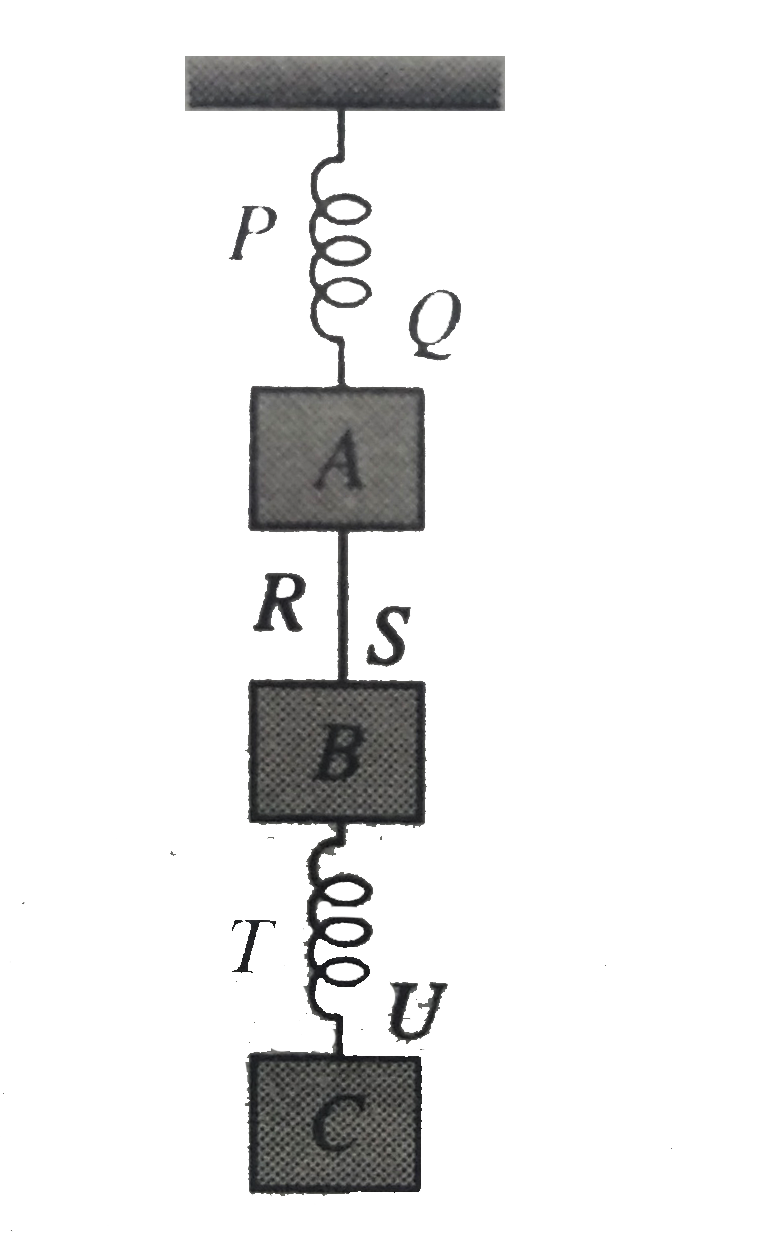
The value of acceleration a1 at the moment string RS is cut isAg, downwardBg, upwardCMore than g, downwardsDZeroThree blocks A, B, and C of masses 3M,2M, and M are suspended vertically with the help of spring PQ and TU, and a string RS as shown in fig. If the acceleration of blocks A, B and C is a1,a2 and a3 , respectively, then
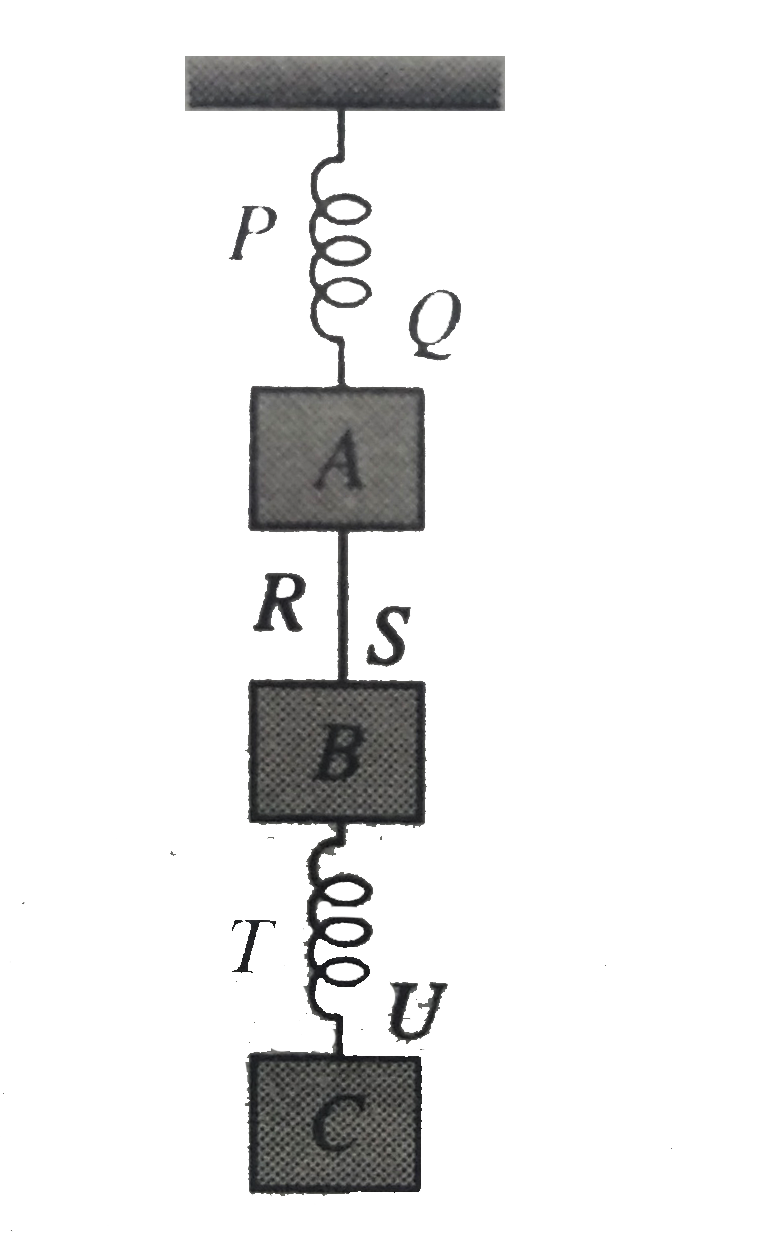
The value of acceleration a2 at the moment spring TU is cut isAg/5, upwardBg/5 downwardCg/3, upwardDZero
A planet moving around sun sweeps area A1 in 2 days, A2 in 3 days and A3 in 6 days, then find the relation between A1,A2andA3
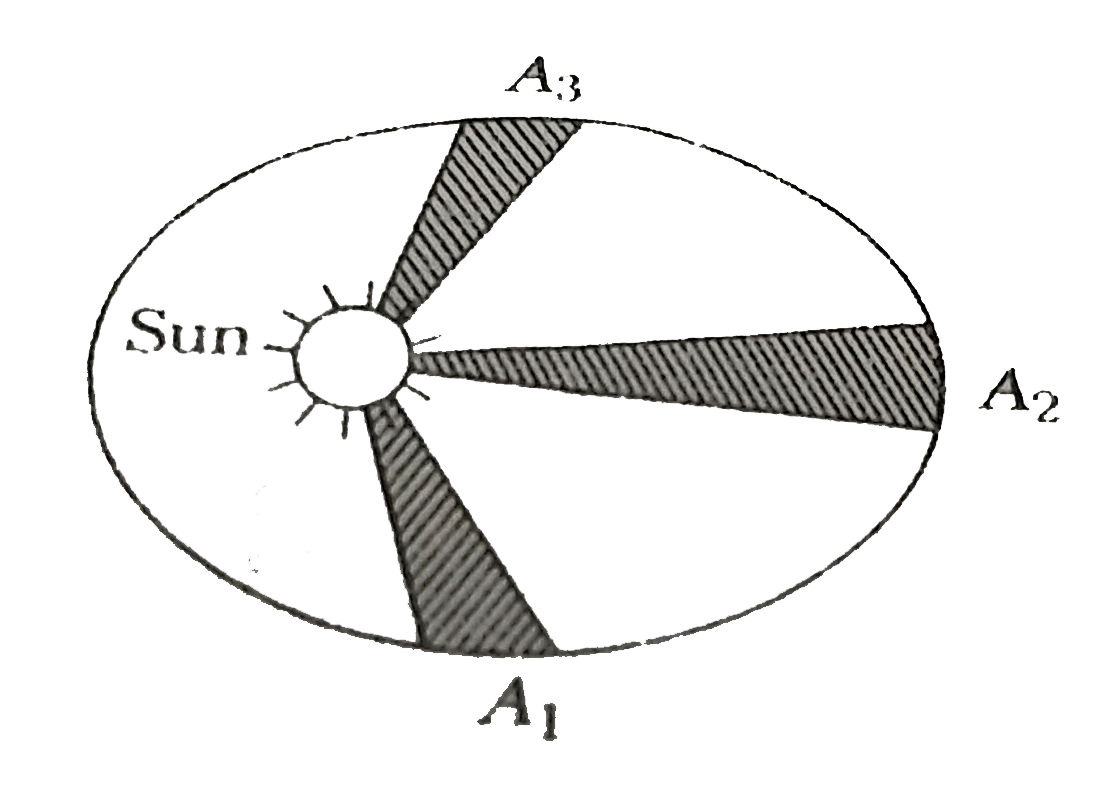
View SolutionThree blocks A, B, and C of masses 3M,2M, and M are suspended vertically with the help of spring PQ and TU, and a string RS as shown in fig. If the acceleration of blocks A, B and C is a1,a2 and a3 , respectively, then
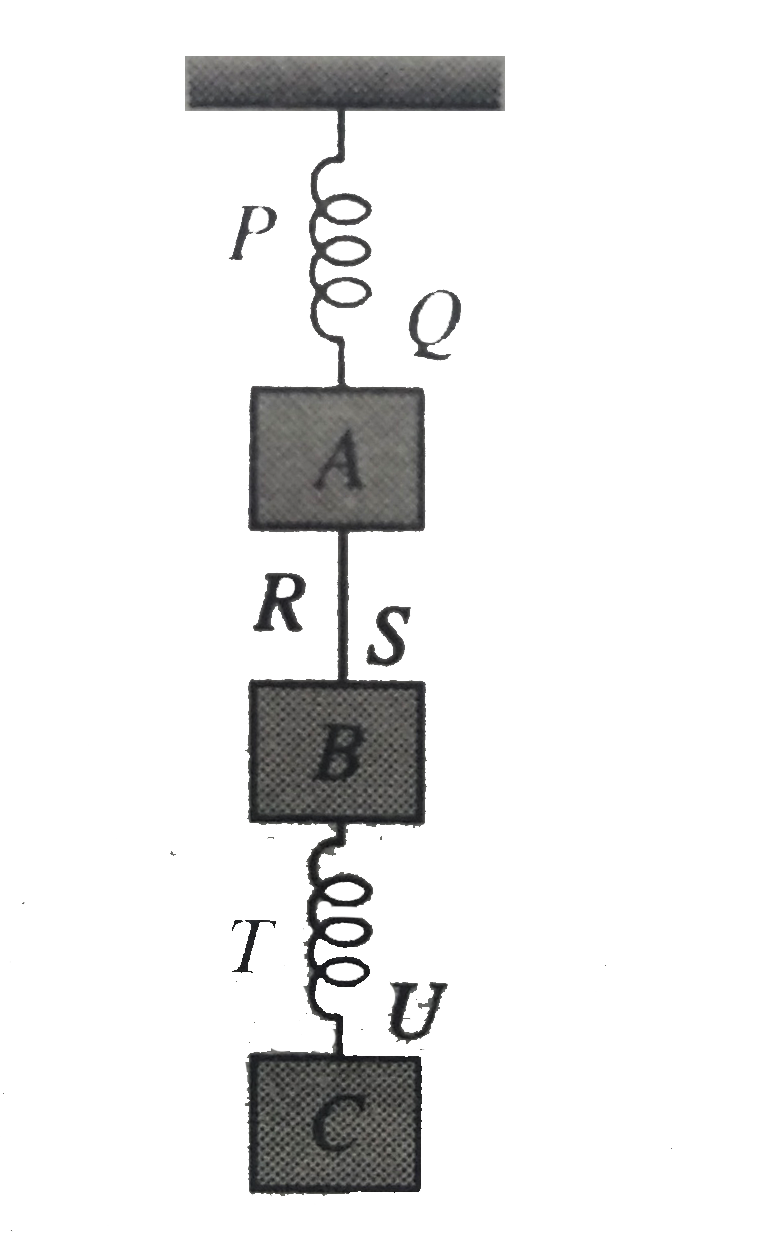
The value of acceleration a3 at the moment spring PQ is cut is
View SolutionIf the blocks are moving as shown in the figure the relation between a1 , a2 and a3 will be.
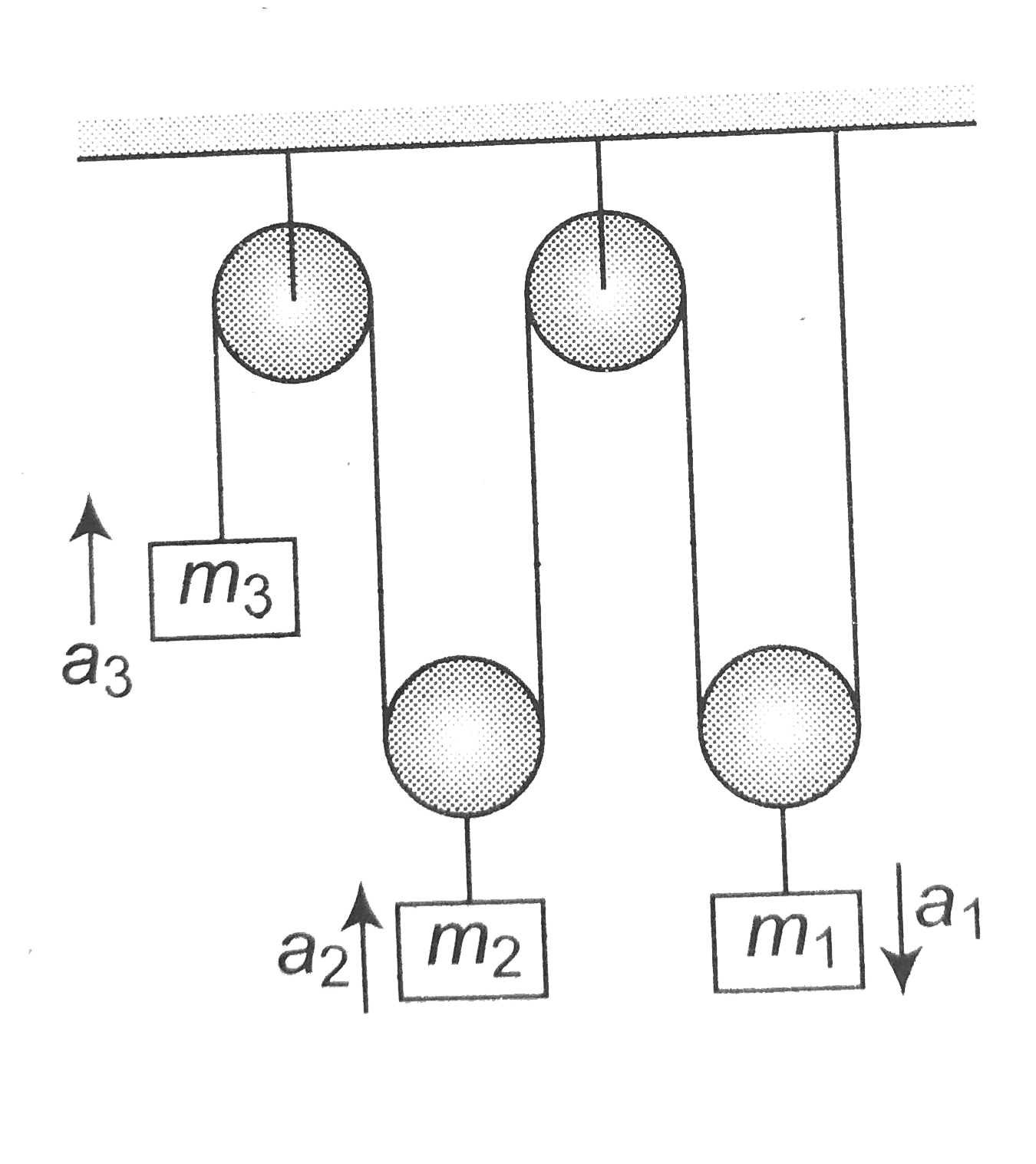
View SolutionA pulley-block arrangement is shown in the adjacent figure, if acceleration of m1,m2 and m3 are a1,a2 and a3 respectively, then relation between a1,a2 and a3 is ( All string and pulleys are ideal . )
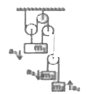
View SolutionIn the adjacent figure, all the pulleys are frictioniess and massless, all the stringes are also massless. The relation between a1,a2 and a3 is (where a1,a2 and a3 are the acceleration of masses m1,m2 and m3 respectively in the direction as shown in the figure)
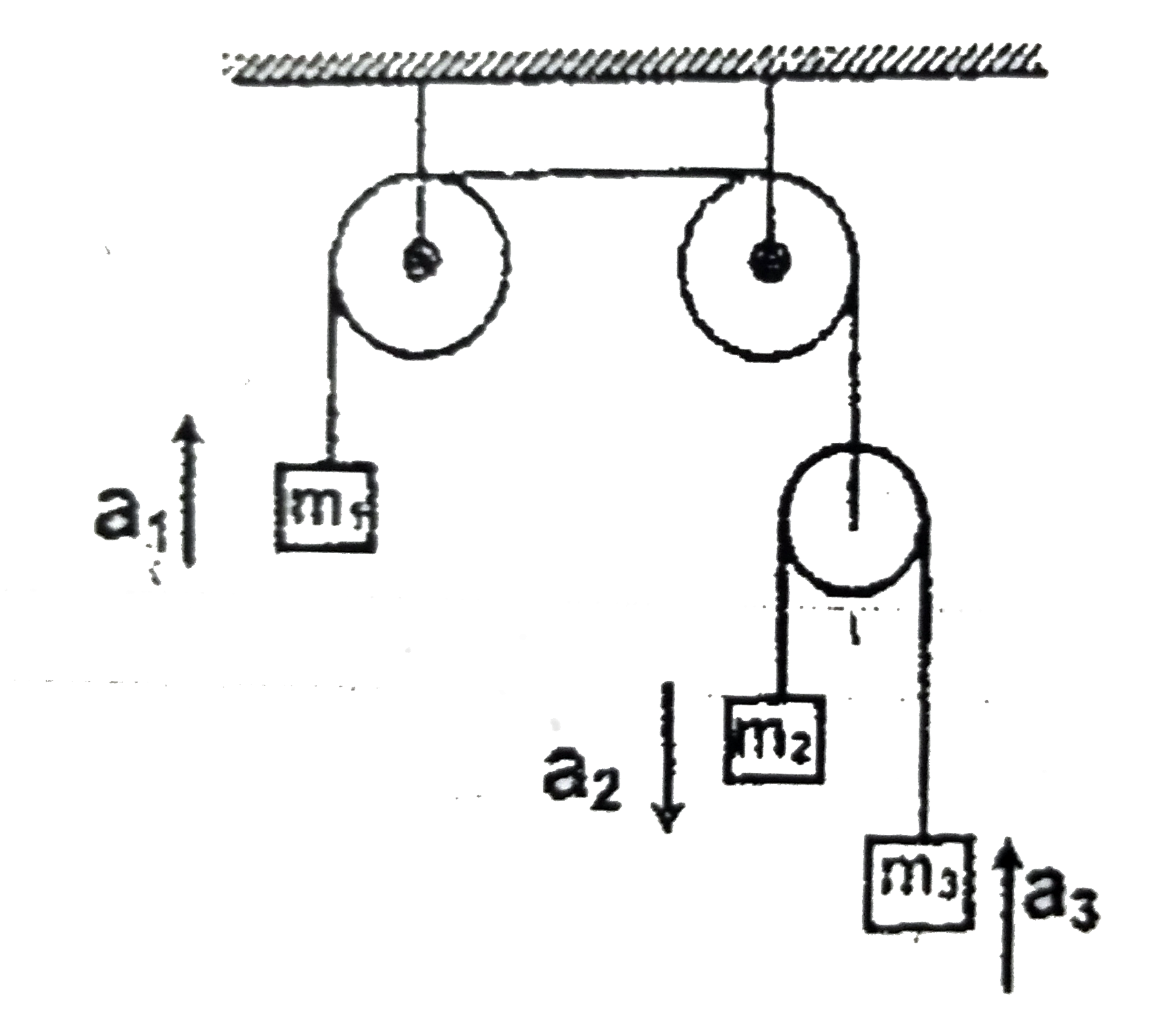
View Solution

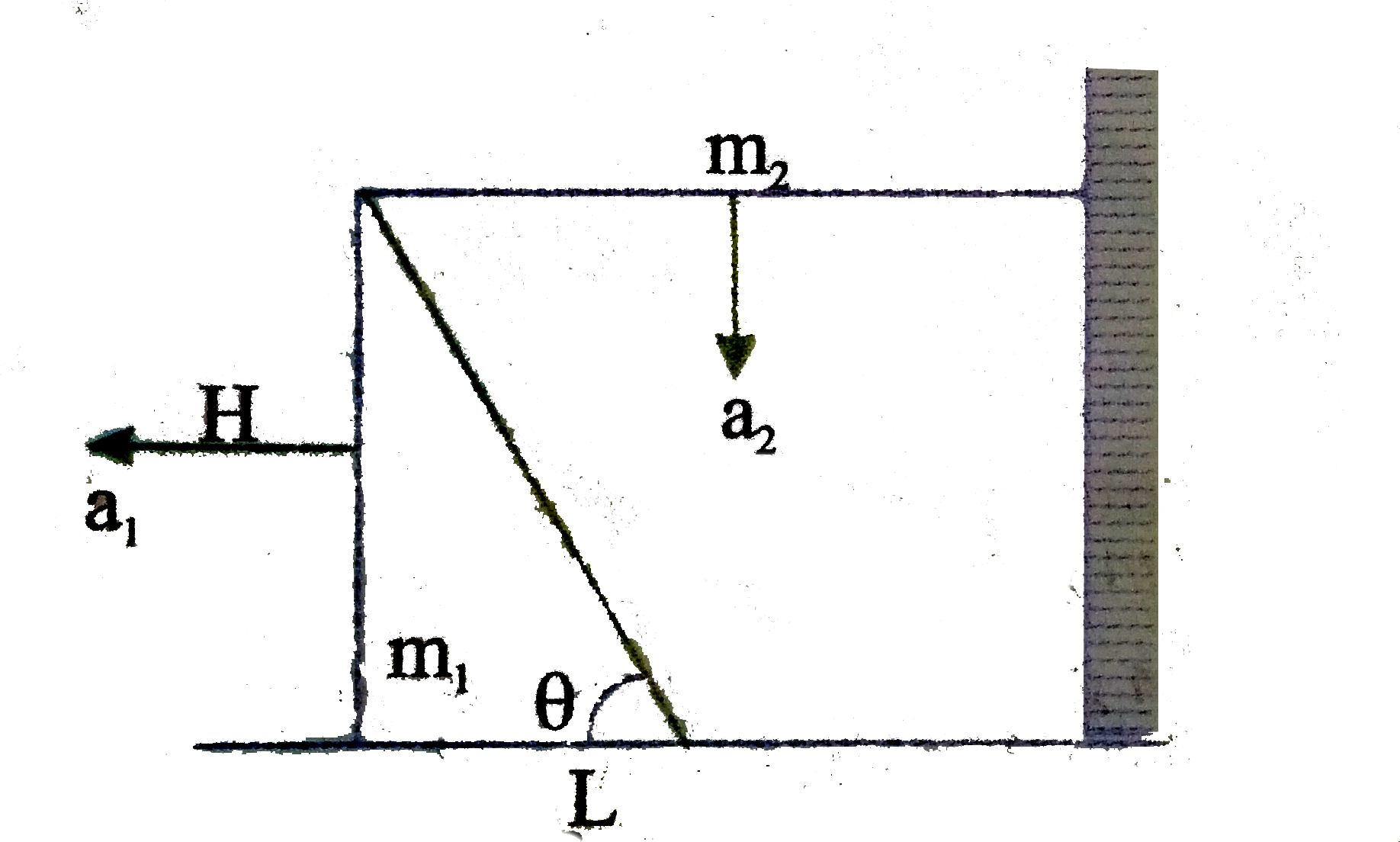 .
.