In the shown figure inside a fixed hollow cylinder with vetical axis a pendulumm is rotating in a conical path with its axis same as that of the cylinder with uniform angular velocity. Radius of culinder is 30cm, length of string is 50cm and mass of bob is 400gm. The bob makes contact with the inner fricitonless wall of the cylinder while moving
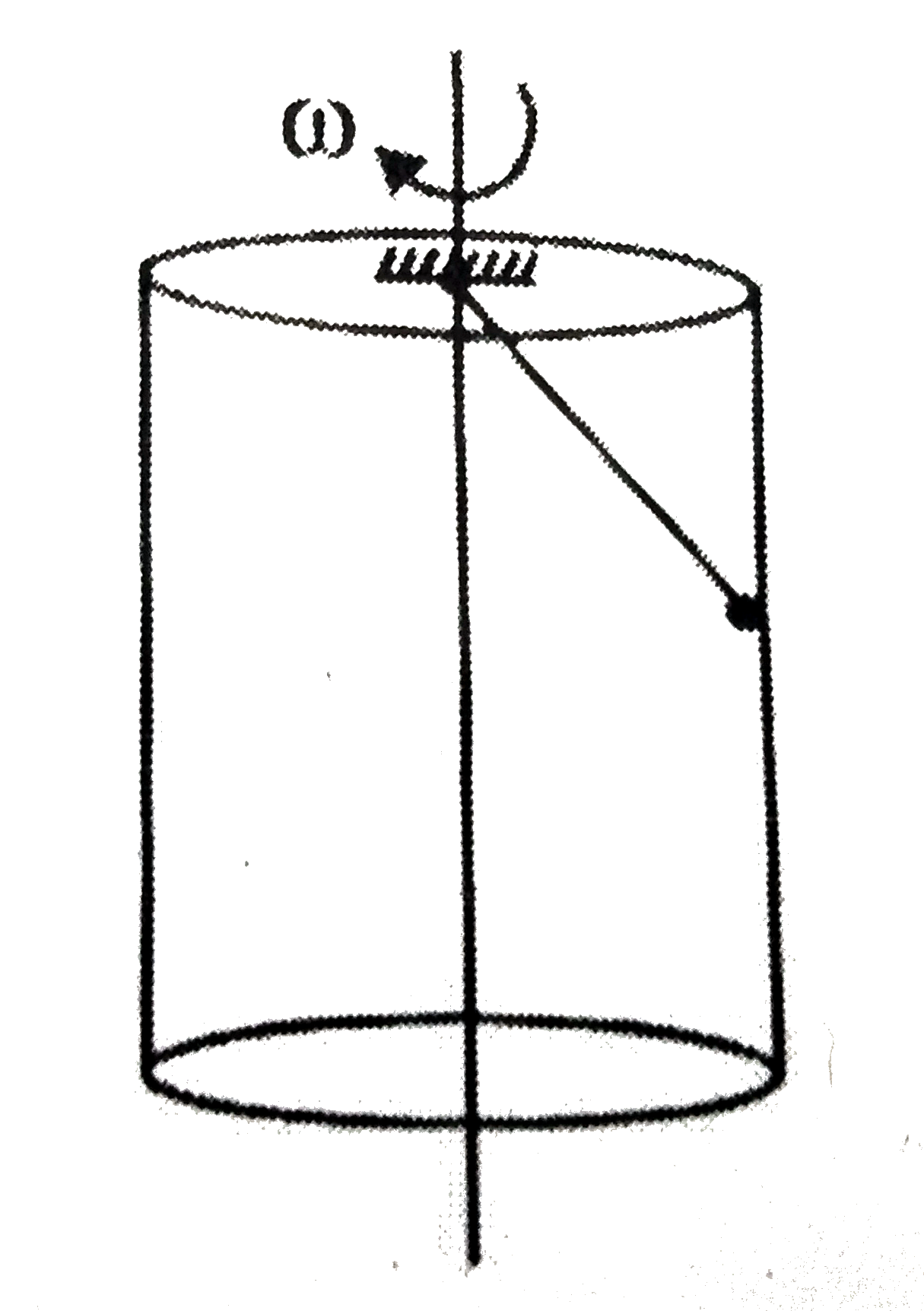
The minimum value of angular velocity of the bob so that it does not leave contact is s 5 rad/s
Tension in the string is 5N for all values of angular velocity
For angular velocity of 10 rad/s the bob pushes the cylinder with a force of 9N
For angular velocity of 10 rad/s, tension in the string is 20N
The correct Answer is:A, B, C
Step by step video, text & image solution for In the shown figure inside a fixed hollow cylinder with vetical axis a pendulumm is rotating in a conical path with its axis same as that of the cylinder with uniform angular velocity. Radius of culinder is 30cm, length of string is 50cm and mass of bob is 400gm. The bob makes contact with the inner fricitonless wall of the cylinder while moving by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - ALLENCHAPTER - CIRCULAR MOTIONEXERCISE - EXERCISE (J-M)
5 Videos
- CIRCULAR MOTIONBOOK - ALLENCHAPTER - CIRCULAR MOTIONEXERCISE - EXERCISE (J-A)
6 Videos
- CIRCULAR MOTIONBOOK - ALLENCHAPTER - CIRCULAR MOTIONEXERCISE - EXERCISE(O-2)
7 Videos
- BASIC MATHSBOOK - ALLENCHAPTER - BASIC MATHSEXERCISE - Question
1 Videos
- CURRENT ELECTRICITYBOOK - ALLENCHAPTER - CURRENT ELECTRICITYEXERCISE - All Questions
427 Videos
Similar Questions
Let the moment of inertia of a hollow cylinder of length 30 cm (inner radius 10 cm and outer radius 20 cm ), about its axis be I. the radius of a thin cylinder of the same about its axis is also I is :
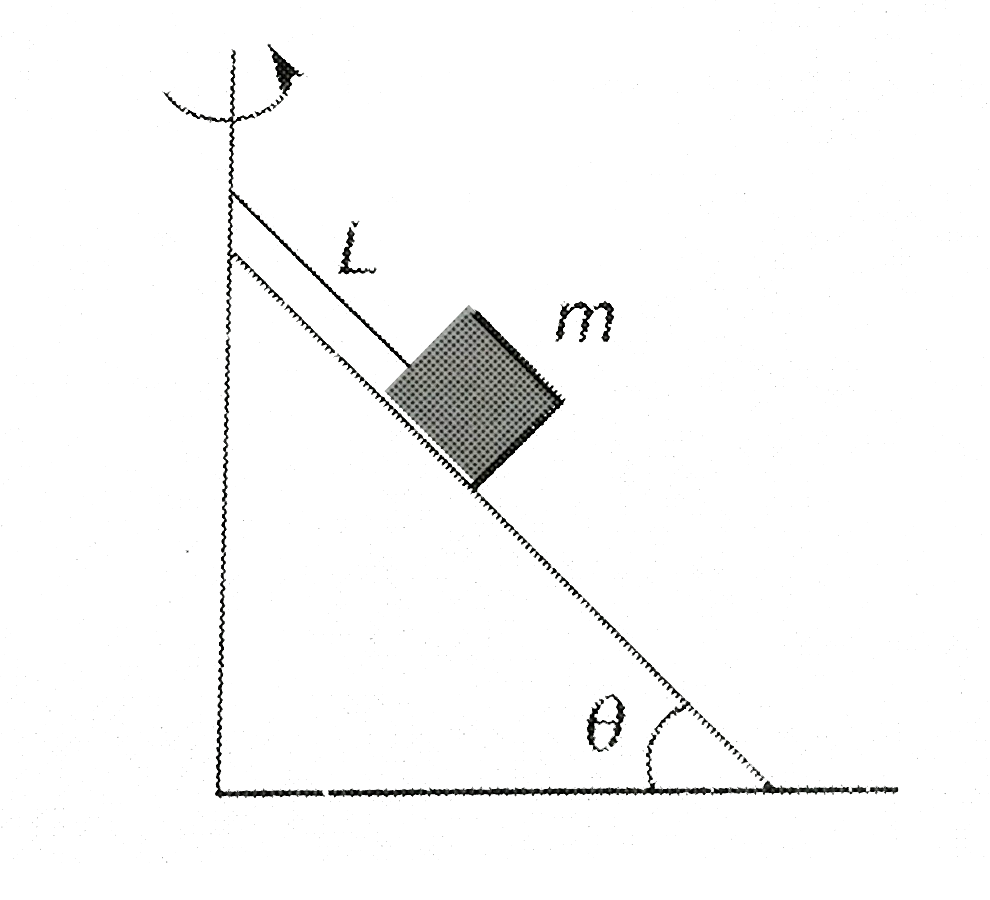
View SolutionA small block of mass m is tied to the top of a smooth inclined plane with the help of a string of length L as shown in the figure. The inclined plane of inclination θ with horizontal is rotated with an angular velocity ω about a verticle axis passing through the end of the string fixed to the plane.
(a) Find the maximum value of ω so that the block maintains contact with the inclined plane.
(b) Find the ratio of the tension in the string and the normal reaction between the block and the plane.
View Solution
Knowledge Check
A solid cylinder of mass 2 kg and radius 0.2m is rotating about its own axis without friction with angular velocity 3 rads/s. A particle of mass 0.5 kg and moving with a velocity 5 m/s strikes the cylinder and sticks to it as shown in figure. The angular momentum of the cylinder before collision will be?
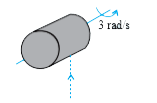 A0.12J-sB12J-sC1.2J-sD1.12J-s
A0.12J-sB12J-sC1.2J-sD1.12J-sThe axis of the uniform cylinder in figure is fixed. The cylinder is initially at rest. The block of mass M is initially moving to the friction and with speed v1. It passes over the cylinder to the dashed position. When it first makes contact with the cylinder, it slips on the cylinder, but the friciton is large enough so that slipping ceases before M loses contacts wtih the cylinder. the cylinder has a radius R and a rotaitonal intertia I
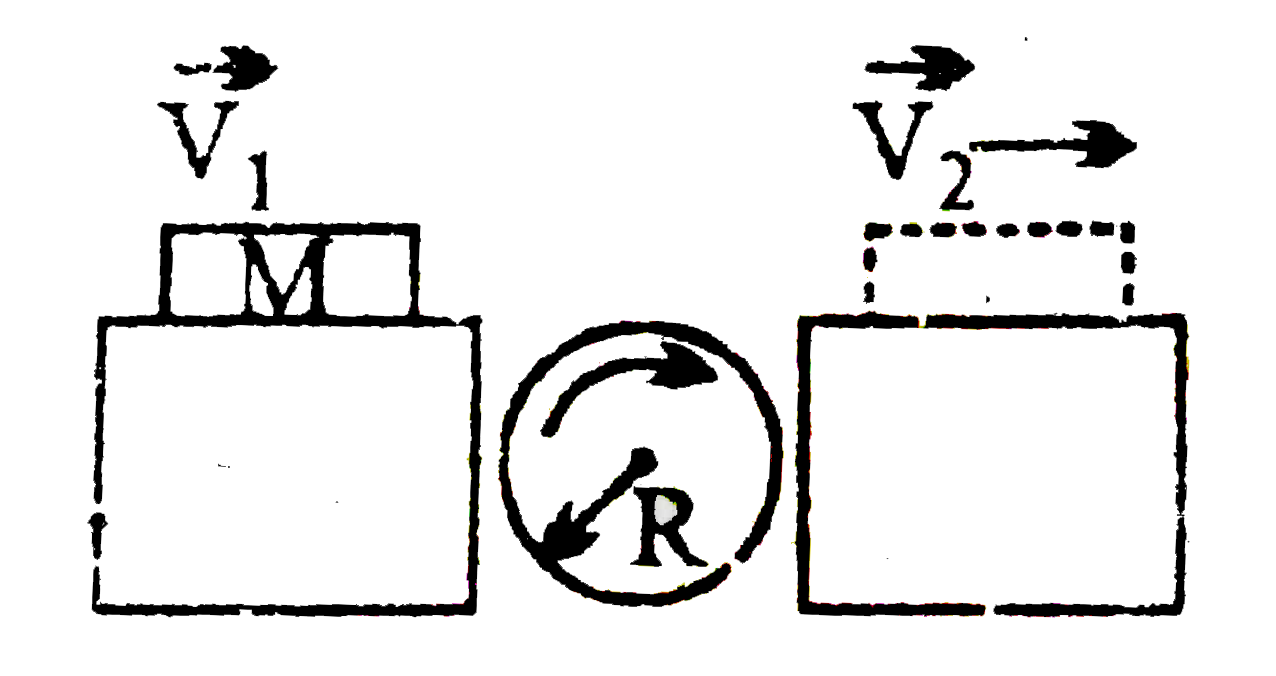
If ω is final angular velocity of the cylinder, thenAv1=ωRBv2=ωRCv1<ωRDnoneThe axis of the uniform cylinder in figure is fixed. The cylinder is initially at rest. The block of mass M is initially moving to the friction and with speed v1. It passes over the cylinder to the dashed position. When it first makes contact with the cylinder, it slips on the cylinder, but the friciton is large enough so that slipping ceases before M loses contacts wtih the cylinder. the cylinder has a radius R and a rotaitonal intertia I
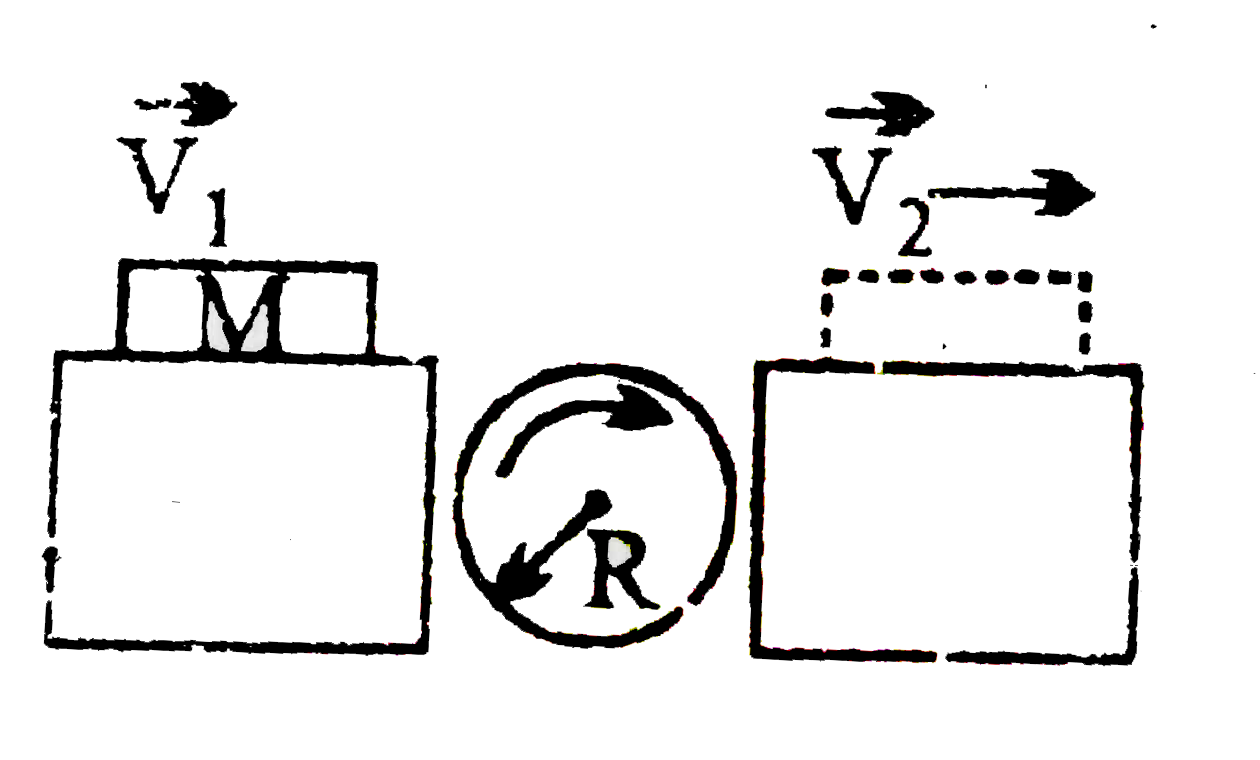
For the entire process the quantitiy (ies) which will remain conserved for the (cylinder+block) system is/ are (anuglar momentum is considered about the cylinder axis)Amechanical energy, momentum and angular momentumBmechanical energy & angular momentum onlyCmomentum & angular momentum onlyDangular momentum only
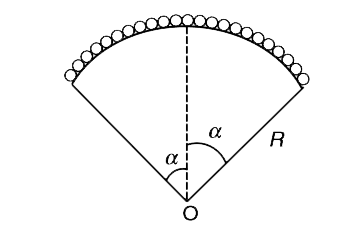
(a) A uniform chain is lying in form of on arc of a circle of radius R. The arc subtends an angle of 2α at the centre of the circle. Find the distance of the centre of mass of the chain from the centre of the circle.
(b) A uniform chain of length πR2 is lying symmetrically on the top of a fixed smooth half cylinder (see figure) of radius R. The chain is pulled slightly from one side and released. It begins to slide. Find the speed of the chain when its one end just touches the floor. What is speed of centre of mass of the chain at this instant ?
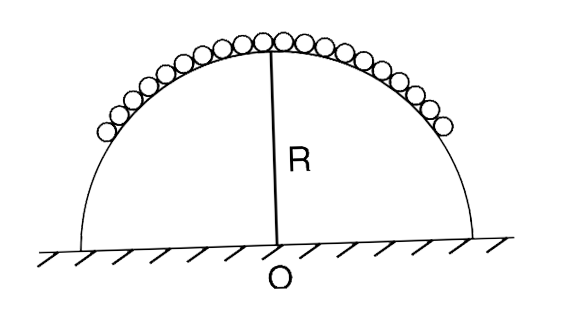
(c) In part (b) assume that the half cylinder is not fixed and can slide on the smooth floor. Find the displacement of the cylinder by the time one end of the chain touches the floor. Mass of cylinder is equal to that of the chain. For part (b) and (c) assume that the chain remains in contact with the cylinder all the while.
View SolutionA uniform cylinder of radius 12 cm and mass 25 kg is mounted so as to rotate freely about a horizontal axis that is parallel to and 5.0 cm from the central longitudinal axis of the cylinder. (a) What is the rotational inertia of the cylinder about the axis of rotation? (b) If the cyliner is released from rest with its central longitudinal axis at the same height as the axis about which the cylinder rotates, what is the angular speed of the cylinder as it passes through its lowest position?
View SolutionThere is a hollow cylinder of radius R and it is rotating with constant angular speed ω. There is a point mass which rotates along with the cylinder and geta carried upward. Friction is enough such that the point mass does not slip with respect to the cylinder as long as the normal force is becomes zero. If the path of the particle after it lost contact with the cylinder is as shown in the figure then,

In Order to happen this where most the particle lose contact with the cylinder?
View SolutionThe axis of the uniform cylinder in figure is fixed. The cylinder is initially at rest. The block of mass M is initially moving to the friction and with speed v1. It passes over the cylinder to the dashed position. When it first makes contact with the cylinder, it slips on the cylinder, but the friciton is large enough so that slipping ceases before M loses contacts wtih the cylinder. the cylinder has a radius R and a rotaitonal intertia I
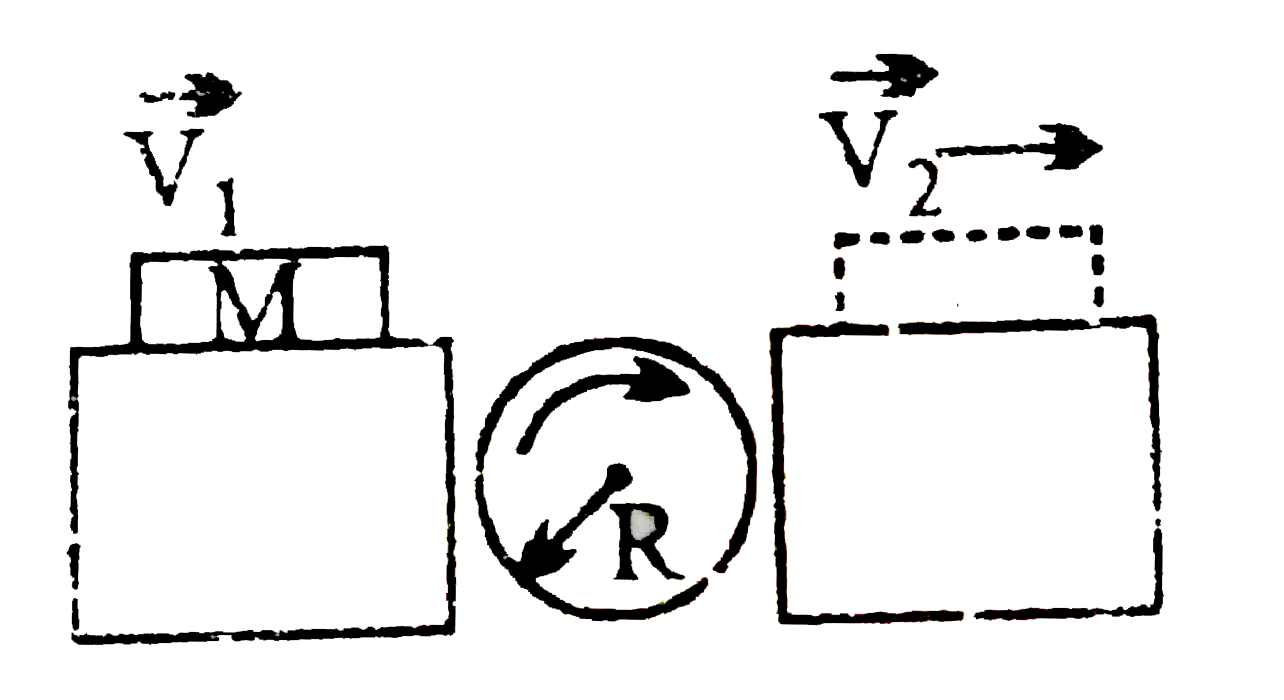
Assertion : Momentum of the block -cylinder system is conserved
Reason: Force of friction between block and cylinder is internal force of block -cylinder system.
View SolutionThere is a hollow cylinder of radius R and it is rotating with constant angular speed ω. There is a point mass which rotates along with the cylinder and geta carried upward. Friction is enough such that the point mass does not slip with respect to the cylinder as long as the normal force is becomes zero. If the path of the particle after it lost contact with the cylinder is as shown in the figure then,

What is the value of ω (in terms of g and R)
View Solution
