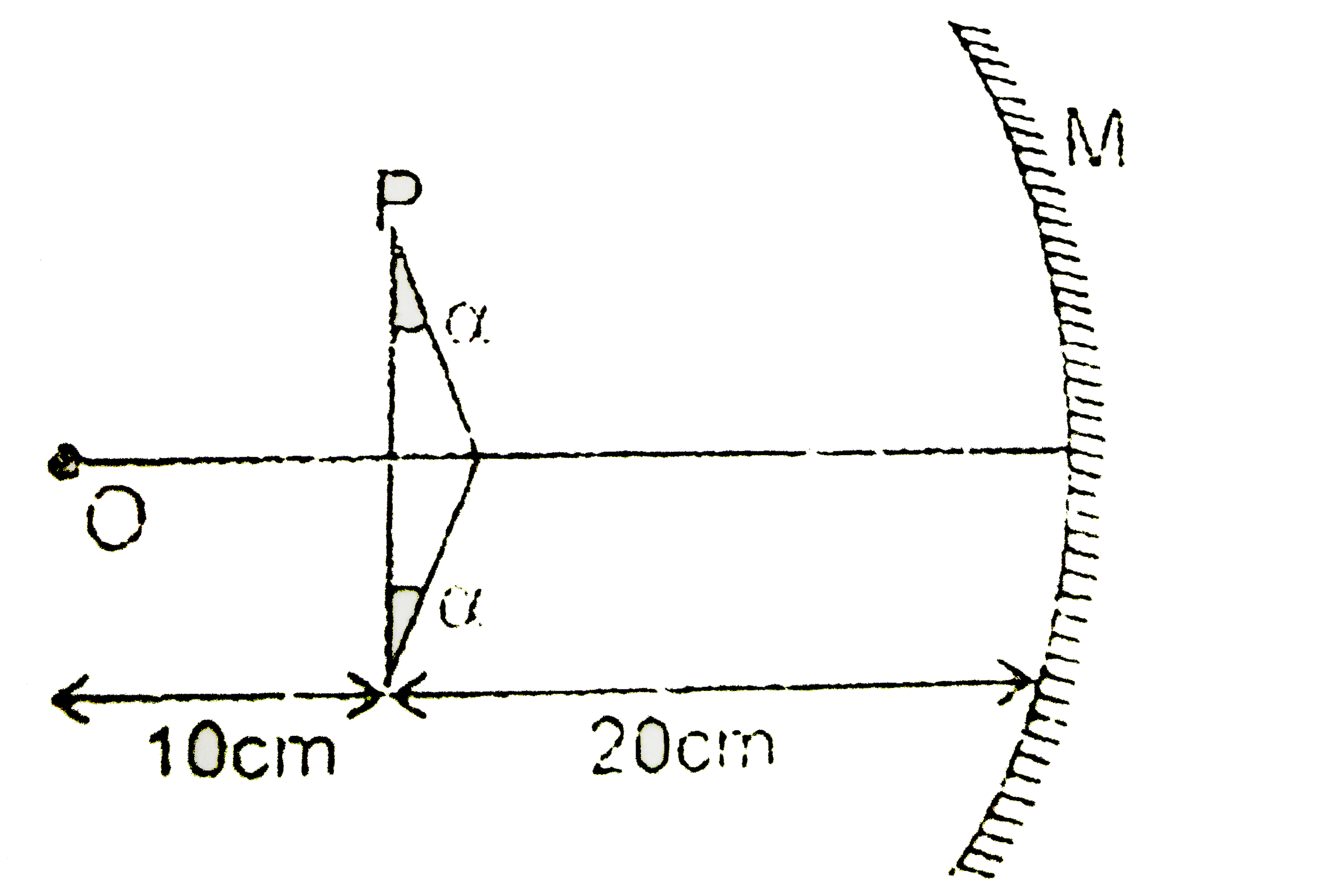
In the given figure, is a point object kept on the principal axis of a concave mirror of radius of curvature . is a prism of angle . Light falling on the prism (at small angle of incidence) get refracted through the prism and then fall on the mirror. Rrfractive index of prism is . Find the distance between the images formed by the concave mirror due to this light.
The correct Answer is:.
For thin prism
Distance
similarly distance
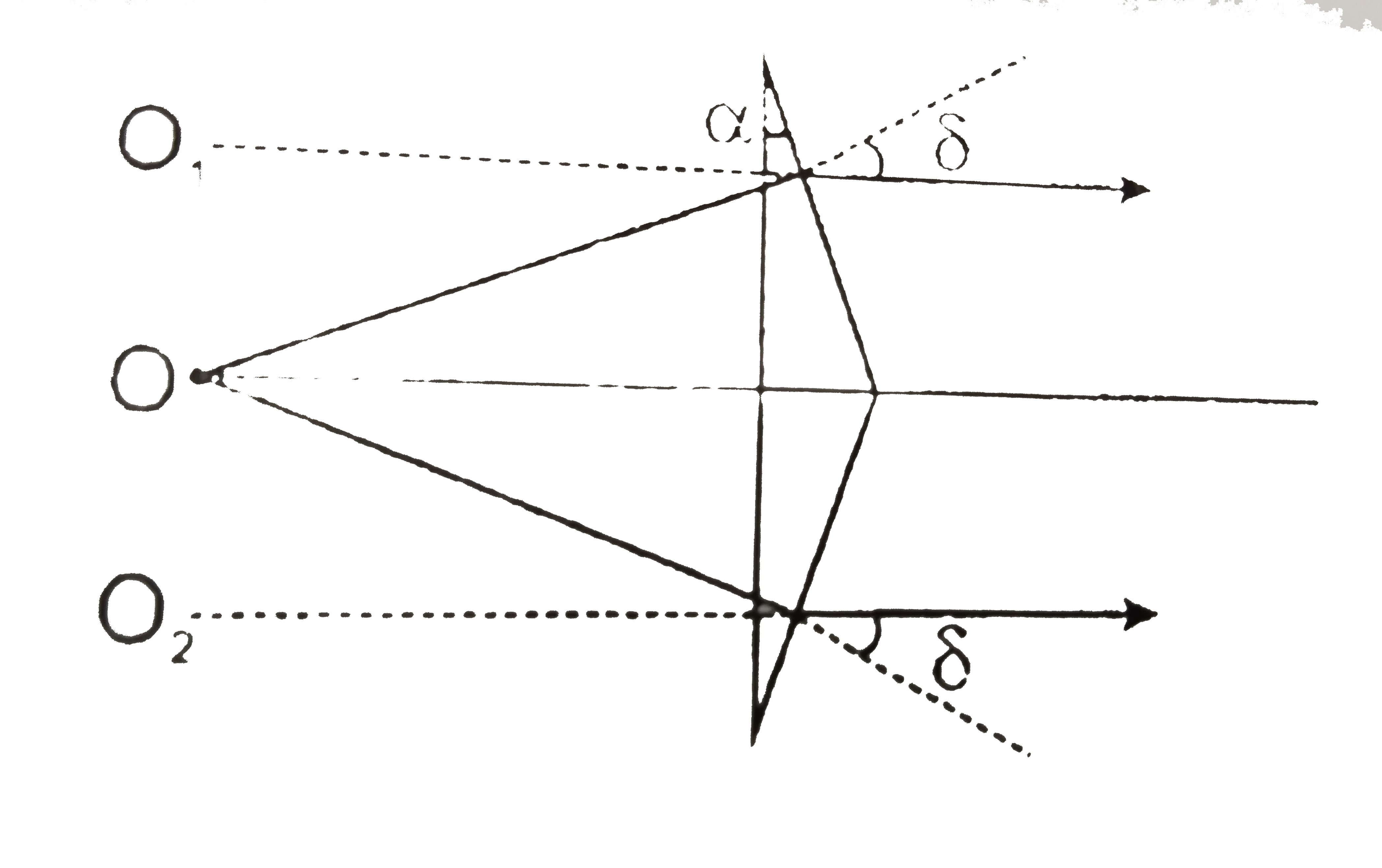
For mirror are two point objects, at a distance of .
Now applying mirror formula
Lateral magnification
(-ve sign indicates inverted image)
O_(1)O_(2)O_(1)'O'_(2) =(1)/(2)O_(1)O_(2)=(1)/(2).(pi)/(10)=(pi)/(20)cm.
Topper's Solved these Questions
Similar Questions
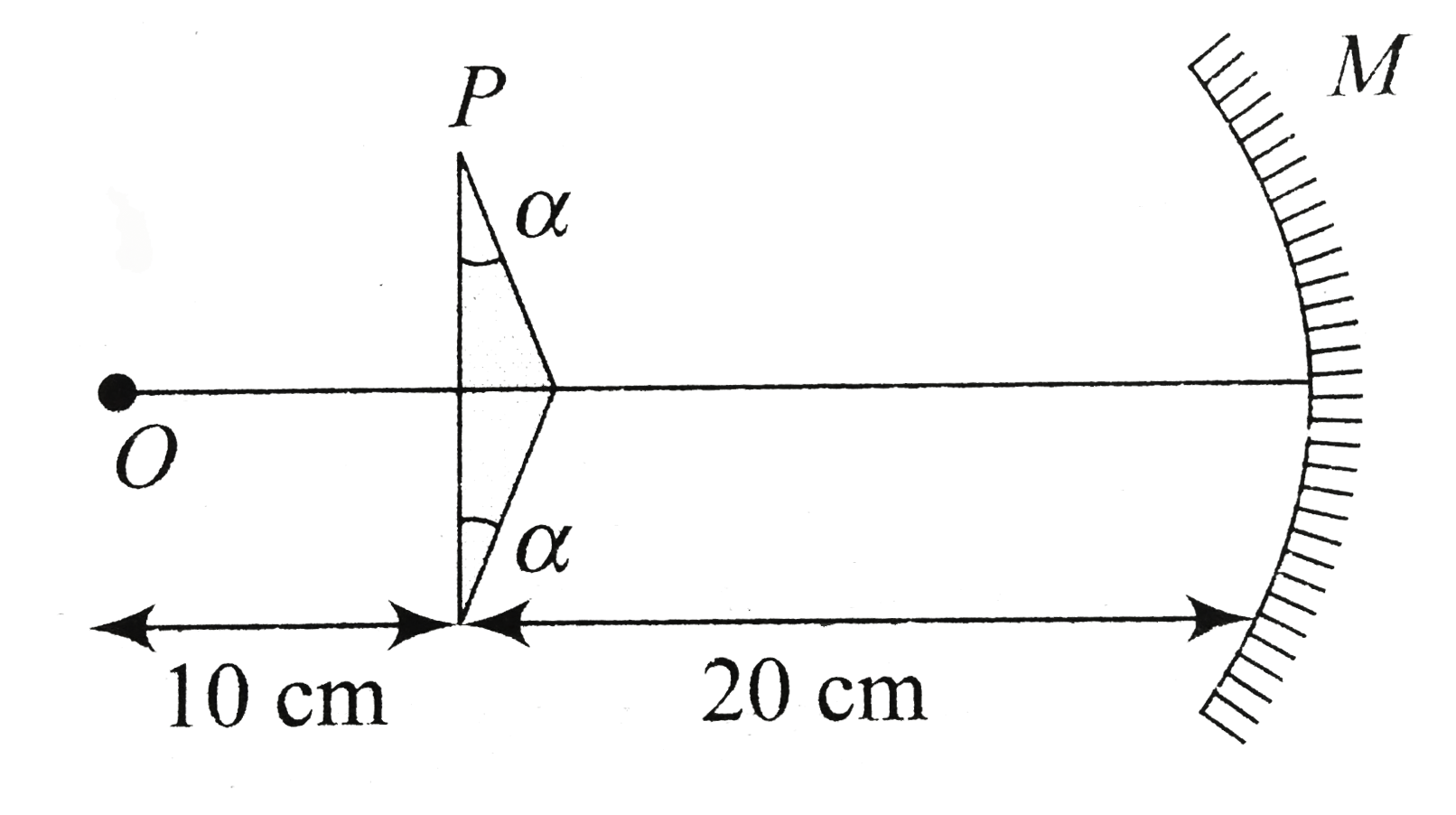
O is a point object kept on the principal axis of a concave mirror M of radius of curvature 20cm. P is a prism of angle 1.8∘ . Light falling on the prism (at small angle of incidence) gets refracted through the prism and then falls on the mirror. Refractive index of the prism is 3/2 . Find the distance between the images formed by the concave mirror due to this light.
View SolutionA ray of light falls on a glass prism at a angle of incidence of 30∘. Find the direction of the emergent ray if the angle of the prism is 60∘ and refractive index is 1.50
View Solution
Knowledge Check
The refractive index of a prism is 2. this prism can have a maximum refracting angle of
A90∘B60∘C45∘D30∘A ray of light is incident at an angle of incidence 45∘ on an equilateral prism and emerge at an angle 45∘ then the refractive index of the medium of the prism is:
A2B√2C1√2D√3A thin prism deviates an incident ray by 3.2∘. If the refractive index of the prism is 2.6 then the angle of prism is
A1∘B2∘C3∘D4∘
A thin prism of refracting angle 2∘ deviates an incident ray through an angle of 1∘.Find the value of refractive index of material of prism.
View SolutionA ray of light falling at an angle of 50∘ is refracted through a prism and suffers minimum deviation. The angle of the prism is 60∘. Find the angle of minimum deviation and refraction index of the material of the prism.
View SolutionA ray of light incident on a prism of angle 60∘, does not emerge out. Refractive index of material of prism may be
View SolutionA ray of monochromatic light is incident on the refracting face of a prism (angle 75∘0 . It passes through the prism and is incident on the other face at the critical angle. If the refractive index of the prism is √2 , then the angle of incidence on the first of the prism is
View SolutionLight falls at normal incidence on one face of a glass prism of refractive index √2. Then the angle of emergence when the angle of the prism is 45∘
View Solution