Focal length of a thin lens in air, is 10 cm. Now medium on one side of the lens is replaced by a medium of refractive index μ=2. The radius of curvature of surface of leng, in contact with the medium, is 20 cm. Find the point on principal axis where parallel rays incident on lens from air parallel to axis will converge.
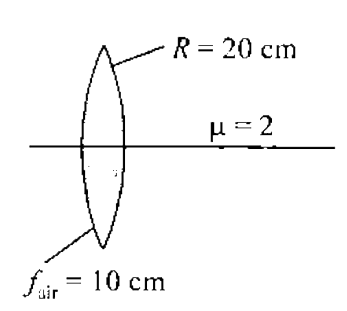
Let radius of 1 surface be R1 and refreactive index of lens be μ. Let parallel rays be incident on the lens. Appyling refractiion formula at first surface
μv1−1∞=μ−1R1.....(1)
At II surface 2v−μv1=2−μ−20....(2)
Adding (1) and (2)
μv1−1∞+2v−μv1=μ−1R(1)+2−μ−20
=(μ−1)(1R1−1−20)=μ−120−2−μ20=1f (in air) +120−220
⇒v=40cm⇒f=40cm
Topper's Solved these Questions
- GEOMATRICAL OPTICS Book:RESONANCEChapter:GEOMATRICAL OPTICS Exercise:Problem
Explore 33 Videos
- GEOMATRICAL OPTICS Book:RESONANCEChapter:GEOMATRICAL OPTICS Exercise:Exercise-1
Explore 142 Videos
- EXPERIMENTAL PHYSICSBook:RESONANCEChapter:EXPERIMENTAL PHYSICSExercise:PART -II
Explore 10 Videos
- GRAVITATIONBook:RESONANCEChapter:GRAVITATIONExercise:HIGH LEVEL PROBLEMS
Explore 16 Videos
Similar Questions
Focal length of a thin lens in air is 10cm. Now, medium on one side of the lens is replaced by a medium of refractive inidex μ=2. The radius of curvature of the surface of lens, in contact with the medium, is 20cm. Find the new focal length.
View SolutionA thin lens of refractive index 1.5 has focal length of 15cm in air. When the lens is placed is a medium of refractive index (4)/(3), its focal length will become …..cm.
View Solution
Knowledge Check
A planoconvex lens is made of refractive index 1.6. The radius of curvature of curved surface is 60 cm. The focal length of the lens is
A50 cmB100 cmC200 cmD400 cmA plano-convex lens is made of material having refractive index 1.5. The radius of curvature of curved surface is 60 cm. The focal length of the lens is ______ cm.
A−60B120C60D−120Focal length of convex lens in air is 16 cm (μglass=1.5). Now the lens is submerged in liquid of refractive index 1.42. Find the ratio of focal length in medium to focal length in air has closest value
A9B17C1D5
The refractive index of a converging lens is 1.4. What will be the focal length of this lens if it is placed in a medium of same refractive index? Assume the radii of curvature of the faces of lens are R1andR2 respectively.
View SolutionThe focal length of a thin convex-lens is 30cm. At a distance of 10 cm from the lens there is a plane refracting surface fo refractive inidex 3/2 Where will parallel rays incident on lens converge?
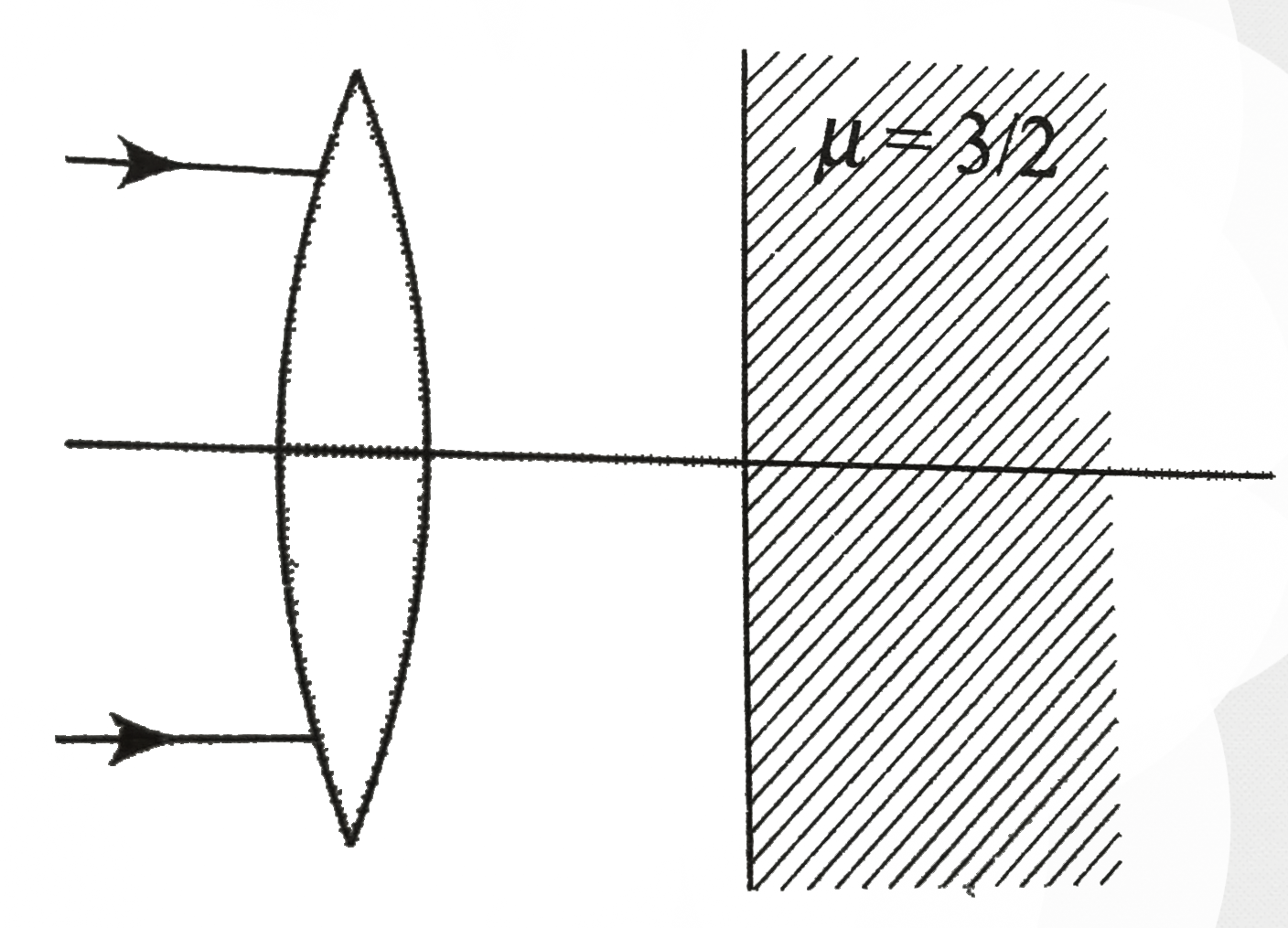
View SolutionThe radius of curvature of each surface of a lens is 40 cm. Its refractive index is 1.5. The focal length of lens will be
View SolutionFocal length of a thin convex lens is 30 cm. At distance of 10 cm from the lens there is a plan refracting surface of refractive index 3/2. Where w the parallel rays incident on lens converge ?
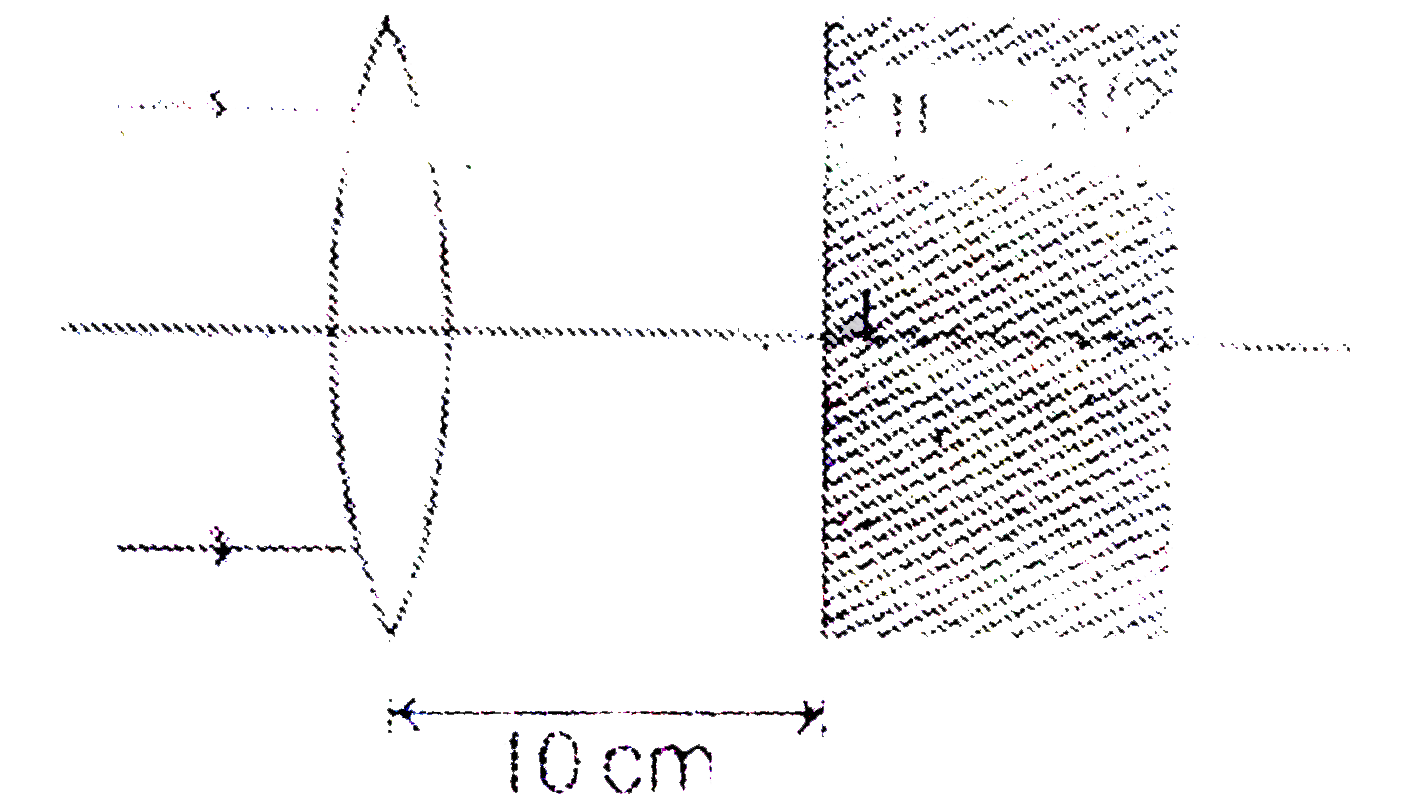
View SolutionThe focal length of a biconvex lens is 20 cm and its refractive index is 1.5. If the radii of curvatures of two surfaces of lens are in the ratio 1:2, then the larger radius of curvature is (in cm)
View Solution
