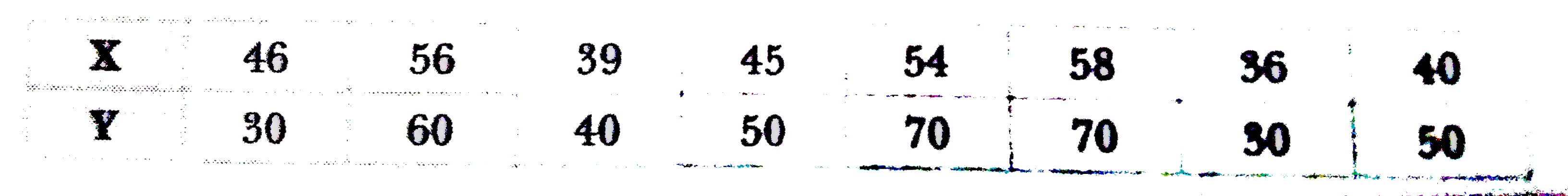The coefficient of correlation between X and Y is 0.6. Their covariance is 4.8 var (x)=9. Find the value of σy.
The correct Answer is:83
To find the value of the standard deviation of Y (denoted as σ_y), we can use the relationship between the coefficient of correlation (r), covariance (Cov(X,Y)), and the standard deviations of X and Y. The formula for the coefficient of correlation is given by:
r=Cov(X,Y)σx⋅σy
Where:
- r is the coefficient of correlation,
- Cov(X,Y) is the covariance between X and Y,
- σx is the standard deviation of X,
- σy is the standard deviation of Y.
Given in the question:
- r=0.6
- Cov(X,Y)=4.8
- Var(X)=9
Step 1: Calculate the standard deviation of X (σ_x).
Since the variance of X is given as 9, we can find the standard deviation by taking the square root of the variance:
σx=√Var(X)=√9=3
Step 2: Substitute the known values into the correlation formula.
Now we can substitute the known values into the correlation formula to find σ_y:
0.6=4.83⋅σy
Step 3: Rearranging the equation to solve for σ_y.
To isolate σ_y, we can rearrange the equation:
0.6⋅3⋅σy=4.8
1.8⋅σy=4.8
Step 4: Solve for σ_y.
Now, divide both sides by 1.8 to find σ_y:
σy=4.81.8
σy=4818=83≈2.67
Thus, the value of the standard deviation of Y (σ_y) is approximately 2.67.
- r is the coefficient of correlation,
- Cov(X,Y) is the covariance between X and Y,
- σx is the standard deviation of X,
- σy is the standard deviation of Y.
- r=0.6
- Cov(X,Y)=4.8
- Var(X)=9
Since the variance of X is given as 9, we can find the standard deviation by taking the square root of the variance:
Now we can substitute the known values into the correlation formula to find σ_y:
To isolate σ_y, we can rearrange the equation:
Now, divide both sides by 1.8 to find σ_y:
Topper's Solved these Questions
- STATISTICSBOOK - FIITJEECHAPTER - STATISTICSEXERCISE - Assignment Problems (Objective) Level -I
20 Videos
- STATISTICSBOOK - FIITJEECHAPTER - STATISTICSEXERCISE - Assignment Problems (Objective) Level II
25 Videos
- STATISTICSBOOK - FIITJEECHAPTER - STATISTICSEXERCISE - Exercise 3
2 Videos
- SET, RELATION & FUNCTIONBOOK - FIITJEECHAPTER - SET, RELATION & FUNCTIONEXERCISE - Exercise 3
8 Videos
- STRAIGHT LINE BOOK - FIITJEECHAPTER - STRAIGHT LINE EXERCISE - NUMERICAL BASED
4 Videos
Similar Questions
Knowledge Check
If the coefficient of correlation between x and y os 0.6, covariance is 27 and variance of y is 25, then what is the variance x?
A9/5B81/25C9D81The coefficient of correlation between random variables X and Y is 0.28, covariance between X and Y is 7.6 and the variance of X is 9, then the standard deviation of Y series is
A9.8B10.1C9.05D10.05The coefficient of correlation between X and Y is 0.6 U and V are two variables defined as U=x−32,V=y−23, then the coefficient of correlation between U and V is
A0.1B0.3C0.2D1
The co-efficient of correlation between two variables x and y is 0.5. Their co-variance is 15 and σx=6, Then σy=
View SolutionCoefficient of correlation between two variable X and Y is 0.32. Their covariance is 7.86. The variance of X is 10. Find the standard deviation of Y-series.
View SolutionFind the Kari Pearson's Coefficient of correlation between x and y for the data

View SolutionFind the coefficient of correlation e(X,Y), when cov (X,Y) =-14.6, var(X)=3.5 var(Y)=64.
View SolutionFind the coefficient of correlation between the values of x and y :
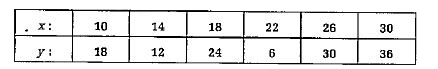
Note : Here,values in x, increase by the interval of 4 and values of y have the common factor 6.
View Solution