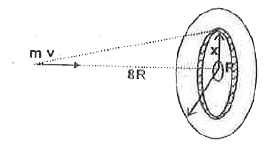
Space junk of mass m leaves its orbit and heads towards on annular disc-shaped meteor at rest relative to earth along its axis at a distance 8R with a velocity v. The surface mass density of the meteor is σ and its inner and outer radii are R and 4R. Find the velocity with which the junk passes the centre of the meteor. (Thickness of the meteor is negligible).
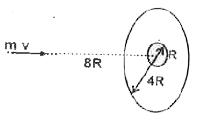
Consider an elemental ring of radius r and width dr.
We have potential energy of the system (ring + junk)
dE1=−Gmdm(x2+r2)1/2=GM2πdrσ(x2+r2)1/2
E1=−2πGmσ∫R2R1rdr(x2+r2)1/2
Substituting , x2+r2=t
2rdr=dt, rdr=dt/2
E1=−2πGmσ∫dt2(t)1/2=−2πGmσ∣∣(x2+r2)1/2∣∣R2R1
=−2πGmsimga[(x2+R22)1/2−(x2+R21)1/2].........(i)
Ath the centre E2=−2πGmσ(R2−R1).........(ii)
The initial kinetic energy of the junk E3=12mv2
Let the velocity of the junk at the centre be v. then E4=12Mv2
Conserving energy, E1+E3=E2+E4
⇒−2πGmσ[(x2+R22)1/2−(x2+R21)1/2]+12mv2=−2πGmσ(R2−R1)+12mv2
⇒−2πGσ[(64R2+16R2)1/2−(64R2+R2)1/2]+12v2=−2πGσ(3R)+12v.2
⇒v.={4πGσ[3−√5(4−√13)]R+v2}1/2
Topper's Solved these Questions
- GRAVITATIONBook:FIITJEEChapter:GRAVITATIONExercise:Solved problem (Objective)
Explore 15 Videos
- GRAVITATIONBook:FIITJEEChapter:GRAVITATIONExercise:Comprehension
Explore 13 Videos
- GRAVITATIONBook:FIITJEEChapter:GRAVITATIONExercise:Numerical based Question
Explore 2 Videos
- GMP ASSESMENTBook:FIITJEEChapter:GMP ASSESMENTExercise:Numerical Based
Explore 61 Videos
- HEAT AND TEMPERATUREBook:FIITJEEChapter:HEAT AND TEMPERATUREExercise:NUMERICAL BASES QUESTIONS
Explore 1 Video
Similar Questions
Calculate the moment of intertia of an annular disc about an axis which lies in the plane of the disc and tangential to the outer circle. Mass of the disc is M and its inner radius is R1 and outer rad/R2.
View SolutionCalculate the moment of intertia of an annular disc about an axis which lies in the plane of the disc and tangential to the inner circle. Mass of the disc is M and its inner radius is R1 and outer rad/R2.
View Solution
Knowledge Check
The moment of inertia of an annular disc of mass M, outer and inner radii R and r, about its diameter is
AM(R2+r2)/2BMR2CMr2DMR2+r24The moment of inertia of an annular disc of mass M, outer and inner radii R and r, about its diameter is
AM(R2+r2)/2BMR2CMr2DMR2+r24Assume the earth's orbit around the sun as circular and the distance between their centres as D Mass of the earth is M and its radius is R if earth has an angular velocity ω0 with respect to its centre and ω with respect to the centre of the sun, the total kinetic energy of the earth is:
AMR2ω205[1+(ω(ω0)2+52)Dω(Rω0)2]BMR2ω205[1+52(DωRω0)2]C52MR2ω20[1+52(DωRω0)2]D25MR2ω20[1+(ωω0)2+52(DωRω0)2]
The mass of a thin circular plate is M and its radius is R. About an axis in the plane of plate at a perpendicular distance R/2 from centre of plate, its moment of inertia is
View SolutionA particle of mass m is placed at a distance R from centre of a uniformly dense thin ring of mass m rand radius R on a axis passing through its centre and perpendicular to its plane. Find the speed of the particle when it reaches at the centre fo the ring due to their mutual gravitation force.
View SolutionA dielectric disc of radius R and uniform positive surface charge density σ is placed on the grup with its axis vertical. A particle of mass m and positive charge q is dropped, along the axis of the disc from a height H with dropped, along the axis of the disc from a height H with zero intial velocity. The charge -mass ratio of the particle is qm=4εon0gσ.
(i). Find the value of H if the particle just reaches the disc.
(ii). Sketch the potential energy of the particle as a function of its height and find its height in equilibrium position.
View SolutionA non-conducting thin disc of radius R charged uniformly over one side with surface density σ, rotates about its axis with an angular velocity ω. Find (a) the magnetic field induction at the centre of the disc (b) the magnetic moment of the disc.
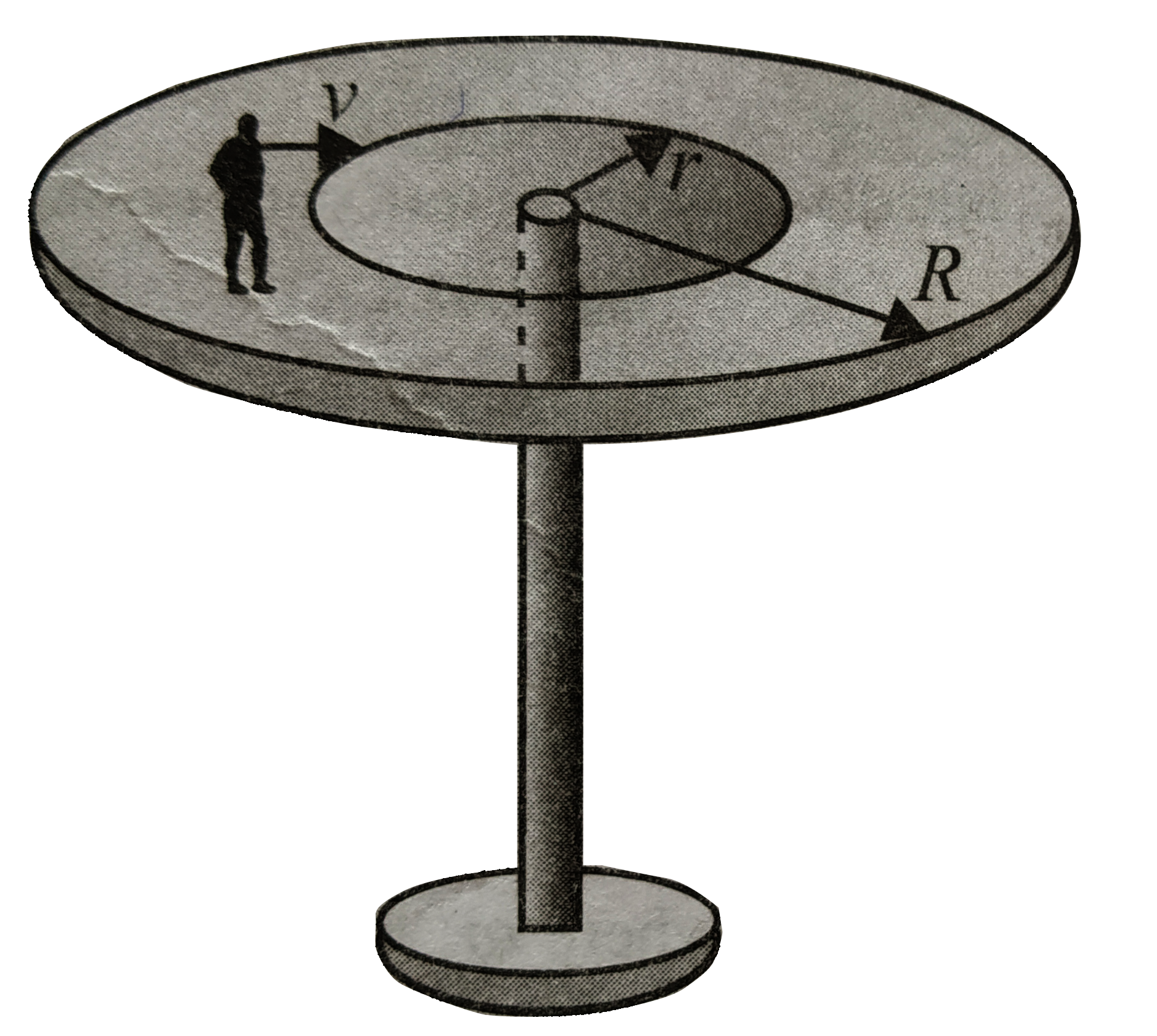
View SolutionA man of mass m stands on a horizontal platform in the shape of a disc of mass m and radius R, pivoted on a vertical axis through its centre about which it can freely rotate. The man starts to move around the centre of the disc in a circle of radius r with a velocity v relative to the disc. Calculate the angular velocity of the disc.
View Solution