The velocity of liquid flowing through a tube at certain distance from the axis of tube
increases from zero to maximum value
decreases from maximum value to zero
remains same throughout the cross section
increases from zero to a maximum value on one side and decreases from a maximum value to zero on the other side .
The correct Answer is:B
Step by step video, text & image solution for The velocity of liquid flowing through a tube at certain distance from the axis of tube by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- FLUID MECHANICS BOOK - FIITJEECHAPTER - FLUID MECHANICS EXERCISE - ASSIGHNMENT PROBLEMS (OBJECTIVE ) ASSERTION REASONING TYPE LEVEL -I
1 Videos
- FLUID MECHANICS BOOK - FIITJEECHAPTER - FLUID MECHANICS EXERCISE - ASSIGHNMENT PROBLEMS (OBJECTIVE ) ASSERTION REASONING TYPE LEVEL-I
1 Videos
- FLUID MECHANICS BOOK - FIITJEECHAPTER - FLUID MECHANICS EXERCISE - ASSIGHNMENT PROBLEMS (SUBJECTIVE ) LEVEL - II & III
15 Videos
- ELECTROSTATICSBOOK - FIITJEECHAPTER - ELECTROSTATICSEXERCISE - Example
14 Videos
- GENERAL PHYSICSBOOK - FIITJEECHAPTER - GENERAL PHYSICSEXERCISE - MATRIX MATCH TYPE
3 Videos
Similar Questions
Assuming that the critical velocity of flow of a liquid through a narrow tube depends on the radius of the tube, density of the liquid and viscosity of the liquid, find an expression for critical velocity.
View SolutionWhat happens when the velocity of the liquid flowing through a horizontal tube is gradually increased?
View Solution
Knowledge Check
Consider streamline flow of a liquid flowing through a tube as shown in the figure which of the following is correct regarding velcities of liquid at different points ?
 Av1= constant ,v2= constant ,v3= constantBv1≠v2≠v3Cv1=v2=v3DBoth (a) and (b) current
Av1= constant ,v2= constant ,v3= constantBv1≠v2≠v3Cv1=v2=v3DBoth (a) and (b) currentA tube of length 1 and radius R carries a steady flow of fluid whose density is ρ and viscosity η. The velocity v of flow is given by v=v0(1−r2/R2) Where r is the distance of flowing fluid from the axis.
A4πηlV0B2πηlV0CπηlV0D23πηlV0Figure shows a liquid flowing through a tube at the rate of 0.1m3/s. The tube is branched into two semicircular tubes of cross-sectional area A/3 and 2A/3. The velocity of liquid at Q is (the cross section of the main tube is A=10−2m2 and vp=20m/s)
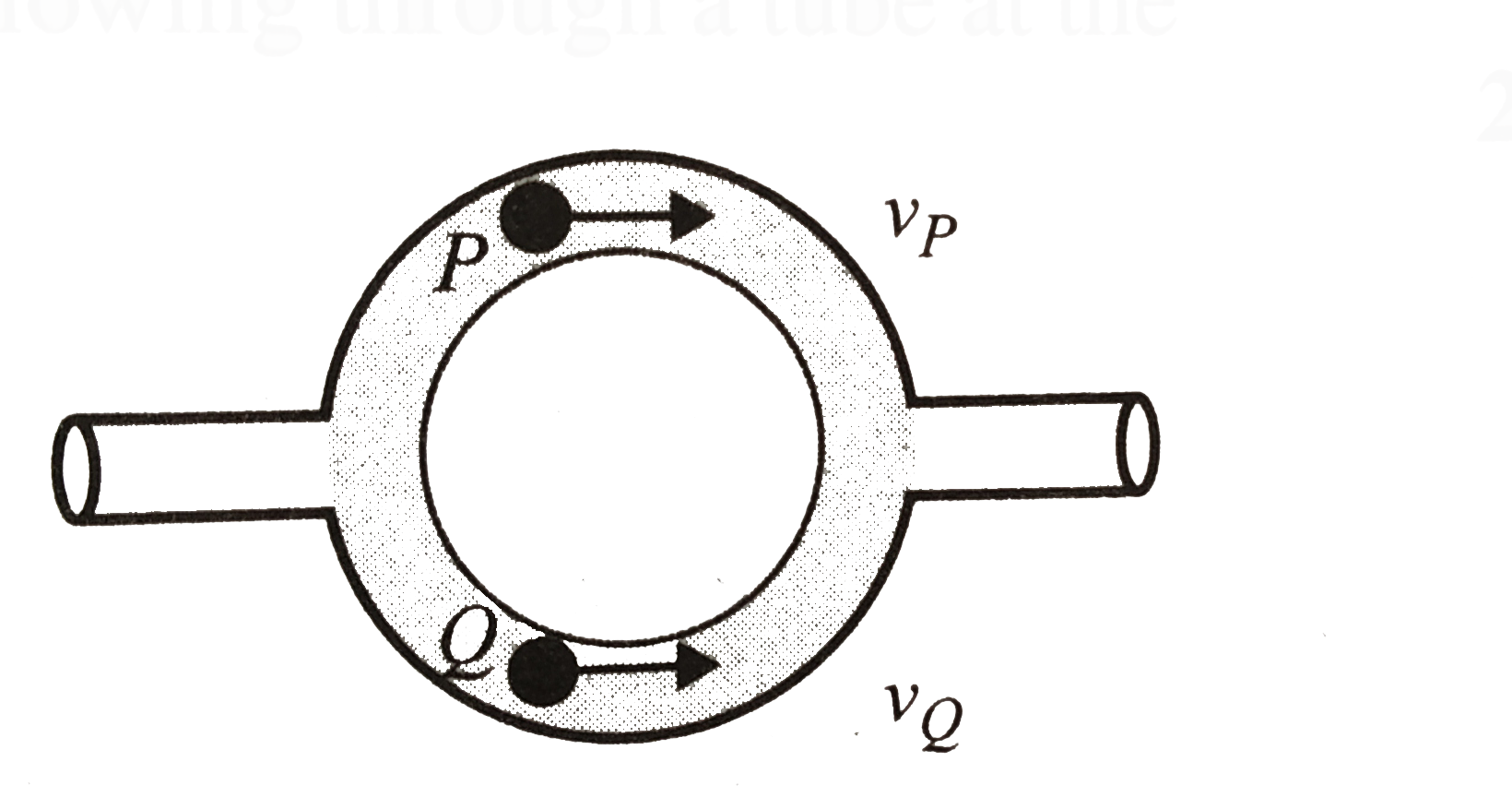 A5m/sB30m/sC35m/sDnone of these
A5m/sB30m/sC35m/sDnone of these
The liquid is flowing steadily through a tube of varying diameter. How are the velocity of liquid flow (V) in any portion and the diameter (D) of the tube in that portion are related?
View SolutionA tube of length l and radius R carries a steady flow of fluid whose density is ρ and viscosity η. The fluid flow velocity depends on the distance r from the axis of the tube as v=v0(1−r2/R2). Find:
(a) the volume of the fluid flowing across the section of the tube per unit time:
(b) the kinetic energy of the fluid within the tube's volume,
(c) the friction force exerted on the tube by the fluid,
(d) the pressure difference at the ends of the tube.
View SolutionThe rate of flow of a liquid through a capillary tube is
View SolutionTurpentine oil is flowing through a tube of length I and radius r. The pressure difference between the two ends of the tube is P , the visocity of oil at a distance x from the axis of tube from this relation, the dimensions of viscosity are :
View SolutionCurrent flows through a straight, thin-walled tube of radius r. The magnetic field at a distance x from the axis of the tube has magnitude B.
View Solution
