A body of mass m is pushed with the initial velocity v0 up an inclined plane of angle of inclination θ. The co-efficient or friction between the body and the plane is μ. What is the net work done by fnction during the ascent of the body before it comes to stop ?
The correct Answer is:μmv202(tanθ+μ)
To find the net work done by friction during the ascent of a body of mass m pushed up an inclined plane with an initial velocity v0, we can follow these steps:
Step 1: Understand the Forces Acting on the Body
The forces acting on the body as it moves up the incline include:
1. The gravitational force acting downwards, which can be resolved into two components:
- mgsinθ (parallel to the incline)
- mgcosθ (perpendicular to the incline)
2. The frictional force, which opposes the motion of the body. The frictional force f is given by:
f=μN=μ(mgcosθ)
Step 2: Apply the Work-Energy Theorem
According to the work-energy theorem, the work done by all forces is equal to the change in kinetic energy:
Wnet=Kf−Ki
Where:
- Kf is the final kinetic energy (0, since the body comes to rest)
- Ki is the initial kinetic energy, given by:
Ki=12mv20
Thus, the net work done is:
Wnet=0−12mv20=−12mv20
Step 3: Calculate Work Done by Gravity
The work done by gravity as the body moves a distance L up the incline is:
Wgravity=−mgsinθ⋅L
Step 4: Calculate Work Done by Friction
The work done by friction can be expressed as:
Wfriction=Wnet−Wgravity
Substituting the expressions we have:
Wfriction=−12mv20−(−mgsinθ⋅L)
This simplifies to:
Wfriction=−12mv20+mgsinθ⋅L
Step 5: Find the Distance L
Using the kinematic equation, we can relate the distance L to the initial velocity v0 and the acceleration a (which is negative due to deceleration):
v2=u2+2aL
Setting v=0 (final velocity), u=v0, and a=−gsinθ−μgcosθ:
0=v20−2(gsinθ+μgcosθ)L
From this, we can solve for L:
L=v202(gsinθ+μgcosθ)
Step 6: Substitute L Back into the Work Done by Friction
Now substituting L back into the work done by friction:
Wfriction=−12mv20+mgsinθ⋅v202(gsinθ+μgcosθ)
Step 7: Simplify the Expression
Factoring out 12mv20:
Wfriction=−12mv20(1−gsinθgsinθ+μgcosθ)
This leads to:
Wfriction=−12mv20(μgcosθgsinθ+μgcosθ)
Final Result
The net work done by friction during the ascent of the body before it comes to stop is:
Wfriction=−μmv202cosθ(tanθ+μ)
The forces acting on the body as it moves up the incline include:
1. The gravitational force acting downwards, which can be resolved into two components:
- mgsinθ (parallel to the incline)
- mgcosθ (perpendicular to the incline)
2. The frictional force, which opposes the motion of the body. The frictional force f is given by:
f=μN=μ(mgcosθ)
According to the work-energy theorem, the work done by all forces is equal to the change in kinetic energy:
Wnet=Kf−Ki
Where:
- Kf is the final kinetic energy (0, since the body comes to rest)
- Ki is the initial kinetic energy, given by:
Ki=12mv20
Wnet=0−12mv20=−12mv20
The work done by gravity as the body moves a distance L up the incline is:
Wgravity=−mgsinθ⋅L
The work done by friction can be expressed as:
Wfriction=Wnet−Wgravity
Substituting the expressions we have:
Wfriction=−12mv20−(−mgsinθ⋅L)
This simplifies to:
Wfriction=−12mv20+mgsinθ⋅L
Using the kinematic equation, we can relate the distance L to the initial velocity v0 and the acceleration a (which is negative due to deceleration):
v2=u2+2aL
Setting v=0 (final velocity), u=v0, and a=−gsinθ−μgcosθ:
0=v20−2(gsinθ+μgcosθ)L
From this, we can solve for L:
L=v202(gsinθ+μgcosθ)
Now substituting L back into the work done by friction:
Wfriction=−12mv20+mgsinθ⋅v202(gsinθ+μgcosθ)
Factoring out 12mv20:
Wfriction=−12mv20(1−gsinθgsinθ+μgcosθ)
This leads to:
Wfriction=−12mv20(μgcosθgsinθ+μgcosθ)
The net work done by friction during the ascent of the body before it comes to stop is:
Wfriction=−μmv202cosθ(tanθ+μ)
Topper's Solved these Questions
- WORK, ENERGY AND POWER Book:FIITJEEChapter:WORK, ENERGY AND POWER Exercise:ASSIGNMENT PROBLEMS ( SUBJECTIVE) (LEVEL-II & III)
Explore 15 Videos
- WORK, ENERGY AND POWER Book:FIITJEEChapter:WORK, ENERGY AND POWER Exercise:ASSIGNMENT PROBLEMS ( OBJECTIVE) (LEVEL-I)
Explore 36 Videos
- WORK, ENERGY AND POWER Book:FIITJEEChapter:WORK, ENERGY AND POWER Exercise:ASSIGNMENT PROBLEMS ( SUBJECTIVE) (LEVEL-I) True or False Type Questions
Explore 3 Videos
- TEST PAPERSBook:FIITJEEChapter:TEST PAPERSExercise:PHYSICS
Explore 747 Videos
Similar Questions
A body is moving down along inclined plane of angle of inclination theta The coefficient of friction between the body and the plane varies as mu=0.5x where x is the distance moved down the plane. The body will have the maximum velocity when
View SolutionA block of mass m is projected up with a velocity v0 along an inclined plane of angle of inclination θ=37∘. The coefficient of friction between the inclined plane AB and blocks is μ(=tanθ). Find the values of v0 so that the block moves in a circular path from B to C.
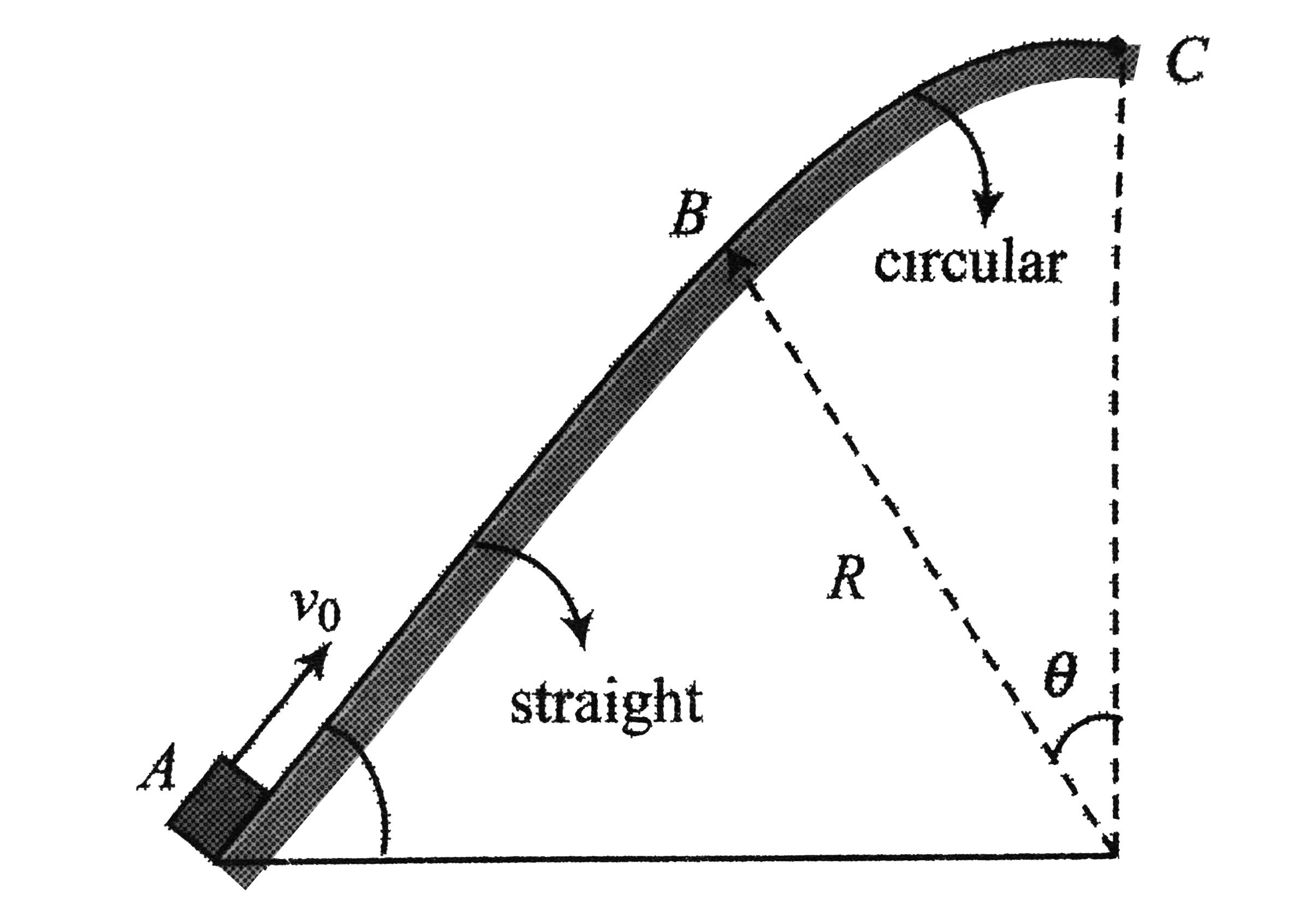
View Solution
Knowledge Check
A body is moving up an inclined plane of angle θ with an anitial kinetic energy E. The coefficient of friction between the plane and body is μ. The work done against friction before the body comes to rest is
AμEcosθcosθ+sinθBECμEcosθμcosθ−sinθDμEcosθμcosθ+sinθA body of mass 0.5 kg is taken up an inclined plane of length 10 m and height 5 m and then allowed to slide down to the bottom again. The coefficient of friction between the body and the plane is 0.1. The work done by the frictional force over the round trip is
A5 JB5√3JC−5JD−5√3JA body is moving down a long inclined plane of angle of inclination θ. The coefficient of friction between the body and the plane varies as μ−0.1x, where x is the distance moved down the plane. The body will have the maximum velocity when it has travelled a distance x given by :
Ax=10tanθBx=5tanθC√2cotθDx=√10cotθ
A block of mass m slides from the top of an inclined plane of angle of inclination θ & length l. The coefficient of friction between the plane and the block is μ. Then it is observed over a distance d along the horizontal surface having the same coefficient of friction μ, before it comes to a stop. Find the value of d.
View SolutionA block of mass m is at rest on a rough inclined plane of angle of inclination θ. If coefficient of friction between the block and the inclined plane is μ, then the minimum value of force along the plane required to move the block on the plane is
View SolutionA body of mass 0.3kg is taken up an inclined plane of length 10m and height 5m, and then allowed to slide down to the bottom again. The coefficient of friction between the body and the plane is 0.15.
What is the
(a) work done by the gravitational force over the round trip ?
(b) work done by the applied force on the upward journey ?
(c) work done by the frictional force over the round trip ,
(d)kinetic energy of the body at the end of the trip ?
View SolutionA body of mass m is pushed with the initial velocity v0 up an inclined plane set at an angle α to the horizontal. The friction coefficient is equal to k. What distance will the body cover before it stops and what work do the friction forces perform over this distance?
View SolutionA body with zero initial velocity slips from the top of an inclined plane forming an angle α with the horizontal. The coefficient of friction μ between the body and the plane increases with the distance l from the top according to the law μ=bl. The body stops before it reaches the end of the plane. Determine the time t from the beginning of motion of the body to the moment when it comes to rest.
View Solution