A charged ball of mass m and charge q is projected from the ground with an initail velocity u and angle of projection θ at the time of projection vertically upward electric field E is switched on. When it reaches the maximum height electric filed is switched off and a horizontal electric feild of same magnitude E is switched on. Then answer the following question (Assume mg> qE)
Q If the time of flight to the maximum height is t1 and the time of flight maximum height to the ground is t2 then
t1=t2
t1<t2
t1>t2
none of these
The correct Answer is:C
To solve the problem, we need to analyze the motion of the charged ball in two phases: the upward motion under the influence of gravity and the electric field, and the downward motion after the electric field is switched.
1. Determine the time to reach maximum height (t₁):
The time taken to reach the maximum height can be calculated using the formula:
t1=usinθg+qEm
Here, u is the initial velocity, θ is the angle of projection, g is the acceleration due to gravity, q is the charge of the ball, E is the electric field strength, and m is the mass of the ball.
2. Determine the time of flight from maximum height to the ground (t₂):
After reaching the maximum height, the electric field is switched off, and the ball falls under the influence of gravity alone. The time taken to fall from maximum height back to the ground can be calculated using the formula:
t2=√2hg
where h is the maximum height reached by the ball. The maximum height can be calculated using:
h=(usinθ)22(g+qEm)
Thus, substituting h into the equation for t2:
t2=
⎷2⋅(usinθ)22(g+qEm)g=usinθ√g(g+qEm)
3. Relate t₁ and t₂:
Now, we can express the relationship between t1 and t2:
t1=usinθg+qEm,t2=usinθ√g(g+qEm)
To find a relationship between t1 and t2, we can take the ratio:
t1t2=√g(g+qEm)g+qEm
Final Answer:
The time of flight to the maximum height t1 and the time of flight from maximum height to the ground t2 can be expressed in terms of the initial velocity, angle of projection, electric field, and gravitational acceleration.
The time taken to reach the maximum height can be calculated using the formula:
t1=usinθg+qEm
Here, u is the initial velocity, θ is the angle of projection, g is the acceleration due to gravity, q is the charge of the ball, E is the electric field strength, and m is the mass of the ball.
After reaching the maximum height, the electric field is switched off, and the ball falls under the influence of gravity alone. The time taken to fall from maximum height back to the ground can be calculated using the formula:
t2=√2hg
where h is the maximum height reached by the ball. The maximum height can be calculated using:
h=(usinθ)22(g+qEm)
Thus, substituting h into the equation for t2:
t2=
⎷2⋅(usinθ)22(g+qEm)g=usinθ√g(g+qEm)
Now, we can express the relationship between t1 and t2:
t1=usinθg+qEm,t2=usinθ√g(g+qEm)
To find a relationship between t1 and t2, we can take the ratio:
t1t2=√g(g+qEm)g+qEm
The time of flight to the maximum height t1 and the time of flight from maximum height to the ground t2 can be expressed in terms of the initial velocity, angle of projection, electric field, and gravitational acceleration.
Topper's Solved these Questions
- KINEMATICSBOOK - FIITJEECHAPTER - KINEMATICSEXERCISE - Assertion reason type
1 Videos
- KINEMATICSBOOK - FIITJEECHAPTER - KINEMATICSEXERCISE - Numerical based question
4 Videos
- KINEMATICSBOOK - FIITJEECHAPTER - KINEMATICSEXERCISE - Comprehension (I)
3 Videos
- HEAT AND TEMPERATUREBOOK - FIITJEECHAPTER - HEAT AND TEMPERATUREEXERCISE - NUMERICAL BASES QUESTIONS
1 Videos
- LAWS OF MOTIONBOOK - FIITJEECHAPTER - LAWS OF MOTIONEXERCISE - COMPREHENSION-III
2 Videos
Similar Questions
A particle having mass m and charge q is projected with a velocity v making an angle q with the direction of a uniform magnetic field B. Calculate the magnitude of change in velocity of the particle after time t=πm2qB.
View SolutionA charged particle of mass m and charge q is released from rest in an electric field of constant magnitude E. The kinetic energy of the particle after time t is
View Solution
Knowledge Check
A charged ball of mass m and charge q is projected from the ground with an initail velocity u and angle of projection θ at the time of projection vertically upward electric field E is switched on. When it reaches the maximum height electric filed is switched off and a horizontal electric feild of same magnitude E is switched on. Then answer the following question (Assume mg> qE)
Q In the above experimentAhorizionatl velocity always remains constantBhorizontal velocity remains constant for hall of the time and then varies.Chorizontal velocity when it strikes the grounds is greater than the intial horizontal velocityDthe initial vertical speed is equal to the final vertical speede.A ball is projected from the ground with velocity u such that its range is maximum Then
AIts velocity at half the maximum height is √32uBIts velocity at the maximum height is uCChange in its velocity when it returns to the ground is uDall the above are trueA ball of mass m is projected from the ground with an initial velocity u making an angle of θ with the vertical. What is the change in velocity between the point of projection and the highest point ?
Aucosθ downwardBucosθ upwardCusinθ upwardDusinθ downward
A charged particle of mass m and charge q is released from rest in an electric field of constant magnitude E. The kinetic energy of the particle after time t is
View SolutionA body is projected with velocity u at an angle of projection θ with the horizontal. The direction of velocity of the body makes angle 30∘ with the horizontal at t=2s and then after 1s it reaches the maximum height. Then
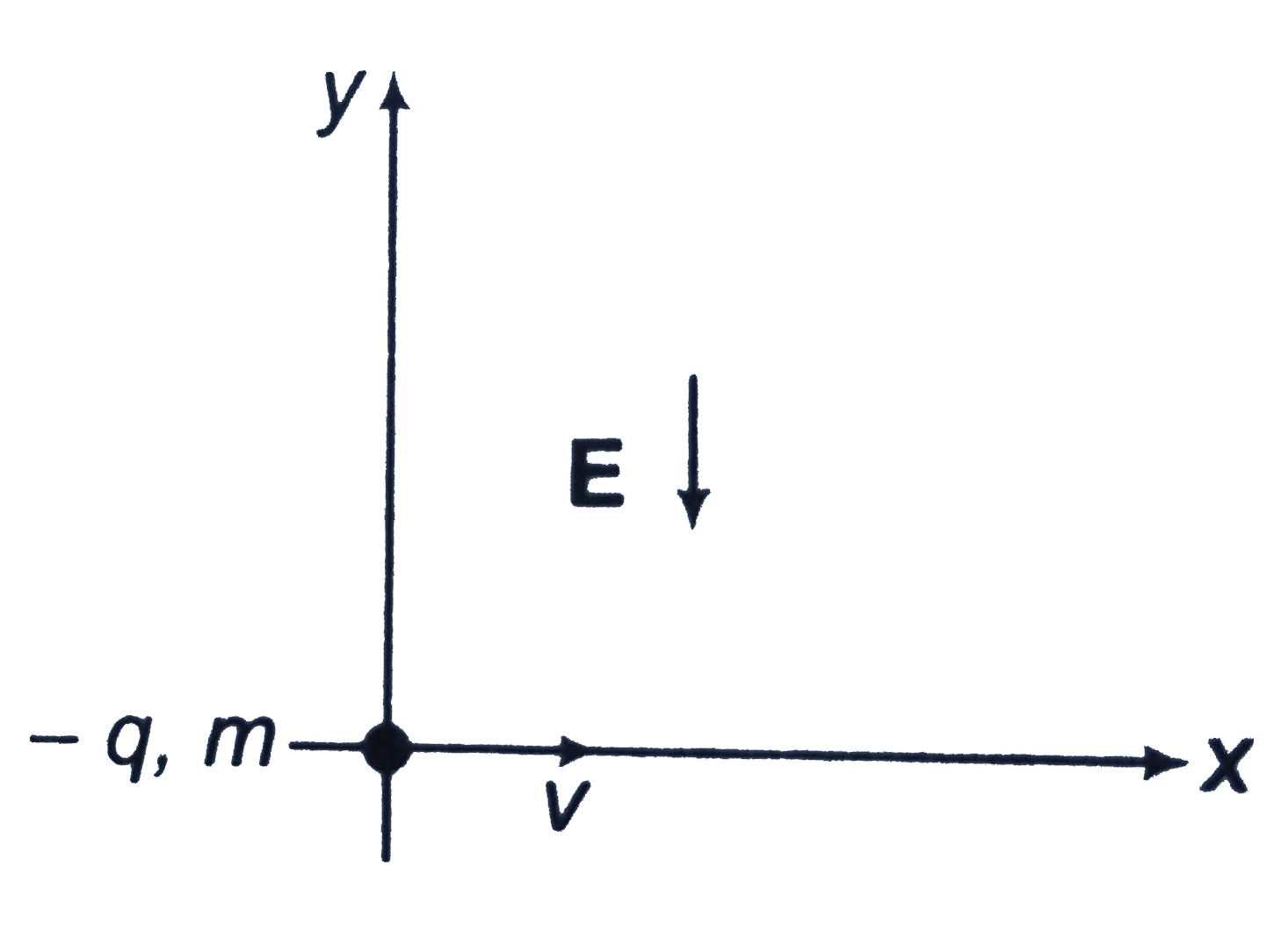
View SolutionA particle of mass m and charge −q is projected from the origin with a horizontal speed v into an electric field of intensity E directed downward. Choose the wrong statement. Neglect gravity
View SolutionA particle of mass m is projected with an initial velocity U at an angle θ to the horizontal. The torque of gravity on projectile at maximum height about the point of projection is
View SolutionA body projected vertically upwards with a velocity of 19.6m/s reaches a height of 19.8 m on earth. If it is projected vertically up with the same velocity on moon, then the maximum height reached by it is
View Solution
