AB is a diameter of a circle. AHandBK are perpendiculars from A and B respectively to the tangent at P Prove that AH+BK=AB.
Let AH=x,BK=yandBM=z
△MBK~△MAH (AA corollary)
∴ BKAH=BMAM ⇒ yx=z2r+z
∴ 2ry+yz=xz ⇒ z(x−y)=2ry
⇒ z=2ryx−y ...(1)
Similarly,
ΔMBK~ΔMOP (AA corollary)
∴ BKOP=BMOM ⇒ yr=zz+r
⇒ yz+yr=zr
⇒ z(r−y)=yr
⇒ z=yrr−y ...(2)
From (1) and (2), we get
2ryx−y=yrr−y ⇒ 2x−y=1r−y
⇒ 2r−2y=x−y
⇒ x+y=2r
AH+BK=AB Hence Proved.
Topper's Solved these Questions
- CIRCLESBOOK - NAGEEN PRAKASHANCHAPTER - CIRCLESEXERCISE - Problems From NCERT/exemplar
11 Videos
- CIRCLESBOOK - NAGEEN PRAKASHANCHAPTER - CIRCLESEXERCISE - Exercise
25 Videos
- ARITHMETIC PROGRESSIONBOOK - NAGEEN PRAKASHANCHAPTER - ARITHMETIC PROGRESSIONEXERCISE - Revision Exercise Long Answer Question
5 Videos
- CO-ORDINATE GEOMETRYBOOK - NAGEEN PRAKASHANCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - Revision Exercise Short Answer Questions
9 Videos
Similar Questions
AB is a diameter of a circle. P is a point on the semi-circle APB.AHandBK are perpendiculars from AandB respectively to the tangent at P. Prove that AH+BK=AB.
View SolutionIn the given figure, AB is a diameter of a circle and CD is perpendicular to AB, if AB = 10 cm and AE = 2 cm, then what is the length of ED ?
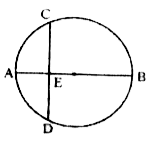
View Solution
Knowledge Check
AB is a diameter of the circle x2+y2=4. If p1 and p2 be the lengths of perpendiculars from A and B on the line x+y=1, then maximum value of p1p2 is:
A1/2B7/2C1D2In the given figure, CD and AB are diameters of circle and AB and CD are perpendicular to each other. LQ and SR are perpendiculars to AB and CD respectively. Radius of circle is 5 cm, PB: PA = 2:3 and CN:ND = 2:3. What is the length (in cm) of SM?
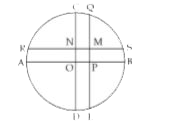 A[(5√3)−3]B[(4√3)−2]C[(2√5)−1]D[(2√6)−1]
A[(5√3)−3]B[(4√3)−2]C[(2√5)−1]D[(2√6)−1]
AB is a diameter of a circle. CD is a chord parallel to AB and 2CD=AB. The tangent at B meets the line AC produced at E then AE is equal to -
View SolutionAB is a diameter of a circle. CD is a chord parallel to AB and 2CD = AB. The tangent at B meets the line AC produced at E then AE is equal to
View SolutionIn the given figure, CD and AB are diameters of circle and AB and CD are perpendicular to each other .LQ and SR are perpendiculars to AB and CD respectively . Radius of circle is 5 cm , PB : PA = 2 : 3 and CN : ND = 2 : 3 . What is the length (in cm) of SM ?

View SolutionIn the given figure, CD and AB are diameters of circle and AB and CD are perpendicular to each other. LQ and SR are perpendiculars to AB and CD respectively. Radius of circle is 5 cm, PB: PA = 2:3 and CN:ND = 2:3. What is the length (in cm) of SM?

View SolutionAB is a diameter of circle. P is point on circle and a perpendicular line is drawn on AB at N from it. If radius is 7 cm. and PB = 12 cm. then BN is (in cm)-
View SolutionProve that a diameter AB of a circle bisects all those chords which are parallel to the tangent at the point A.
View Solution