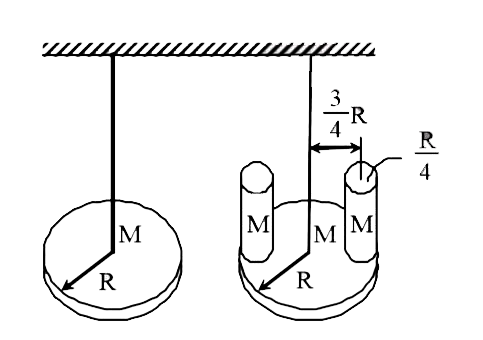
The two torsion pendula differ only by the addition of cylindrical masses as shown in the figure. The radius of each additional mass is 1/4 the radius fo the disc. Each cylinder and disc have equal mass. The ratio of time periods of the two torsion pendula is p. Then 27p2 is.
_E01_110_Q01.png)
I1=MR22
I2=[M2(R4)2+M(3R4)2]+[M2(R4)2+M(3R4)2]+MR22
T∝√1
T1T2∝√I1I2
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-05 [A]
39 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-05 [B]
12 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Comprehension Base Questions (5)
3 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
25 Videos
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
Similar Questions
Find the ratio of the periods of the two torsion pendula shown in figure. The two differ only by the addition of cylindrical masses as shown in the figure. The radius of each additional mass is 1/4 the radius of the disc. Each cylinder and disc have equal mass.
View SolutionTwo satellites having their masses in the ratio of 4:9 are revolving in orbits of radius R. The ratio of their time periods will be
View Solution
Knowledge Check
Find the position of center of mass of the uniform lamina shown in figure, if small disc of radius a2 is cut from disc of radius a.
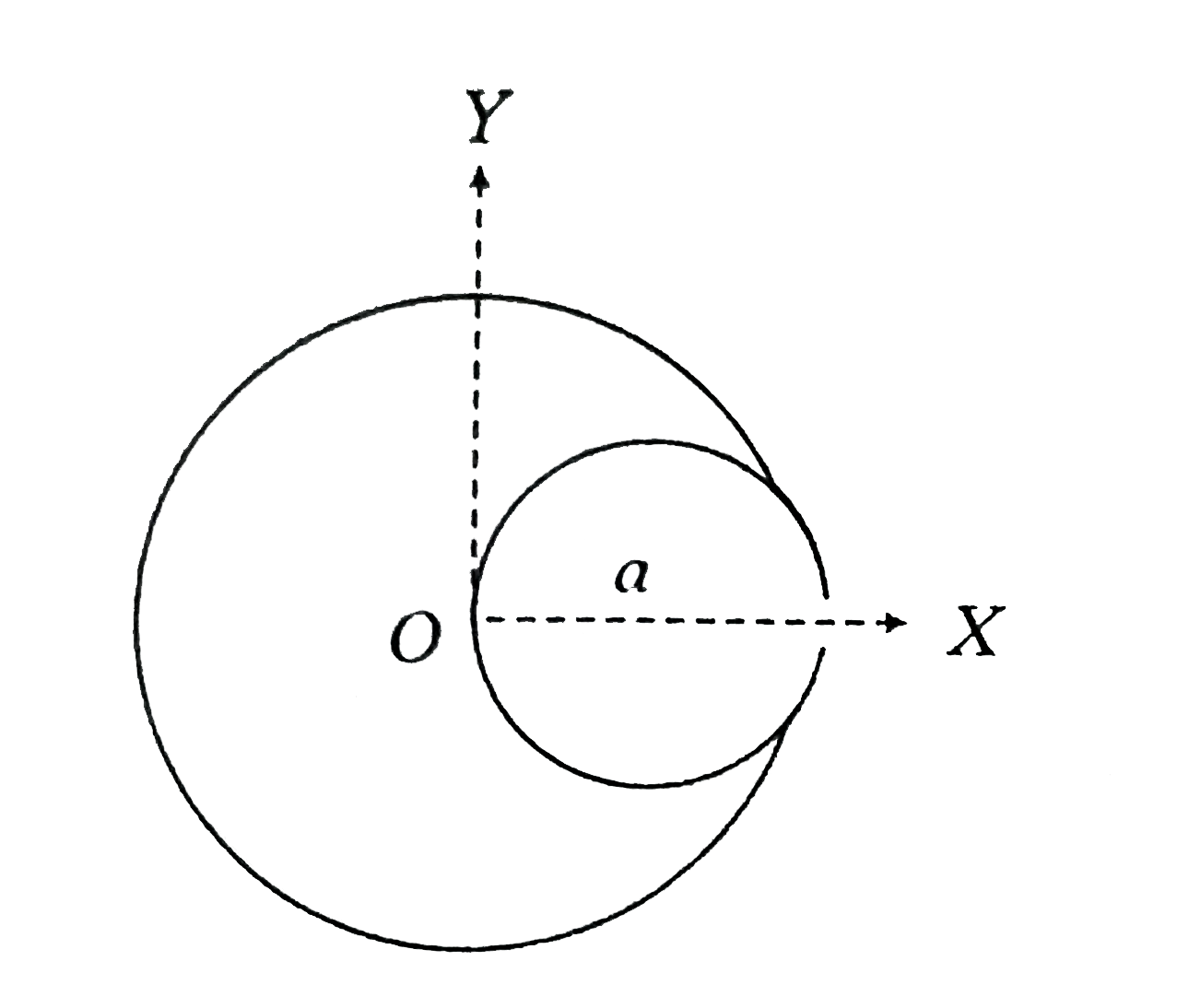 A(−a6,a)B(−a2,0)C(−a6,0)D(−a3,0)
A(−a6,a)B(−a2,0)C(−a6,0)D(−a3,0)The ratio of the radii of gyration of a circular disc to that of a circular ring, each of same mass and radius, around their respective axes is.
A√2:√3B√3:√2C1:√2D√2:1
Two identical discs of mass m and of radius R touch each other and move with the same velocity perpendicularly to the line segment which joins their centres of mass, along the surface of a horizontal smooth tabletop. There is a third disc of mass M and of radius R at rest, at a point on thhe perpendicular bisector of of the line segment joining the centres of mass of the two moving discs as shown in the figure. The two moving discs collide elastically with the third one, which is at rest there is no friction between the rims of the discs. What should the ratio of M/m be in order that after the collision the two discs of mass m move perpendicularly to their intial velocity?A3B2C√3D√2
Two cylinders P and Q of equal mass are made from the same metal. If the radius of P is n times the radius of Q , then calculate ratio of their resistances.
View SolutionThe ratio of the radii of gyration of a circular disc to that of a circular ring, each of same mass and radius, around their respective axes is.
View SolutionA sphere and circular disc of same mass and radius are allowed to roll down an inclined plane from the same height without slipping. Find the ratio of times taken by these two to come to the bottom of incline :
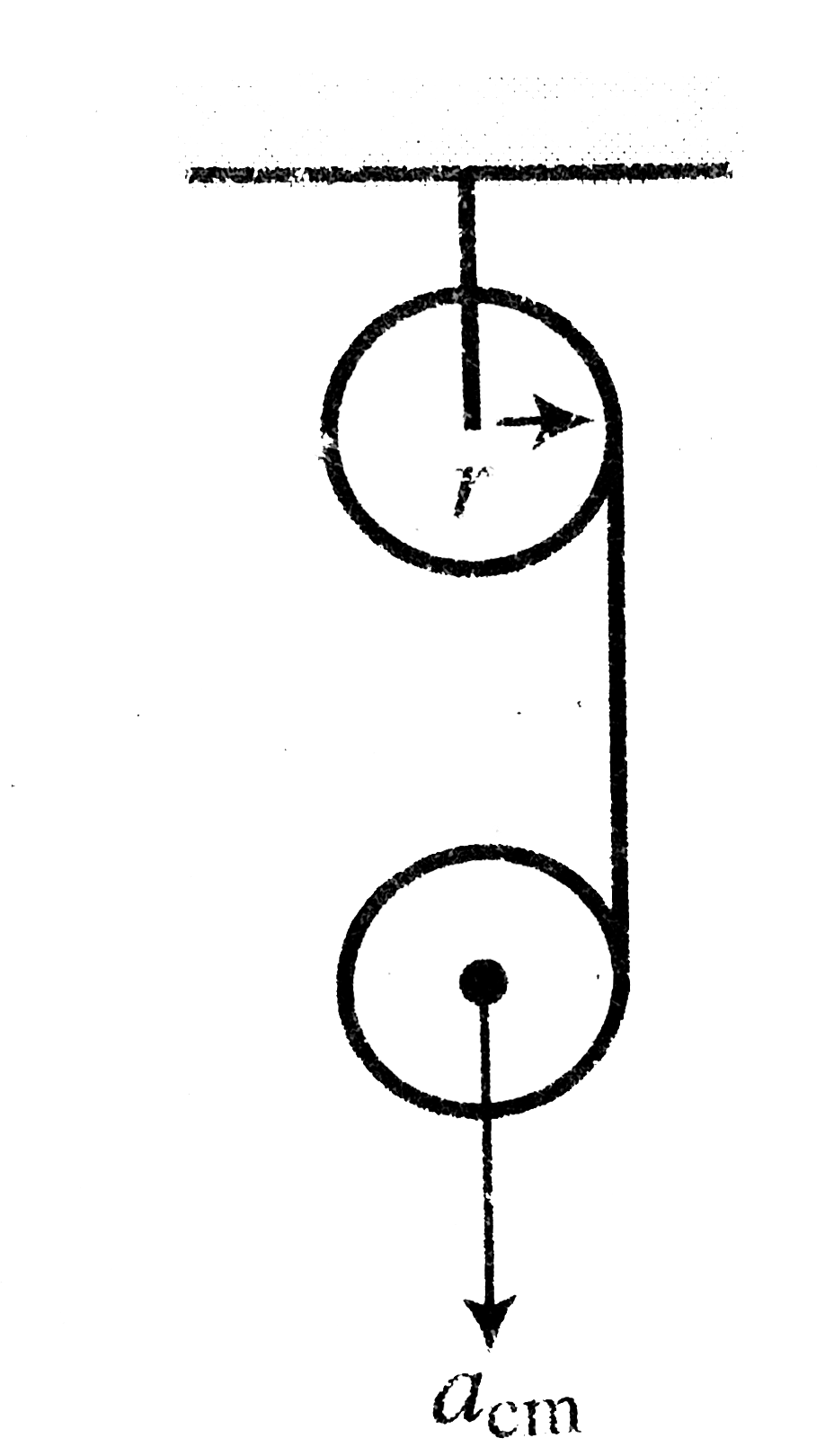
View SolutionTwo identical uniform discs of mass m and radius r are arranged as shown in the figure. If α is the angular acceleration of the lower disc and acm is acceleration of centre of mass of the lower disc, then relation among acm,α and r is
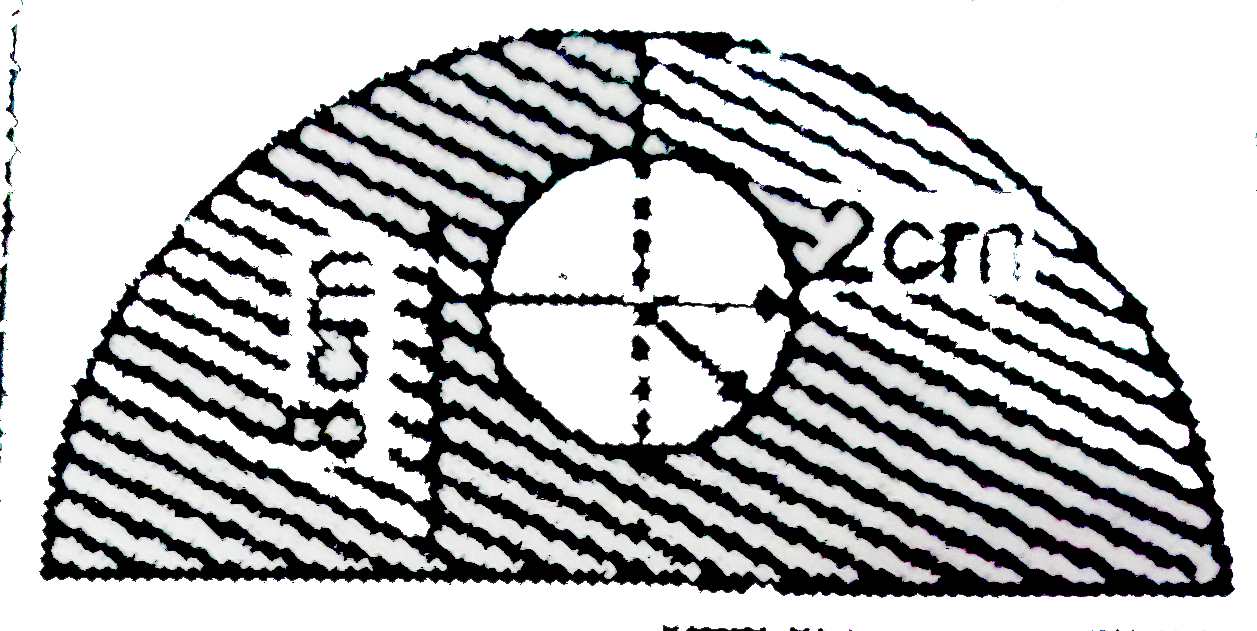
View SolutionIn the figure shown a hole of radius 2 cm is made in a semicircular disc of radius 6π at a distance 8 cm from the centre C of the disc. The distance of the centre of mass of this system from point C is :
View Solution