A particle performing SHM is found at its equilibrium position at t = 1s and it is found to have a speed 0.25 = m//s at t=2s. If the period of oscillation is 6. Calculate amplitude of oscillation.
32πm
34πm.
6πm
38πm
The correct Answer is:A
x=asin(ωt+ϕ)
at t=1s,x=0=asin(ωt+ϕ)⇒ϕ=−ω
∵v=aωcos(ωt+ϕ)
∵ At t=2s,14=aωcos(2ω+ϕ)
⇒14=a(2π6)cos(ω)=aπ3cos(π3)=aπ3(12)⇒a=32π
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-02
19 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise- 3 Match The Column
1 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
25 Videos
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
Similar Questions
A particle performing linear S.H.M. has maximum velocity of 25 cm/s and maximum acceleration of 100cm/s2. Find the amplitude and period of oscillation. (π=3.142)
View SolutionA particle in S.H.M. crosses its equilibrium position at time t = 2 sec. When the motion advances by one second more, its velocity is found to be π/3m/s. If the frequency of the motion is 1/6s10, find the amplitude of the motion in meter.
View Solution
Knowledge Check
A particle performing SHM is found at its eqilbrium at t=1sec.and it is found to have a speed of 0.25m/s at t=2sec. If the period of oscillation is 6sec. Calculate amplitude of oscillation-
A22πmB32πmC6πmD38πA particle performing S.H.M. about equilibrium position. Then the velocity of the particle is
Aslower from mean to extreme positionBfaster from mean to extreme positionCslower initial from mean position and faster lateral and stop at the endDfaster initil from mean position and later on falls off, suddenly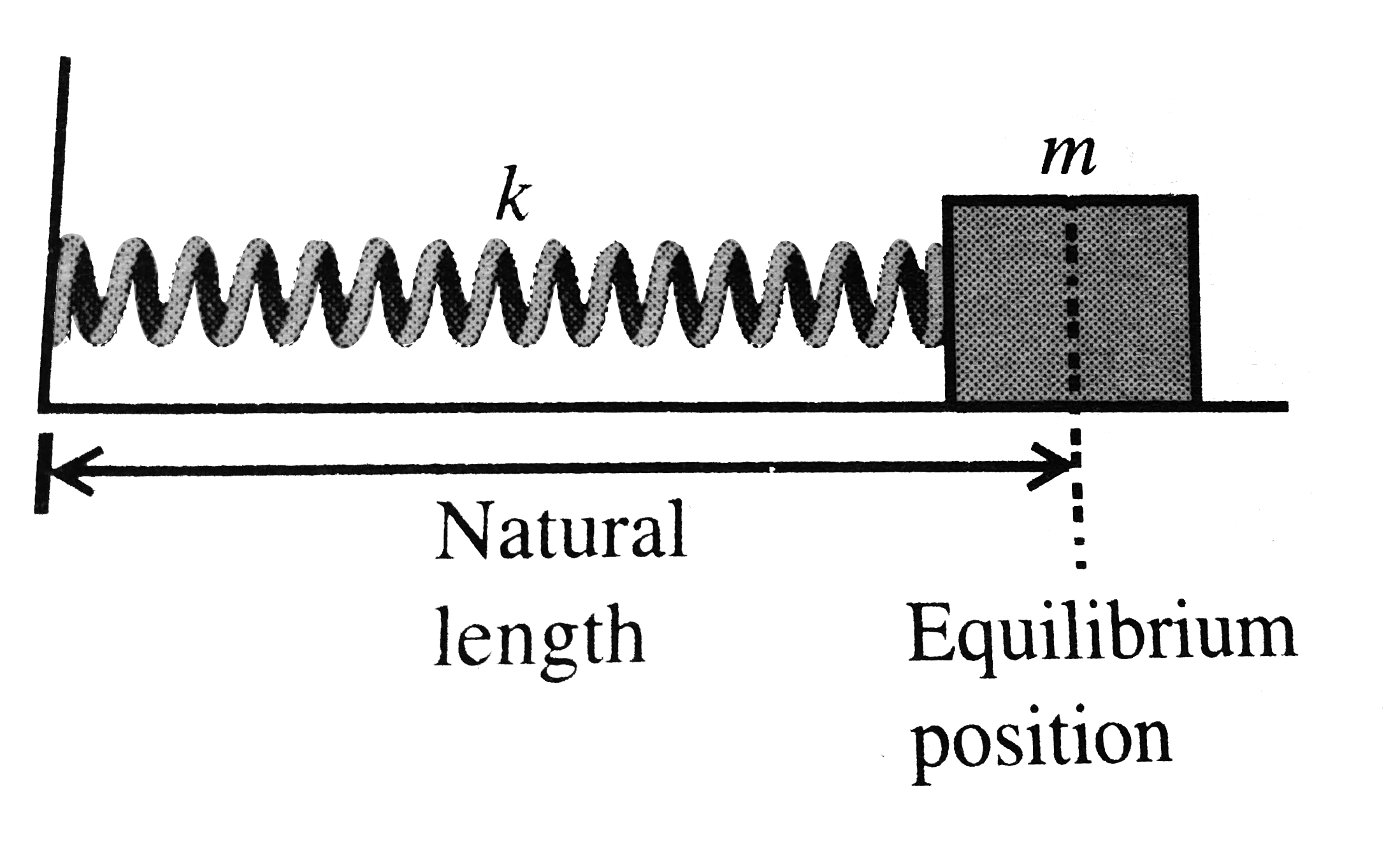
A block of mass m is connected to a spring of spring constant k as shown in Fig. The block is found at its equilibrium position t=1s and it has a velocity of +0.25ms at t=2s. The time period of oscillation is 6 s. Based on the given information answer the following question: Q. the amplitude of oscillation isA32πcmB3mC1πmD1.5m
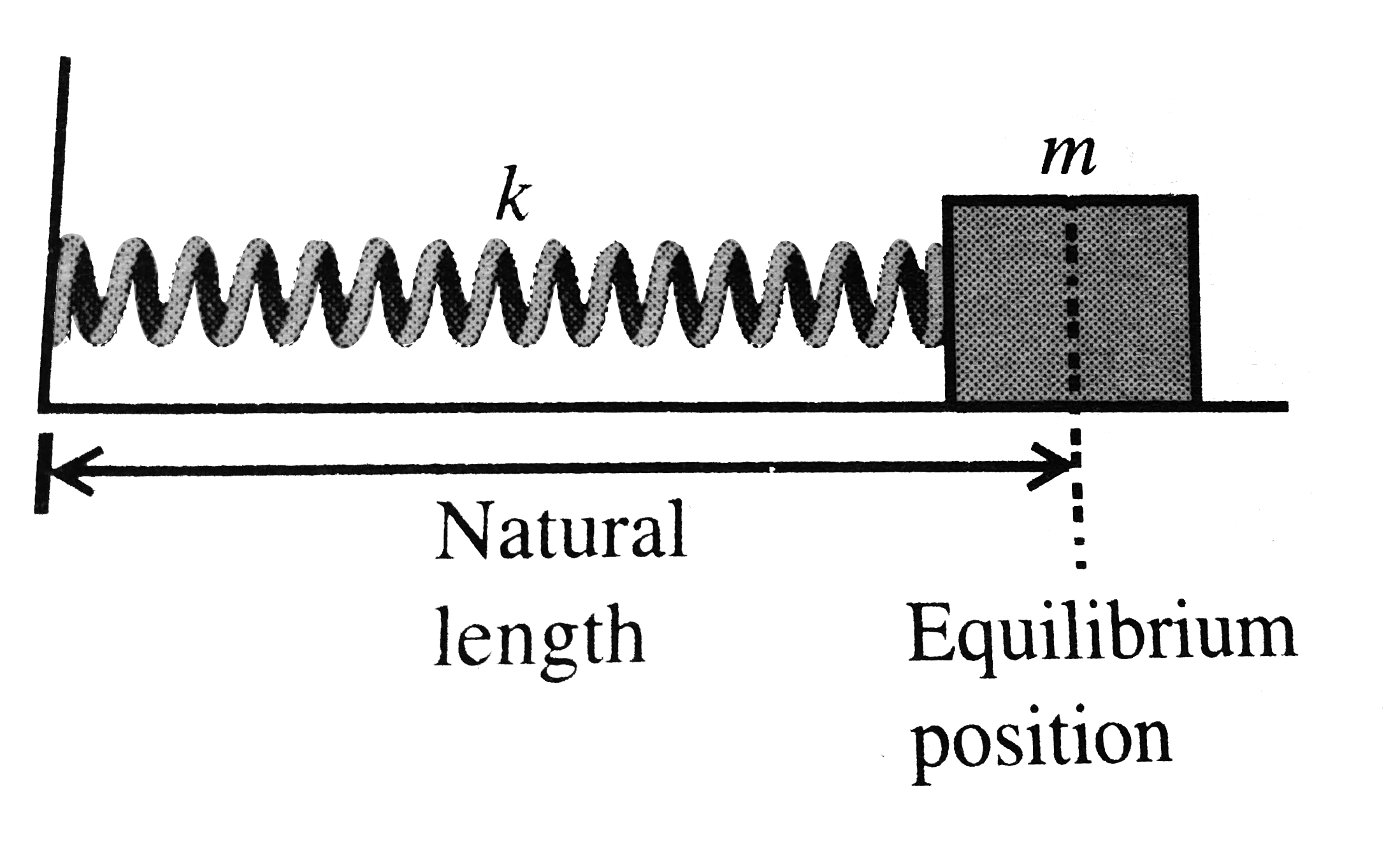
A block of mass m is connected to a spring of spring constant k as shown in Fig. The block is found at its equilibrium position t=1s and it has a velocity of +0.25ms at t=2s. The time period of oscillation is 6 s. Based on the given information answer the following question:
Q. Determine the velocity of particle at t=5s.
View SolutionA particle performing SHM starts equilibrium position and its time period is 16 seconds. After 2 seconds its velocity is πm/s. Amplitude of oscillation is
(cos45∘=1√2)
View SolutionThe time period of oscillation of a particle in S.H.M. depends
View SolutionIf a particle performing S.H.M. from the mean position of amplitude 'A' and period 'T', then the lapse of fraction of period in (7/8)th oscillation will be
View SolutionThe maimum velocity of a particle, executing SHM with an amplitude 7 mm is 4.4 m/s. the period of oscillation is
View Solution
