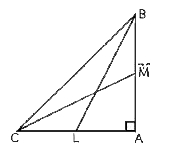
BL and CM are medians of a triangle ABC right angled at A. Prove that 4(BL2+CM2)=5BC2
Video Solution
Text Solution
Please refer to diagram in video.
As BL and CM are median to AC and AB.
So, AM=BM=12AB
and CL=AL=12CA
Now, in ΔABL,
BL2=AL2+AB2
BL2=(AC2)2+AB2=AC24+AB2
⇒4BL2=AC2+4AB2→(1)
Similarly, in ΔACM,
CM2=AM2+AC2
CM2=(AB2)2+AC2=AB24+AC2
⇒4CM2=4AC2+AB2→(2)
Adding (1) and (2),
4BL2+4CM2=AC2+4AB2+4AC2+AB2
⇒4(BL2+CM2)=5(AB2+AC2)
⇒4(BL2+CM2)=5BC2
|
Similar Questions
Knowledge Check
In a Δ right angled at B . AC = 5 cm , median AL=3√52 find the length of second median CM in cm ?
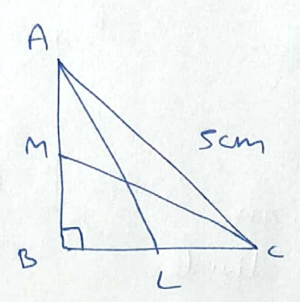 A2√5 cmB5√2 cmC10√2 cmD4√5 cm
A2√5 cmB5√2 cmC10√2 cmD4√5 cm
ABC is an isosceles right triangle right-angled at C . Prove that AB2=2AC2 .
View SolutionABC is a isosceles right angled triangle, right angled at C. prove that AB2=2AC2
View SolutionABC is an isosceles right triangle, right-angled at C . Prove that: AB2=2AC2.
View SolutionIn ΔABC,∠BAC=90∘, seg BL and seg CM are median of ΔABC. Then prove that: 4(Bl2+Cm2)=5BC2
View Solutionseg AN and seg CM are the medians of △ABC in which ∠B=90∘ Prove that 4(AN2+CM2)=5AC2
View SolutionThe points A(2,9),B(a,5),C(5,5) are the vertices of a triangle ABC right angled at B . Find the value of a and hence the area of ABC.
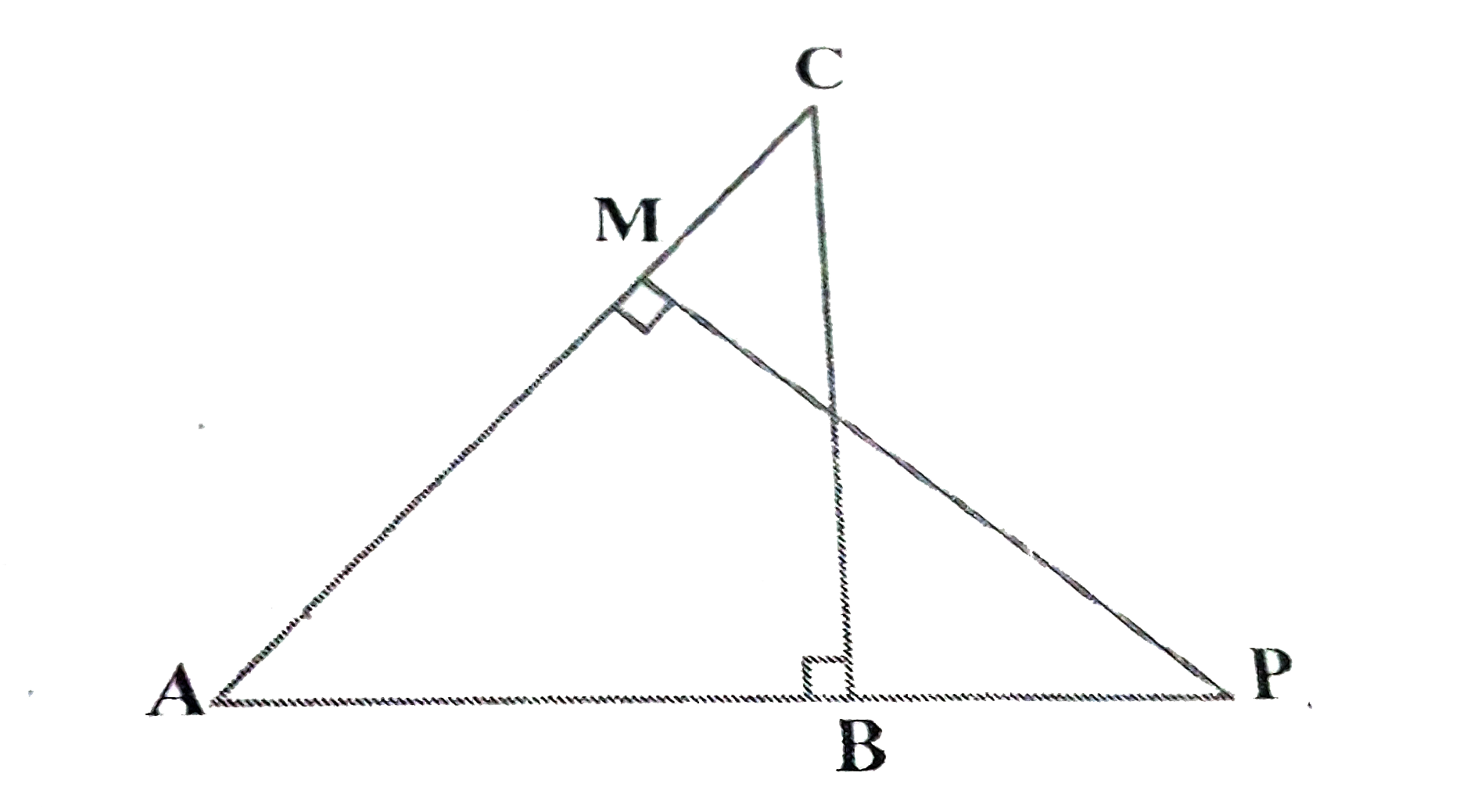
View SolutionIn figure ABC and AMP are two right triangles, right angles at B and M respectively. Prove that(i) ΔABC~ΔAMP (ii) CAPA=BCMP
View Solution