An A.P. consists of 31 terms. If its 16th terms is m, then the sum of all the terms of this A.P. is Solution in Tamil
16m
62 m
31 m
312 m
The correct Answer is:C
Step by step video, text & image solution for An A.P. consists of 31 terms. If its 16th terms is m, then the sum of all the terms of this A.P. is by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- NUMBERS AND SEQUENCESBOOK - PREMIERS PUBLISHERSCHAPTER - NUMBERS AND SEQUENCESEXERCISE - UNIT EXERCISE
12 Videos
- NUMBERS AND SEQUENCESBOOK - PREMIERS PUBLISHERSCHAPTER - NUMBERS AND SEQUENCESEXERCISE - THINKING CORNER
13 Videos
- NUMBERS AND SEQUENCESBOOK - PREMIERS PUBLISHERSCHAPTER - NUMBERS AND SEQUENCESEXERCISE - EXERCISE 2. 9
14 Videos
- MENSURATIONBOOK - PREMIERS PUBLISHERSCHAPTER - MENSURATIONEXERCISE - OTHER IMPORTANT OBJECTIVE TYPE QUESTIONS
16 Videos
- RELATIONS AND FUNCTION BOOK - PREMIERS PUBLISHERSCHAPTER - RELATIONS AND FUNCTION EXERCISE - OTHER IMPORTANT OBJECTIVE TYPE QUESTIONS
25 Videos
Similar Questions
Prove that n2−n divisible by 2 for every positive integer n.
View SolutionWhen a positive integer is divided by 3
What are the possible remainders?
View SolutionFind the sum of the following series
1+2+3+…+60
View SolutionMatch the following:
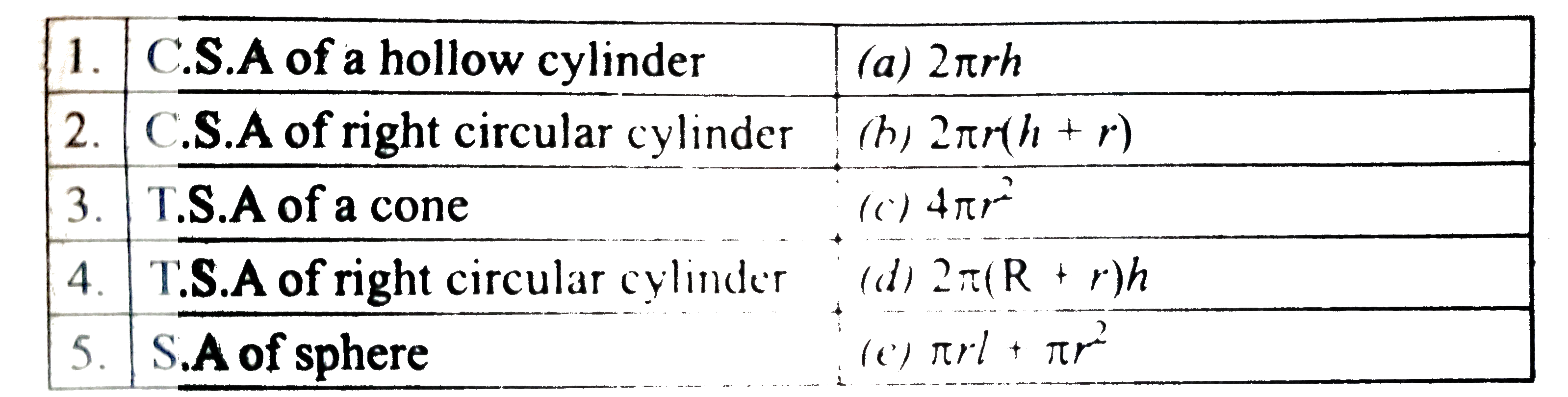
View SolutionIf n(A×B)=24andA={1,3,5,7}then n (B) is
View SolutionIf the sum of m terms of an A.P. is same as the sum of its n terms, then the sum of its (m+n) terms is
View SolutionAn A.P. consists of n terms. If the sum of its first three terms is x and the sum of the last three terms is y, then show that, the sum of all the terms of the A.P. is n6(x+y).
View SolutionAn A.P. consists of 31 terms. If its 16th terms is m, then the sum of all the terms of this A.P. is
View SolutionAn A.P. consists of 31 terms. If its 16th terms is m, then the sum of all the terms of this A.P. is
View SolutionIn an A.P, if 16th term is 47 and 31 st term is 92, then 27th term is
View SolutionIf the sum of m terms of an A.P, is equal to the sum of n terms of the A.P ., then the sum of (m + n) terms is
View SolutionAn A.P. consists of n ( odd terms ) and its middle term is m. Then the sum of the A.P. is :
View SolutionIf 11th term of A.P. is 38 and 16th term is 73, then its first term is :
View SolutionIn an A.P. of 99 terms, the sum of all the odd-numbered terms is 2550. Then find the sum of all the 99 terms of the A.P.
View SolutionShow that the sequence 9,12,15,18,... is an A.P. Find its 16th term and the general term.
View SolutionIf the sum of m terms of an A.P. is same as the sum of its n terms, then the sum of its (m+n) terms is
View Solution
