Three identical cells, each of emf 2 V and internal resistance are connected in series to an external resistor of . Calculate the current in the circuit.
To solve the problem, we need to calculate the current flowing in a circuit consisting of three identical cells connected in series with an external resistor. Here’s a step-by-step breakdown of the solution:
Step 1: Identify the components of the circuit
- Each cell has an EMF (Electromotive Force) of 2 V and an internal resistance of 0.2 Ω.
- There are three cells connected in series.
- The external resistor has a resistance of 7.4 Ω.
Step 2: Calculate the total EMF of the cells
Since the cells are connected in series, the total EMF (V_total) is the sum of the individual EMFs:
Vtotal=EMF1+EMF2+EMF3=2V+2V+2V=6V
Step 3: Calculate the total internal resistance
The internal resistances of the cells also add up when connected in series. Therefore, the total internal resistance (R_internal) is:
Rinternal=r1+r2+r3=0.2Ω+0.2Ω+0.2Ω=0.6Ω
Step 4: Calculate the total resistance in the circuit
The total resistance in the circuit (R_total) is the sum of the total internal resistance and the external resistance:
Rtotal=Rinternal+Rexternal=0.6Ω+7.4Ω=8.0Ω
Step 5: Apply Ohm's Law to find the current
Using Ohm's Law, which states that V=I×R, we can rearrange it to find the current (I):
I=VtotalRtotal=6V8.0Ω=0.75A
Final Answer
The current flowing in the circuit is 0.75A.
---
- Each cell has an EMF (Electromotive Force) of 2 V and an internal resistance of 0.2 Ω.
- There are three cells connected in series.
- The external resistor has a resistance of 7.4 Ω.
Since the cells are connected in series, the total EMF (V_total) is the sum of the individual EMFs:
Vtotal=EMF1+EMF2+EMF3=2V+2V+2V=6V
The internal resistances of the cells also add up when connected in series. Therefore, the total internal resistance (R_internal) is:
Rinternal=r1+r2+r3=0.2Ω+0.2Ω+0.2Ω=0.6Ω
The total resistance in the circuit (R_total) is the sum of the total internal resistance and the external resistance:
Rtotal=Rinternal+Rexternal=0.6Ω+7.4Ω=8.0Ω
Using Ohm's Law, which states that V=I×R, we can rearrange it to find the current (I):
I=VtotalRtotal=6V8.0Ω=0.75A
The current flowing in the circuit is 0.75A.
Similar Questions
Three identical, each of emf 2 V and internal resistance 0.2Ω are connected in series to an external resistor of 7.4Ω. Calculate the current in the circuit.
View SolutionThree identical cells, each of emf 2V and internal resistance 0.2 ohm, are connected in series to an external resistor of 7.4 ohm. Calculate the current in the circuit and the terminal potential difference across an equivalent.
View Solution
Knowledge Check
Four cells, each of emf E and internal resistance r, are connected in series across an external resistance reverse. Then, the current in the external circuit is
A2E4r+RB3E4r+RC3E3r+RD2E3r+R4 cells each of emf 2 V and internal resistance of 1Ω are connected in parallel to a load resistor of 2Ω. Then the current through the load resistor is
A2AB1.5AC1AD0.888AThree identical cells , each having an emf1.5V and a constant internal resistance 2.0ω, are connected in series with a 4.0ωresis→rR, first as in circuit (i) , and second as in circuit (ii) . Then
( power in R in circuit (i))/( Power in R in circuit (ii)) =
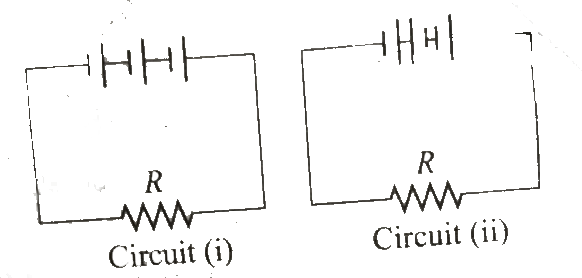 A9.0B7.2C1.8D3.0
A9.0B7.2C1.8D3.0
Three identical cells of emf 4V with 1Ω internal resistance each are connected in series to an external resistance of 6Ω. Find the current flowing in the circuit.
View SolutionThree identical cells of emf 2 V and internal resistances 0.20Ω are connected in series. The combination is further connected to an external resistor of 6Ω . Calculate the current through 6Ω resistor.
View SolutionA set of 4 cell each of emf 2V and internal resistance 1.5Ω are connected across an external load of 10Ω with 2 rows, two cells in eacg row. Calculate the current in each row and potential difference across 10Ω
View SolutionThree similar cells, each of emf 2 V and internal resistance rΩ send the same current through an external resistance of 2Ω, when connected in series or in parallel. The strength of the current flowing through the external resistance is
View SolutionA cell of e.m.f. 2 V and internal resistance 2Ω and an external resistance 18Ω forms closed circuit. The current through the circuit is
View Solution