If a tangential force mg is applied to a disc of mass m and radius r, the angular acceleration produced in it is?
gr
g/r
2gr
2gr
The correct Answer is:C
To find the angular acceleration produced in a disc of mass m and radius r when a tangential force mg is applied, we can follow these steps:
Step 1: Identify the Forces and Torque
We have a disc with a mass m and radius r. A tangential force F=mg is applied at the edge of the disc. The torque τ produced by this force about the center of the disc can be calculated using the formula:
τ=r×F
Substituting the value of F:
τ=r×mg
Step 2: Relate Torque to Angular Acceleration
According to the rotational dynamics, the torque is also related to the moment of inertia I and angular acceleration α by the equation:
τ=Iα
Step 3: Calculate the Moment of Inertia
For a solid disc rotating about its center, the moment of inertia I is given by:
I=12mr2
Step 4: Set Up the Equation
Now we can set the two expressions for torque equal to each other:
r⋅mg=Iα
Substituting the moment of inertia:
r⋅mg=(12mr2)α
Step 5: Solve for Angular Acceleration
We can now solve for α:
1. Rearranging the equation gives:
α=r⋅mg12mr2
2. Simplifying this expression:
α=2gr
Final Answer
Thus, the angular acceleration produced in the disc is:
α=2gr
---
We have a disc with a mass m and radius r. A tangential force F=mg is applied at the edge of the disc. The torque τ produced by this force about the center of the disc can be calculated using the formula:
τ=r×F
Substituting the value of F:
τ=r×mg
According to the rotational dynamics, the torque is also related to the moment of inertia I and angular acceleration α by the equation:
τ=Iα
For a solid disc rotating about its center, the moment of inertia I is given by:
I=12mr2
Now we can set the two expressions for torque equal to each other:
r⋅mg=Iα
Substituting the moment of inertia:
r⋅mg=(12mr2)α
We can now solve for α:
1. Rearranging the equation gives:
α=r⋅mg12mr2
2. Simplifying this expression:
α=2gr
Thus, the angular acceleration produced in the disc is:
α=2gr
Similar Questions

A tangential force F acts at the top of a thin spherical shell of mass m and radius R. Find the acceleration of the shell if it rolls without slipping.
View SolutionA uniform disc of mass M and radius R is hinged at its centre C . A force F is applied on the disc as shown . At this instant , angular acceleration of the disc is

View Solution
Knowledge Check
A uniform disc of mass M and radius R is hinged at its centre C. A force F is applied on the disc as shown. At this instant, the angular acceleration of the disc is
 A√3FMRBFMRC2√3FMRDF2MR
A√3FMRBFMRC2√3FMRDF2MRA tangential force F is applied on a disc of radius R, due to which it deflects through an angle θ from its initial position. The work done by this force would be
AFRBFθCFRθDFRθA disc has mass 'M" and radius 'R'. How much tangential force should be applied to the rim of the disc so as to rotate with angular velocity ω in time 't'?
AMrω4tBMRω2tCMRωtDMRωt
A man of mass m stands on a horizontal platform in the shape of a disc of mass m and radius R, pivoted on a vertical axis through its centre about which it can freely rotate. The man starts to move around the centre of the disc in a circle of radius r with a velocity v relative to the disc. Calculate the angular velocity of the disc.
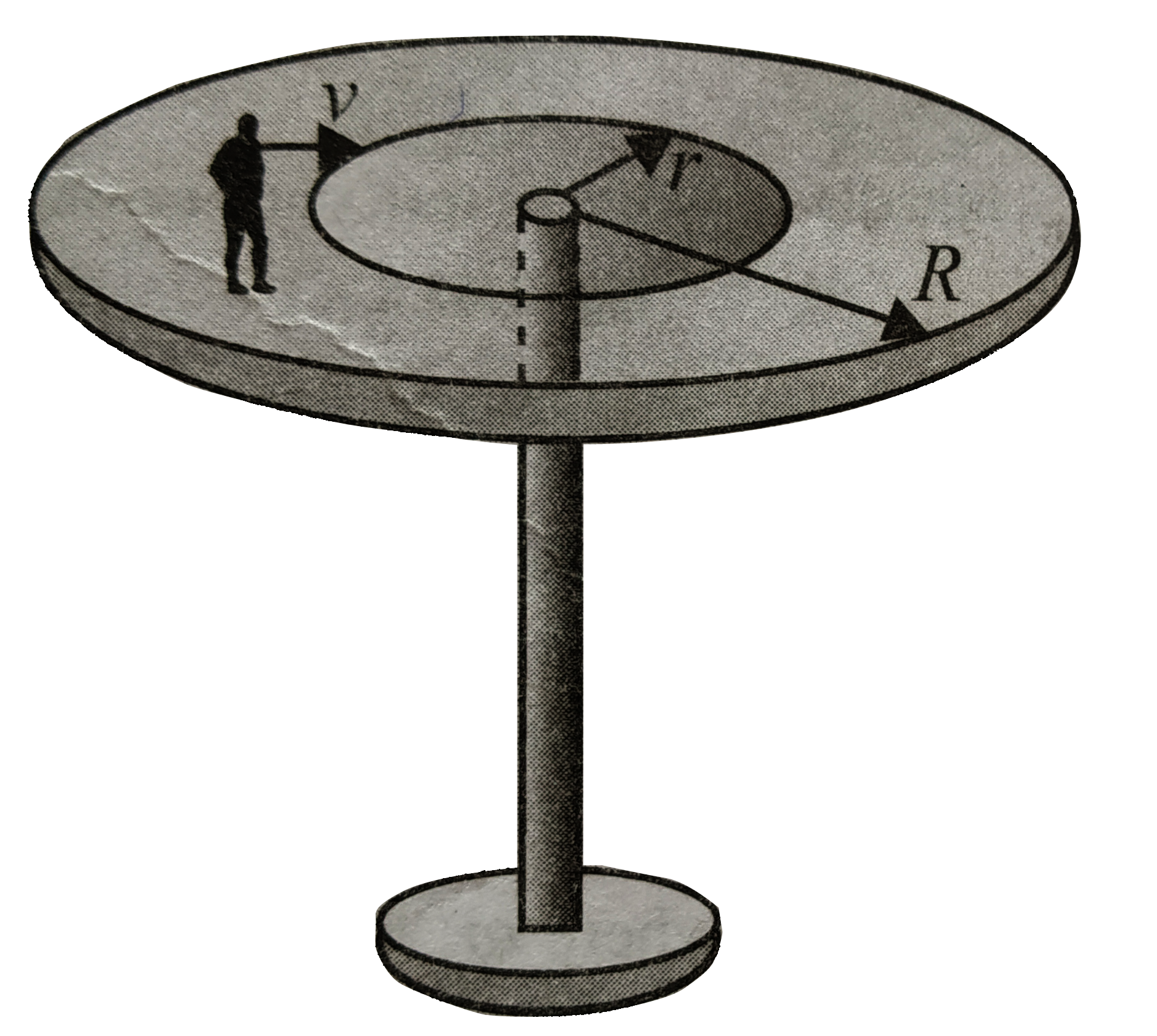
View Solution
In the figure shown, a force F is applied at the top of a disc of mass 4 kg and radius 0.25 m. find maximum velue of F for no slipping.
View SolutionTwo equal and opposite forces are allplied tangentially to a uniform disc of mass M and radius R as shown in the figure. If the disc is pivoted at its centre and free to rotate in its plane, the angular acceleration of the disc is :

View SolutionA disc has mass 'M' and radius 'R'. How much tangential force should be applied to the rim of the disc, so as to rotate with angular velocity 'ω' in time 't' ?
View SolutionA disc has mass 'M' and radius 'R'. How much tangential force should be applied to the rim of the disc, so as to rotate with angular velocity 'ω' in time 't' ?
View Solution
