A ball is thrown from the top of a 35m high tower with initial velocity of magnitude u=80 ms−1 at an angle 25∘ with horizontal.
Find the time to reach the ground and the horizontal distance covered by the ball.
To solve the problem step by step, we will break it down into parts: calculating the time taken for the ball to reach the ground and then calculating the horizontal distance covered by the ball.
Step 1: Determine the initial velocity components
The initial velocity u is given as 80m/s at an angle of 25∘ with the horizontal.
1. Horizontal component ux:
ux=u⋅cos(25∘)=80⋅cos(25∘)
Using cos(25∘)≈0.9063:
ux=80⋅0.9063≈72.5m/s
2. Vertical component uy:
uy=u⋅sin(25∘)=80⋅sin(25∘)
Using sin(25∘)≈0.4226:
uy=80⋅0.4226≈33.8m/s
Step 2: Set up the equation for vertical motion
We will use the following equation of motion to find the time t taken to reach the ground:
s=ut+12at2
Where:
- s=−35m (the displacement is negative because the ball is falling down)
- u=uy=33.8m/s
- a=−g=−9.8m/s2 (acceleration due to gravity)
Substituting these values into the equation:
−35=33.8t−12(9.8)t2
Rearranging gives:
12(9.8)t2−33.8t−35=0
This simplifies to:
4.9t2−33.8t−35=0
Step 3: Solve the quadratic equation
Using the quadratic formula t=−b±√b2−4ac2a:
- Here, a=4.9, b=−33.8, and c=−35.
Calculating the discriminant:
D=b2−4ac=(−33.8)2−4⋅4.9⋅(−35)
D=1142.44+686=1828.44
Now, substituting into the quadratic formula:
t=33.8±√1828.442⋅4.9
Calculating √1828.44≈42.7:
t=33.8±42.79.8
Calculating the two possible values for t:
1. t=33.8+42.79.8≈7.8s
2. t=33.8−42.79.8 (this will yield a negative time, which is not physically meaningful)
Thus, the time taken to reach the ground is approximately:
t≈7.8s
Step 4: Calculate the horizontal distance covered
The horizontal distance R can be calculated using the formula:
R=ux⋅t
Substituting the values:
R=72.5⋅7.8≈566.55m
Final Results
- Time to reach the ground: t≈7.8s
- Horizontal distance covered: R≈566.55m
---
ux=u⋅cos(25∘)=80⋅cos(25∘)
Using cos(25∘)≈0.9063:
ux=80⋅0.9063≈72.5m/s
uy=u⋅sin(25∘)=80⋅sin(25∘)
Using sin(25∘)≈0.4226:
uy=80⋅0.4226≈33.8m/s
s=ut+12at2
Where:
- s=−35m (the displacement is negative because the ball is falling down)
- u=uy=33.8m/s
- a=−g=−9.8m/s2 (acceleration due to gravity)
−35=33.8t−12(9.8)t2
Rearranging gives:
12(9.8)t2−33.8t−35=0
This simplifies to:
4.9t2−33.8t−35=0
- Here, a=4.9, b=−33.8, and c=−35.
D=b2−4ac=(−33.8)2−4⋅4.9⋅(−35)
D=1142.44+686=1828.44
t=33.8±√1828.442⋅4.9
Calculating √1828.44≈42.7:
t=33.8±42.79.8
1. t=33.8+42.79.8≈7.8s
2. t=33.8−42.79.8 (this will yield a negative time, which is not physically meaningful)
t≈7.8s
R=ux⋅t
Substituting the values:
R=72.5⋅7.8≈566.55m
- Time to reach the ground: t≈7.8s
- Horizontal distance covered: R≈566.55m
Similar Questions
A ball is thrown from the top of a tower with an initial velocity of 10 m//s at an angle of 30∘above the horizontal. It hits the ground at a distance of 17.3 m from the base of the tower. The height of the tower (g=10m/s2) will be
View SolutionA ball is thrown from the top of a tower with an intial velocity of 10 m//s at an angle 37∘ above the horizontal, hits the ground at a distance 16 m from the base of tower. Calculate height of tower. [g=10m/s2]
View Solution
Knowledge Check
A ball is thrown from the top of tower with an initial velocity of 10ms−1 at an angle of 30∘ with the horizontal. If it hits the ground of a distance of 17.3m from the back of the tower, the height of the tower is (Takeg=10ms−2)
A5mB20mC15mD10mA ball is thrown from the top of 36m high tower with velocity 5m/ss at an angle 37∘ above the horizontal as shown. Its horizontal distance on the ground is closest to [g=10m/s2
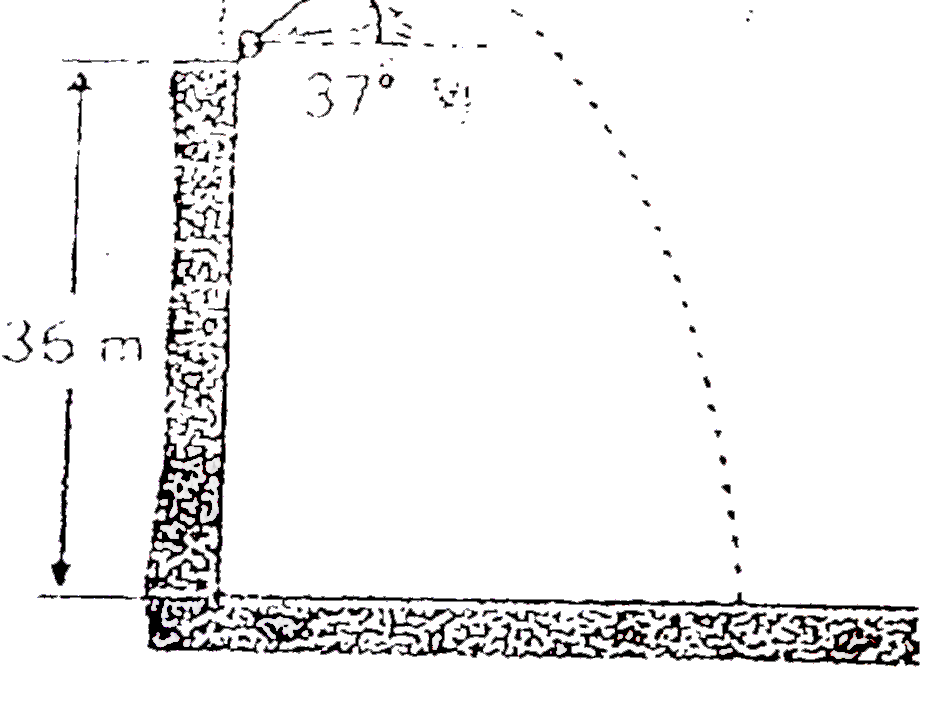 : A12mB18mC24mD30m
: A12mB18mC24mD30mA ball is projected horizontal from the top of a tower with a velocity v0. It will be moving at an angle of 60∘ with the horizontal after time.
Av0√3gB√3v0gCv0gDv0g
A ball is thrown from the top of a tower of 61m high with a velocity 24.4ms−1 at an elevation of 30∘ above the horizontal. What is the distance from the foot of the tower to the point where the ball hits the ground?
View SolutionA ball is thrown from the top of a building 45m high with a speed 20ms−1 above the horizontal at an angle of 30∘. Find
(a) The time taken by the ball to reach the ground.
(b) The speed of ball just before it touches the ground.
View SolutionA ball is thrown from the ground with velocity u at an angle θ with horizontal. If the coefficient of restitution is e , find the time of flight , the maximum height and the horizontal range after the first collision.
View SolutionA ball is projected upwards from the top of a tower with a velocity 50ms−1 making an angle 30∘ with the horizontal. The height of tower is 70m. After how many seconds from the instant of throwing, will the ball reach the ground. (g=10ms−2)
View SolutionA ball is thrown from the ground with a velocity of 20√3 m/s making an angle of 60∘ with the horizontal. The ball will be at a height of 40 m from the ground after a time t equal to (g=10ms−2)
View Solution

