A layer of benzene (μ=1.5) 12 cm thick floats on water layer (μ=4/3) 8 cm thick in a vessel. When viewed from the top, the apparent depth of bottom of vessel below the surface of benzene will be
20 cm
14 cm
7 cm
21 cm
The correct Answer is:B
To find the apparent depth of the bottom of the vessel below the surface of benzene, we need to consider the refractive indices and the actual depths of the layers of benzene and water.
1. Identify the Given Data:
- Refractive index of benzene, μbenzene=1.5
- Thickness of benzene, dbenzene=12cm
- Refractive index of water, μwater=43
- Thickness of water, dwater=8cm
2. Calculate the Apparent Depth of Benzene:
The formula for apparent depth when viewing through a medium is given by:
Apparent Depth=Actual DepthRefractive Index
For benzene:
Apparent Depthbenzene=dbenzeneμbenzene=12cm1.5=8cm
3. Calculate the Apparent Depth of Water:
Using the same formula for the water layer:
Apparent Depthwater=dwaterμwater=8cm43=8cm×34=6cm
4. Calculate the Total Apparent Depth:
The total apparent depth from the top of the benzene to the bottom of the vessel is the sum of the apparent depths of both layers:
Total Apparent Depth=Apparent Depthbenzene+Apparent Depthwater=8cm+6cm=14cm
5. Conclusion:
Thus, the apparent depth of the bottom of the vessel below the surface of benzene is 14cm.
Final Answer:
The apparent depth of the bottom of the vessel below the surface of benzene is 14cm.
---
- Refractive index of benzene, μbenzene=1.5
- Thickness of benzene, dbenzene=12cm
- Refractive index of water, μwater=43
- Thickness of water, dwater=8cm
The formula for apparent depth when viewing through a medium is given by:
Apparent Depth=Actual DepthRefractive Index
For benzene:
Apparent Depthbenzene=dbenzeneμbenzene=12cm1.5=8cm
Using the same formula for the water layer:
Apparent Depthwater=dwaterμwater=8cm43=8cm×34=6cm
The total apparent depth from the top of the benzene to the bottom of the vessel is the sum of the apparent depths of both layers:
Total Apparent Depth=Apparent Depthbenzene+Apparent Depthwater=8cm+6cm=14cm
Thus, the apparent depth of the bottom of the vessel below the surface of benzene is 14cm.
The apparent depth of the bottom of the vessel below the surface of benzene is 14cm.
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - AAKASH INSTITUTECHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - ASSIGNMENT (SECTION - D)
16 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - AAKASH INSTITUTECHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - ASSIGNMENT (SECTION - B)
30 Videos
- PHYSICAL WORLDBOOK - AAKASH INSTITUTECHAPTER - PHYSICAL WORLDEXERCISE - ASSIGNMENT (Section-B)
5 Videos
- SEMICONDUCTOR ELECTRONICS (MATERIAL, DEVICES AND SIMPLE CIRUITS )BOOK - AAKASH INSTITUTECHAPTER - SEMICONDUCTOR ELECTRONICS (MATERIAL, DEVICES AND SIMPLE CIRUITS )EXERCISE - Assignment SECTION - D (Assertion & reason type Question)
10 Videos
Similar Questions
An object lies 100 cm inside water (μ=4/3). It is viewed from air nearly normally. Find the apparent depth of the object.
View SolutionA layer of oil 3 cm thick is floating on a layer of coloured water 5 cm thick. Refractive index of coloured water is 5/3 and the apparent depth of the two liquids appears to be 36/7cm. Find the refractive index of oil.
View Solution
Knowledge Check
A mark is made on the bottom of a vessel and over this mark, a glass slab to thickness 3.5cm and refractive index 74 is placed. Now water (refractive index, 43) is poured into the vessel so that the surface of water is 8cm above the upper surface of the slab. Looking down of normally through the water, the apparent depth of the mark below the surface of water will be :
A6.33cmB7.5cmC8cmD10cmA glass sphere (μ =1.5) of radius 20 cm has small air bubble 4 cm below its centre. The sphere is viewed from outside and along vertical line through the bubble. The apparent depth of the bubble below the surface of sphere is (in cm)
A13.33B26.67C15D30A layer of oil 3 cm thick is flowing on a layer of coloured wtater 5 cm thick. Refractive index of coloured water is 53 and the apparent depth of the two liquids appears to be 367 cm . What is the refractive index of oil?
A1.4B2C2.4D3
A 20 mm thick layer of water (n=1.33) floats on a 40 mm thick layer of carbon tetrachloride (n=1.46) in a tank. A coin lies at the bottom of the tank. At what depth below the top water surface do you perceive the coin ?
View SolutionA glass sphere(μ=1.5) with a radius of 15.9 cm has a tiny air bubble 5 cm its centre.This sphere is viewed looking down along the extended radius containing the bubble.What is the apparent depth of the bubble below the sirface of the sphere ?
8.57 cm from top
View SolutionChoose the most appropriate option.
A 4cm thick layer of water covers a 6 cm thick glass slab. A coin is placed at the bottom of the slab and is being observed from the air side along the normal to the surface. Find the apparent position of the coin from
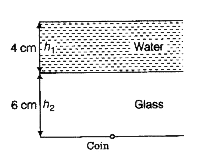
View SolutionA glass beaker is filled with water up to 5cm. It is kept on top of a 2cm thick glass slab. When a coin at the bottom of the glass slab is viewed at the normal incidence from above the beaker, its apparent depth from the water surface is dcm. Value of d is close (the refractive index of water and glass are 1.33 and 1.50,respectively)
View SolutionA rectangular tank of depth 8 m is full of water (μ=4/3). The bottom is seen at the depth
View Solution
