A: There may be an induced emf in a loop without induced current.
R: Induced current depends on the resistance of the loop as well.
If both Assertion & Reason are true and the reason is the correct explanation of the assertion.
If both Assertion & Reason are true but the reason is not the correct explanation of the assertion.
If Assertion is true statement but Reason is false.
If both Assertion and Reason are false statements.
The correct Answer is:B
To solve the problem, we need to analyze the two statements given:
1. Statement A: "There may be an induced emf in a loop without induced current."
2. Statement R: "Induced current depends on the resistance of the loop as well."
Step 1: Analyze Statement A
- We know that induced emf (electromotive force) can be generated due to a change in magnetic flux through a loop. This is described by Faraday's law of electromagnetic induction.
- However, for a current to flow, the loop must be closed. If the loop is not closed, an emf can still be induced, but no current will flow.
- Therefore, Statement A is True. An induced emf can exist in an open loop without any induced current.
Step 2: Analyze Statement R
- The induced current in a closed loop is given by Ohm's law, which states that current (I) is equal to the induced emf (E) divided by the resistance (R) of the loop:
I=ER
- This shows that the induced current does indeed depend on the resistance of the loop. If the resistance is high, the current will be low for a given emf, and vice versa.
- Therefore, Statement R is also True. The induced current depends on the resistance of the loop.
Step 3: Check if R is a correct explanation of A
- Statement R explains that the induced current depends on the resistance of the loop. Since Statement A is about the existence of induced emf without current, and Statement R discusses the conditions under which current can flow, we see that both statements are related.
- The existence of induced emf without current (Statement A) is indeed explained by the fact that if the loop is open (high resistance), no current will flow despite the presence of induced emf (Statement R).
- Therefore, Statement R correctly explains Statement A.
Conclusion:
Both statements A and R are true, and R is a correct explanation of A.
The final answer is that both statements are correct, and R is the correct explanation of A.
---
2. Statement R: "Induced current depends on the resistance of the loop as well."
- We know that induced emf (electromotive force) can be generated due to a change in magnetic flux through a loop. This is described by Faraday's law of electromagnetic induction.
- However, for a current to flow, the loop must be closed. If the loop is not closed, an emf can still be induced, but no current will flow.
- Therefore, Statement A is True. An induced emf can exist in an open loop without any induced current.
- The induced current in a closed loop is given by Ohm's law, which states that current (I) is equal to the induced emf (E) divided by the resistance (R) of the loop:
I=ER
- This shows that the induced current does indeed depend on the resistance of the loop. If the resistance is high, the current will be low for a given emf, and vice versa.
- Therefore, Statement R is also True. The induced current depends on the resistance of the loop.
- Statement R explains that the induced current depends on the resistance of the loop. Since Statement A is about the existence of induced emf without current, and Statement R discusses the conditions under which current can flow, we see that both statements are related.
- The existence of induced emf without current (Statement A) is indeed explained by the fact that if the loop is open (high resistance), no current will flow despite the presence of induced emf (Statement R).
- Therefore, Statement R correctly explains Statement A.
Both statements A and R are true, and R is a correct explanation of A.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBook:AAKASH INSTITUTEChapter:ELECTROMAGNETIC INDUCTIONExercise:ASSIGNMENT(SECTION -C) Previous Years Questions
Explore 23 Videos
- ELECTRIC CHARGES AND FIELDS Book:AAKASH INSTITUTEChapter:ELECTRIC CHARGES AND FIELDS Exercise:SECTION -J(Aakash Challengers Questions)
Explore 5 Videos
- ELECTROMAGNETIC WAVESBook:AAKASH INSTITUTEChapter:ELECTROMAGNETIC WAVESExercise:ASSIGNMENT SECTION - D Assertion-Reason Type Questions
Explore 25 Videos
Similar Questions
Statement-1 : The induced e.m.f. and current will be same in two identical loops of copper and aluminium, when rotated with same speed in the same magnetic field.
Statement-2 : Induced e.m.f. is proportional to rate of change of magnetic field while induced current depends on resistance of wire.
View SolutionThe south pole of a magnet is brought near a conducting loop. What is the direction of induced current as seen by a person on the other side of the loop ?
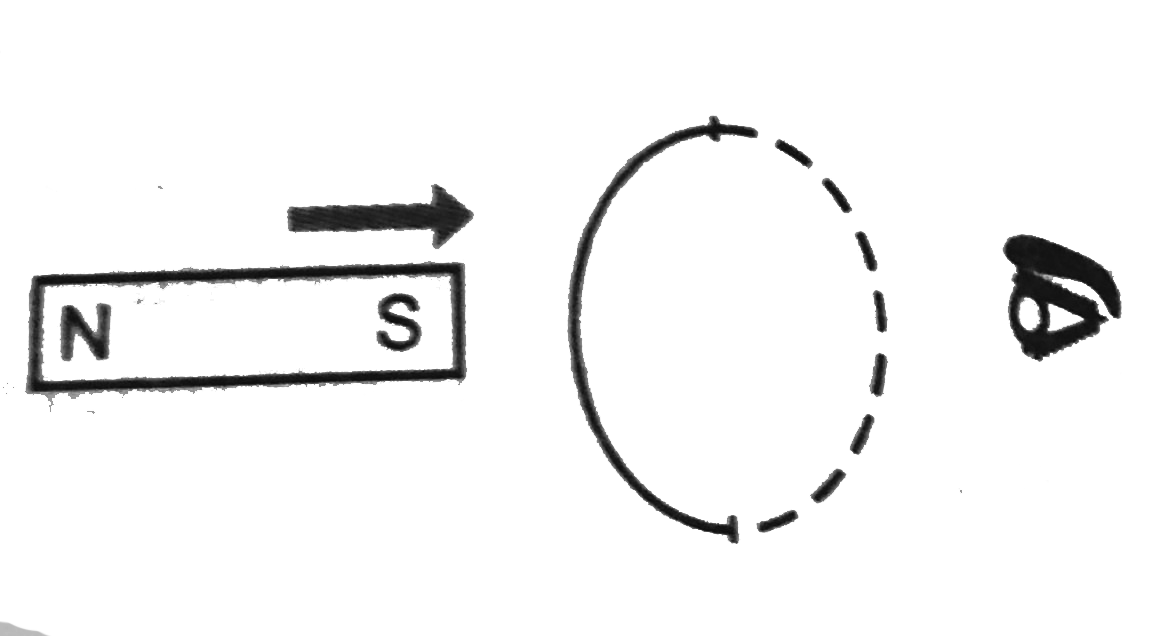
View Solution
Knowledge Check
The strength of the induced current depends on
Athe number of turns in the coilBcross-sectional area of the coilCstrength of the magnetic filedDspeed of rotation of the coilAssertion : There can be induced e.m.f. in an inductor even if the current through it is zero.
Reason : Induced e.m.f. depends upon the rate of change of current rather than the current itself.AAssertion is true and Reason is correct explanation of AssertionBAssertion is true and Reason is falseCAssertion and Reason both are falseDAssertion is false but Reason is trueInduced emf in the coil depends upon :
Aconductivity of coilBamount of fluxCrate of change of linked fluxDresistance of coil
What is the direction of induced current in resistance 'R' ?
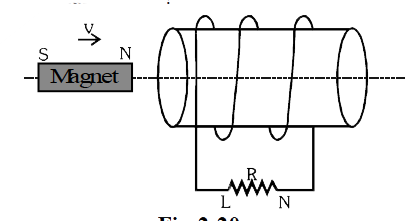
View SolutionInduced emf in the coil depends upon :
View SolutionInduced emf in the coil depends upon
View SolutionAsseration:The induced e.m.f. and curent will be same in two identical loops of copper and aluminium, when rotated with same speed in the same magnetic field.
Reason:Induced e.m.f. is proportional to rate of change of magnetic field while induced current depends on resistance of wire.
View SolutionThe direction of induced current in the loop
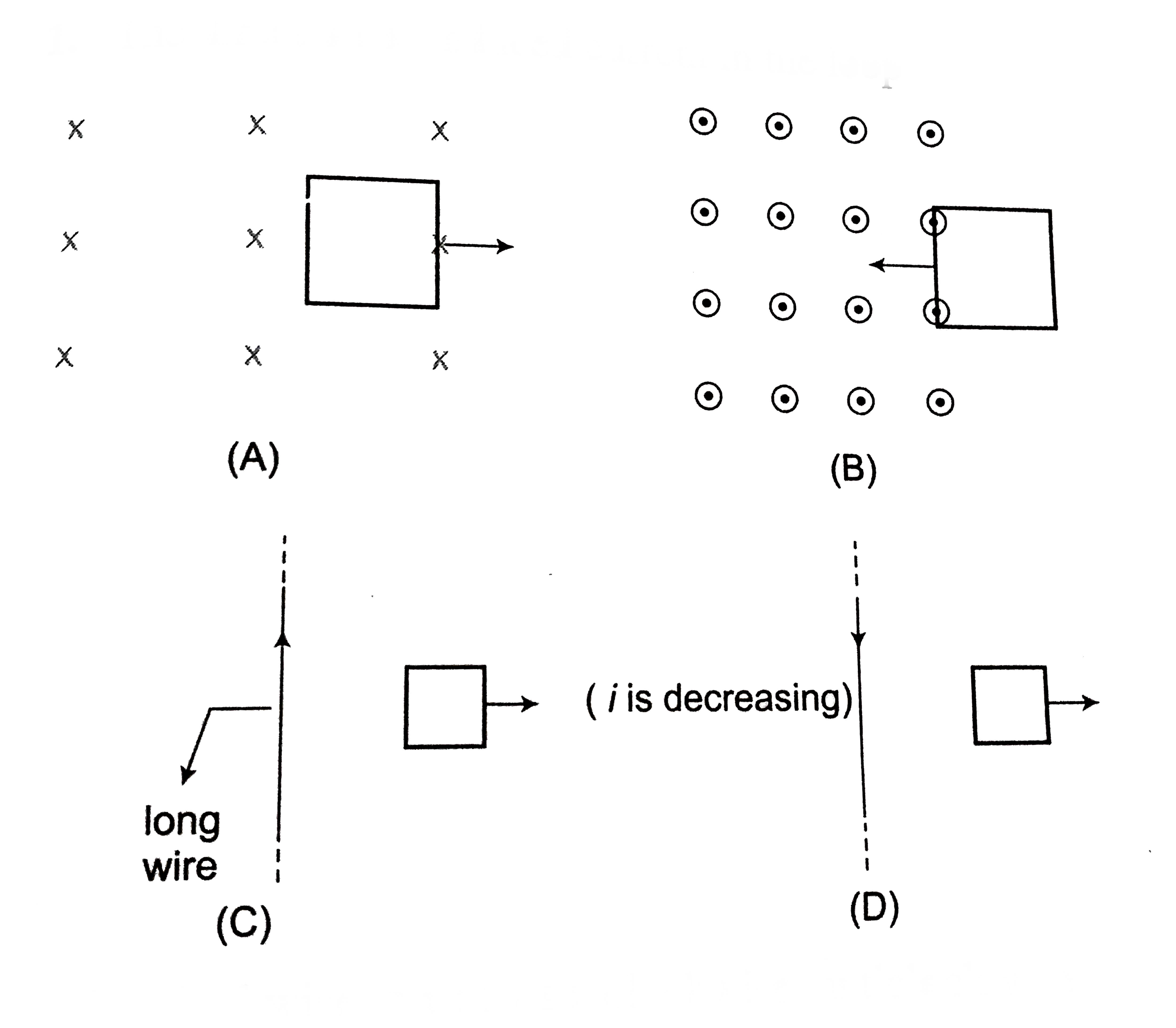
View Solution