An aluminium cylinder 10 cm long, with a cross-sectional area of 20cm2, is to be used as a spacer between two steel walls. At 17.2∘C it just slips in between the walls. When it warms to 22.3∘C, calculate the stress in the cylinder and the total force if exerts on each wall, assuming that the walls are perfectly rigid and a constant distance apart.
For aluminum, Y=7.0×1010Pa and prop =2.4×10−5k−1. The temperture change is ΔT,
ΔT=5.1C∘=5.1 K
Stress=FA=−Y∝ΔT
=−(0.70×1011Pa)(2.4×10−5k−1)(5.1K)
Stress =-8.6×106Pa
The total force F is the cross-sectional times the stress
F=(FA)A
=(20×10−4m2)(−8.6×106Pa)
=−1.7×104N
Topper's Solved these Questions
- THERMAL PROPERTIES OF MATTERBOOK - AAKASH INSTITUTECHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Try Youself
16 Videos
- THERMAL PROPERTIES OF MATTERBOOK - AAKASH INSTITUTECHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Assignment (Section-A) Objective Type questions (one option is correct)
50 Videos
- TEST 9BOOK - AAKASH INSTITUTECHAPTER - TEST 9EXERCISE - EXAMPLE
61 Videos
- THERMODYNAMICSBOOK - AAKASH INSTITUTECHAPTER - THERMODYNAMICSEXERCISE - ASSIGNMENT (SECTION -D) (Assertion - Reason Type Questions)
10 Videos
Similar Questions
An aluminium cylinder 10 cm long. As a spacer between two steel walls. At 12∘C it juct slips in between the walls. When it warms to 23∘C calculate the stress in the chlinder and the total force it exerts on each wall, assuming that the walls are perfectly rigid and a constant distance apart.
View SolutionA solid cylinder of mass M=10kg and cross - sectional area A=20cm2 is suspended by a spring of force contant k=100N/m and hangs partically immersed in water. Calculate the period of small oscillation of the cylinder.
View Solution
Knowledge Check
A wire of cross sectional area 3 mm2 is just stretched between two fixed points at a temperature of 20∘C . Then the tension in the wire when the temperature falls to 10∘C is,
(α=1.2×10−5/∘C,Y=2×1011N/m2)A80 NB60 NC72 ND50 NThe steel wire of cross-sectional area =2mm2[Y=200GPa,α=10−5.∘C] is stretched and tied firmly between two rigid supports, at a tempeture =20∘C. At this moment, the tension in the wire is 200N. At what temperature will the tension become zero?
A70∘CB40∘CC50∘CD30∘CA wire of cross-sectional area 3mm2 is first stretched between two fixed points at a temperature of 20∘C. Determine the tension when the temperature falls to 10∘C Coefficient of linear expansion α=10−5.∘C−1andY=2×1011N/m2
A20 NB30 NC60 ND120 N
Figure shows a steel rod of cross sectional ara 2×10−6m2 is fixed between two vertical walls. Initially at 20∘C there is no force between the ends of the rod and the walls. Find the force which the rod will exert on walls at 100∘C. Given that the coefficient of linear expansion of steel is 1.2×10−5.∘C−1 and Young's modulus is 2×1011N/m2

View SolutionA particle of mass m is trapped between two perfectly rigid parallel walls. The particle bounces back and forth between the walls without losing any energy. From a wave point of view, the particle trapped between the walls is like a standing wave in a stretched string between the walls. Distance between the two walls is L.
(a) Calculate the energy difference between third energy state and the ground state (lowest energy state) of the particle.
(b) Calculate the ground state energy if the mass of the particle is m=1mg and separation between the walls is L=1cm.
View SolutionA cylinder of mass 2kg and radius 10cm is held between two planks as shown in Fig. Calculate KE of the cylinder when there is no slipping at any point.
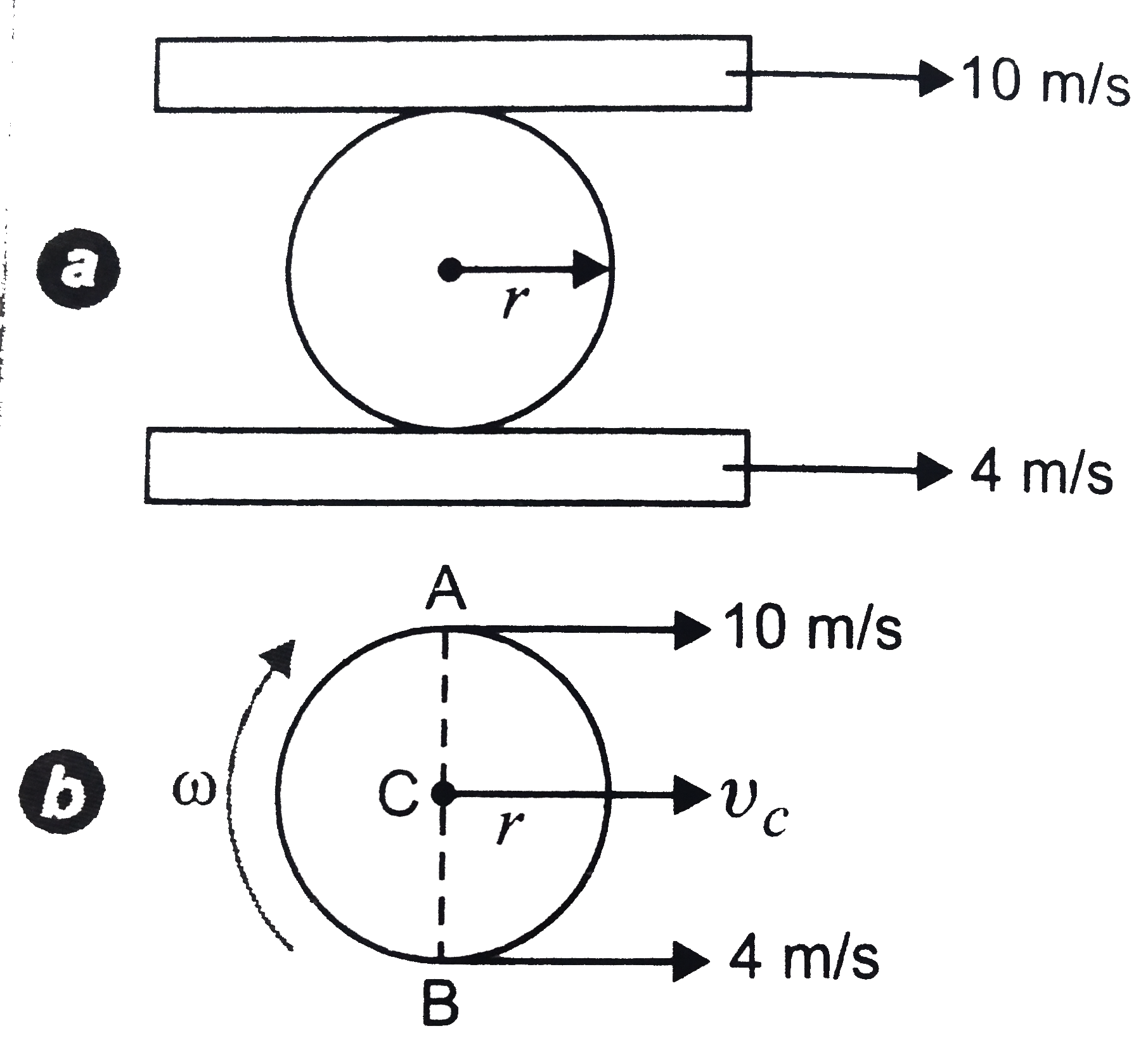
View SolutionA super-ball of mass m is to bounce elastically back and forth between two rigid walls at a distance d from each other. Neglecting gravity and assuming the velocity of super-ball to be v0 horizontally, the average force being exerted by the super-ball on each wall is
View SolutionAn iron cylindar of base area 7cm2 and mass 10 kg is melted and reshaped into a into a rod of area of cross section 1cm2.Calculate the increase in the pressure exerted on a plane surface when both the cylinder and the rod are made to stand vertically on a plane surface (g=10m−2)
View Solution
