Derive the relation $f = R / 2$ in the case of a concave mirror. Solution in Kannada

Video Solution

Text Solution

Verified by Experts

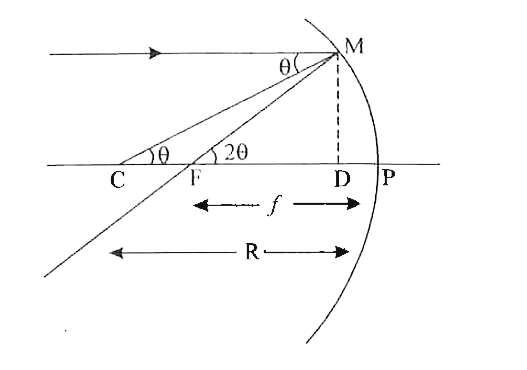
When a parallel beam of light is incident on a concave mirror, the rays are paraxial , i.e. they are incident at a point M , and make a small angle with principal axis. The reflected ray converges at a F on the principal axis. The point F is called the focus of the mirror ,the distance between the principal focus and the pole is called focal length (f).
Consider a ray parallel to the principal axis stricking the mirror at M, then
$∠ M F D = ∠ C M F + ∠ M C F = θ + θ = 2 θ$
$∠ M F D$ is the external angle of the $∠ M C F$
In $△^{le}$ MCD , $tan θ = \frac{MD}{CD}$ ...(1)
In $△^{le}$ MFD , tan $2 θ = \frac{MD}{FD}$ ...(2)
$θ$ is small , So $tan θ \approx θ, tan 2 θ \approx 2 θ$ ,
Substituting tan $θ$ and tan $2 θ$ in the equations,
$θ = \frac{MD}{CD}, 2 θ = \frac{MD}{FD}$
2xxcancel(MD)/(CD)=cancel(MD)/(FD) rArr 2/(CD)=1/(FD) on CD =2FD
Point D is very close to point P.
$∴ D F \approx P F \approx f$ and DC=PC=R
$\frac{2}{C P} = \frac{1}{F P}$ CP=3FP
R=2f
$f = \frac{R}{2}$

|

Updated on:21/07/2023

Class 12 PHYSICS PUE BOARD MODEL QUESTION PAPER 1

Topper's Solved these Questions

Similar Questions

SUBHASH PUBLICATION-PUE BOARD MODEL QUESTION PAPER 1-QUESTION

Home
Ask Doubt
Profile