Question

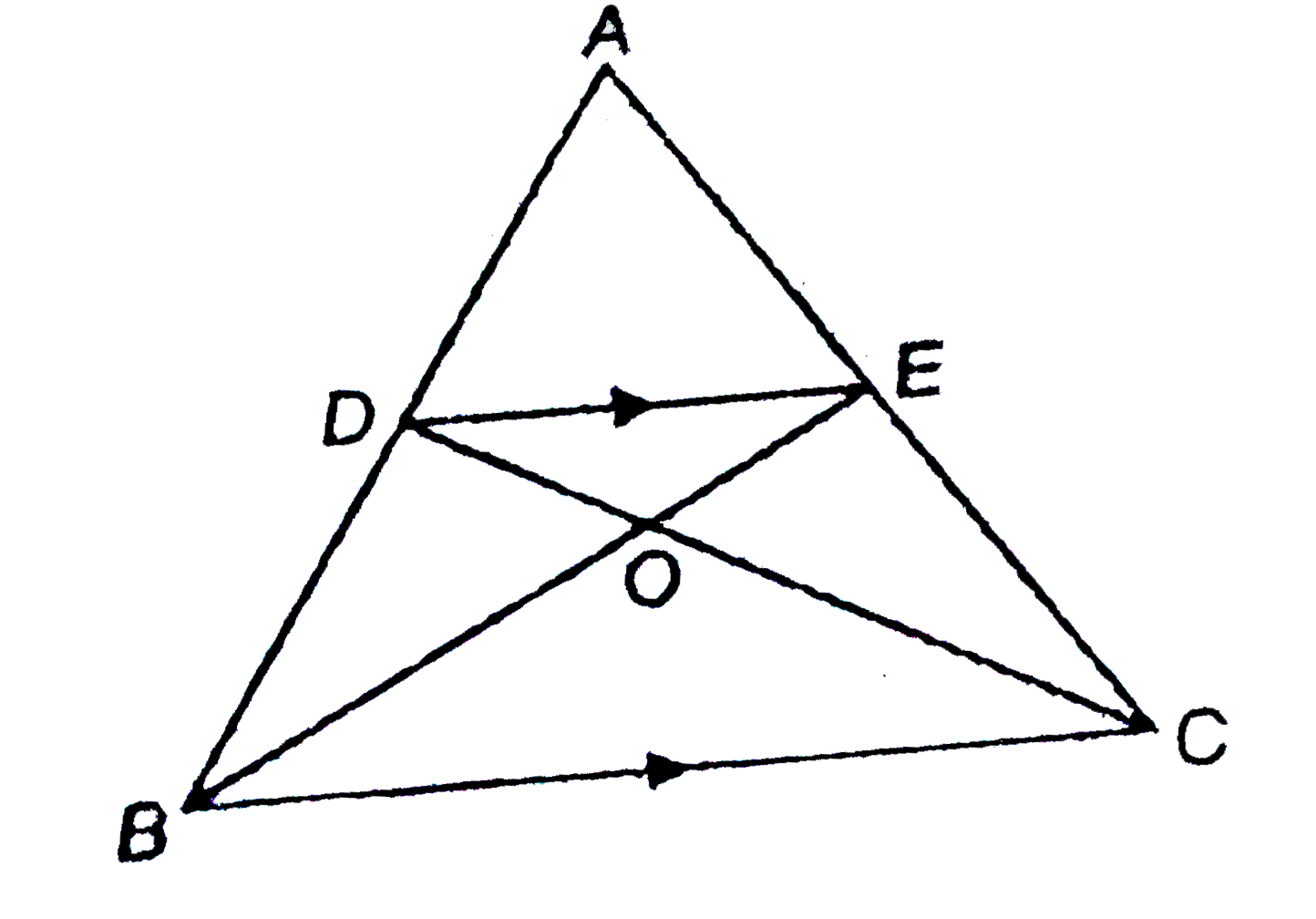
In the adjoining figure, AD is the median of $\Delta ABC$ and X be any point on side AD. Prove that:
area $(\Delta ABX)=\text{area}(\Delta ACX)$

Explanation

In `Delta ABC`,
D is the mid-point of BC.
`:.` area `(Delta ABD) = " area " (Delta ACD)`
(median divides the triangle into equal area) ..(1)
Again, in `Delta XBC`
D is the mid-point of BC
area `(DeltaXBD) = " area " (Delta XCD)`
(`.:` median divides the triangle into two equal areas) ..(2)
Subtract (2) from (1), we get
area `(Delta ABD) - " area " (Delta XBD) = " area " (Delta ACD) - " area " (Delta XCD)`
`rArr " area " (Delta ABX) = " area " (Delta ACX)`