The radius of curvature of the convex face of a plano convex lens is 12cm and the refractive index of the material of the lends 1.5. Then, the focal length of the lens is
6cm
12cm
18cm
24cm
The correct Answer is:D
To find the focal length of a plano-convex lens, we can use the lens maker's formula:
1f=μ−1(1R1−1R2)
Where:
- f is the focal length of the lens,
- μ is the refractive index of the lens material,
- R1 is the radius of curvature of the first surface (convex surface),
- R2 is the radius of curvature of the second surface (plano surface).
Step 1: Identify the values
- Given:
- Radius of curvature of the convex face, R1=+12cm (positive because it is convex)
- The second surface is plano, so R2=∞ (the radius of curvature for a flat surface is considered infinite)
- Refractive index, μ=1.5
Step 2: Substitute the values into the lens maker's formula
Using the lens maker's formula:
1f=μ−1(1R1−1R2)
Substituting the known values:
1f=(1.5−1)(112−1∞)
Step 3: Simplify the equation
Since 1∞=0, the equation simplifies to:
1f=0.5(112−0)
1f=0.5⋅112
Step 4: Calculate 1f
Calculating 1f:
1f=0.512=124
Step 5: Find the focal length f
Taking the reciprocal gives us:
f=24cm
Conclusion
The focal length of the plano-convex lens is 24cm.
---
- f is the focal length of the lens,
- μ is the refractive index of the lens material,
- R1 is the radius of curvature of the first surface (convex surface),
- R2 is the radius of curvature of the second surface (plano surface).
- Given:
- Radius of curvature of the convex face, R1=+12cm (positive because it is convex)
- The second surface is plano, so R2=∞ (the radius of curvature for a flat surface is considered infinite)
- Refractive index, μ=1.5
Using the lens maker's formula:
Since 1∞=0, the equation simplifies to:
Calculating 1f:
Taking the reciprocal gives us:
The focal length of the plano-convex lens is 24cm.
Topper's Solved these Questions
Similar Questions
The radius of curvature of the convex face of a plano-convex lens is12cm and its refractive index is1.5(a)Find the focal length of this lens.The plane surface of the lens is now silvered.(b)AT what distanc efrom the lens will parallel rays incident on the convex face converage?(c)Sketch the ray diagram to locate the image,when a point object is placed on the axis20cmfrom the lens(d)Calculate the image distance when the object is placed as in (c).
View SolutionThe redius of curvature of the convex face of a plano-convex lens is 12cm and its refractive index is 1.5.
a. Find the focal length of this lens. The plane surface of the lens is now silvered.
b. At what distance form the lans will parallel rays incident on the convex face converge?
c. Sketch the ray diagram to locate the image, when a point object is places on the axis 20cm from the lens.
d. Calculate the image distance when the object is placed as in (c).
View Solution
Knowledge Check
The radius of curvature of the curved surface of a plano-convex lens is 20cm. If the refractive index of the material of the lens be 1.5, it will
A30cmB50cmC20cmD40cmThe radius of curvature of the curved surface of a plano-convex lens is 20cm. If the refractive index of the material of the lens be 1.5, it will
Aact as a convex lens only for the object that lie on its curved side.Bact as a concave lens for the objects that lie on its curved side.Cact as a convex lens irrespective of the side on which the object lies.Dact as a concave lens irrespective of side on which the object lies.The radius of curvature of the convex surface of a thin plano-convex lens is 15 cm and the refractive index of its material is 1.6. The power of the lens is
A+1DB−2DC+3DD+4D
Find the radius of curvature of the convex surface of a plano-convex lens, whose focal length is 0.3 m and the refractive index of the material of the lens is 1.5.
View SolutionFind the radius of curvature of the convex surface of a plano-convex lens, whose focal length is 0.3 m and the refractive index of the material of the lens is 1.5.
View SolutionThe radius of curvature of the convex surface of a thin plano-convex lens is 12 cm, and the refractive index of its material is 1.6. What is the power of lens ?
View SolutionThe effective focal length of the lens combination shown in Fig. is −60cm. The radii of curvature of the curved surfaces of the plano-convex lenses are 12cm each and refractive index of the material of the lens is 1.5. The refractive index of the liquid is :
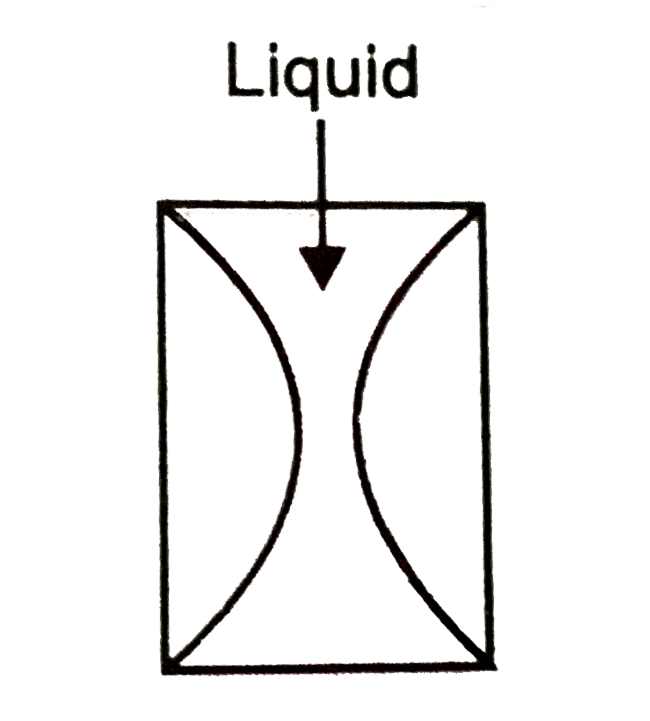 .
.
View SolutionThe radius of curvature of the convex surface of a planoconvex lens is 12 cm and its refractive index 1.5. If the plane face of the lens is silvered, then the distance from the lens at which parallel rays incident on its convex surface converge is
View Solution
