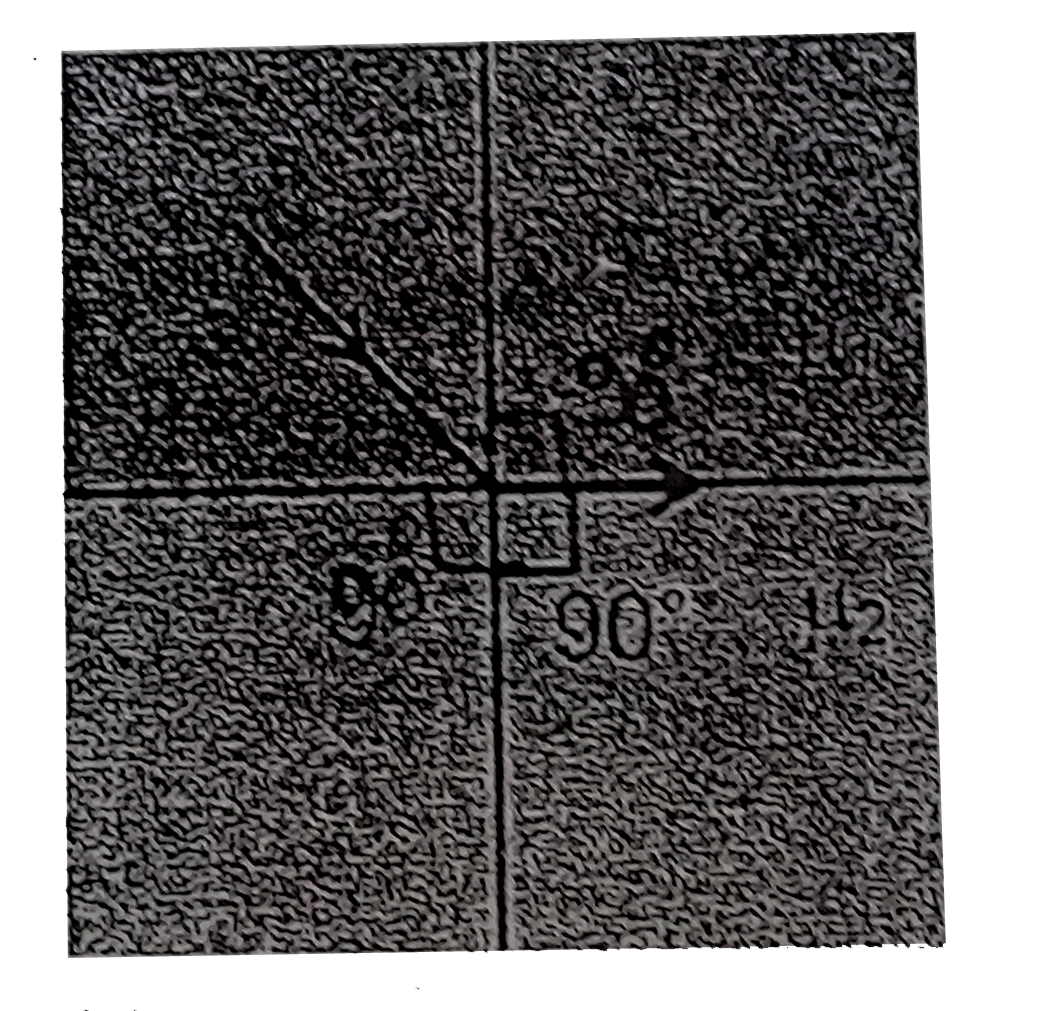
A vessel consists of two plane mirrors at right angles as shown in figure. The vessel is filled with water. The toal deviation in incident ray is

0∘
60∘
90∘
180∘
The correct Answer is:D
Angle between the two plance mirrors, θ=90∘
As relection is independent of medium Deviation produced by the combination of two plane mirrors is δ=2π−2θ=2π−2(π/2)=π=180∘
Topper's Solved these Questions
Similar Questions
Knowledge Check
A vessel consists of two plane mirrors at right angles (as shown in figure). The vessel is filled with water. The total deviation in incident ray is
 A0∘B90∘C180∘DNone of the above
A0∘B90∘C180∘DNone of the aboveA vessel consists of two plane mirrors at angles (as shown in figure). The vessel is filled with water. The total deviation in incident ray is
 A0∘B90∘C180∘DNone of these
A0∘B90∘C180∘DNone of theseA hollow prism is filled with water and placed in air. It will deviate the incident rays
Atowards the baseBaway from baseCparallel to baseDtowards or away from base depending on the location
If two plane mirrors are inclined at angle 0 to each other as shown, than angle of deviation of incident ray is

View SolutionA prism having an apex angle of 4∘ and refractive index of 1.50 is located in front of a vertical plane mirror as shown in the figure. A horizontal ray of light is incident on the prism. The total angle through which the ray is deviated is:
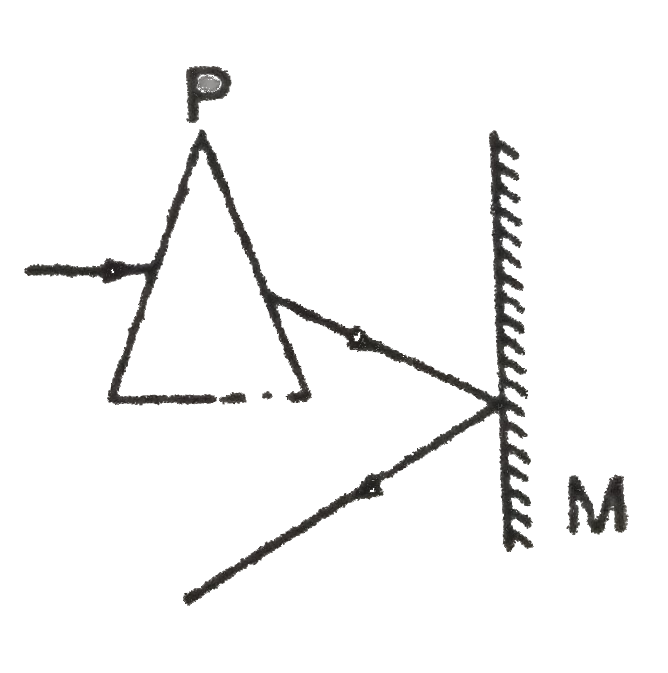
View SolutionP is small angled prism of angle 3∘ made of a material of refractive index 1.5. A ray of light is incident normally to the mirror as shown in figure. M is a plane mirror. The angle of deviation for the ray reflected from the mirror M with respect to the incident ray is :

View SolutionTwo plane mirrors are arranged at right angles to each other as shown in figure.A ray of light is incident on the horizontal mirror at an angle θ. For what value of θthe ray emerges parallel to the incoming ray after reflection from the vertical mirror ?
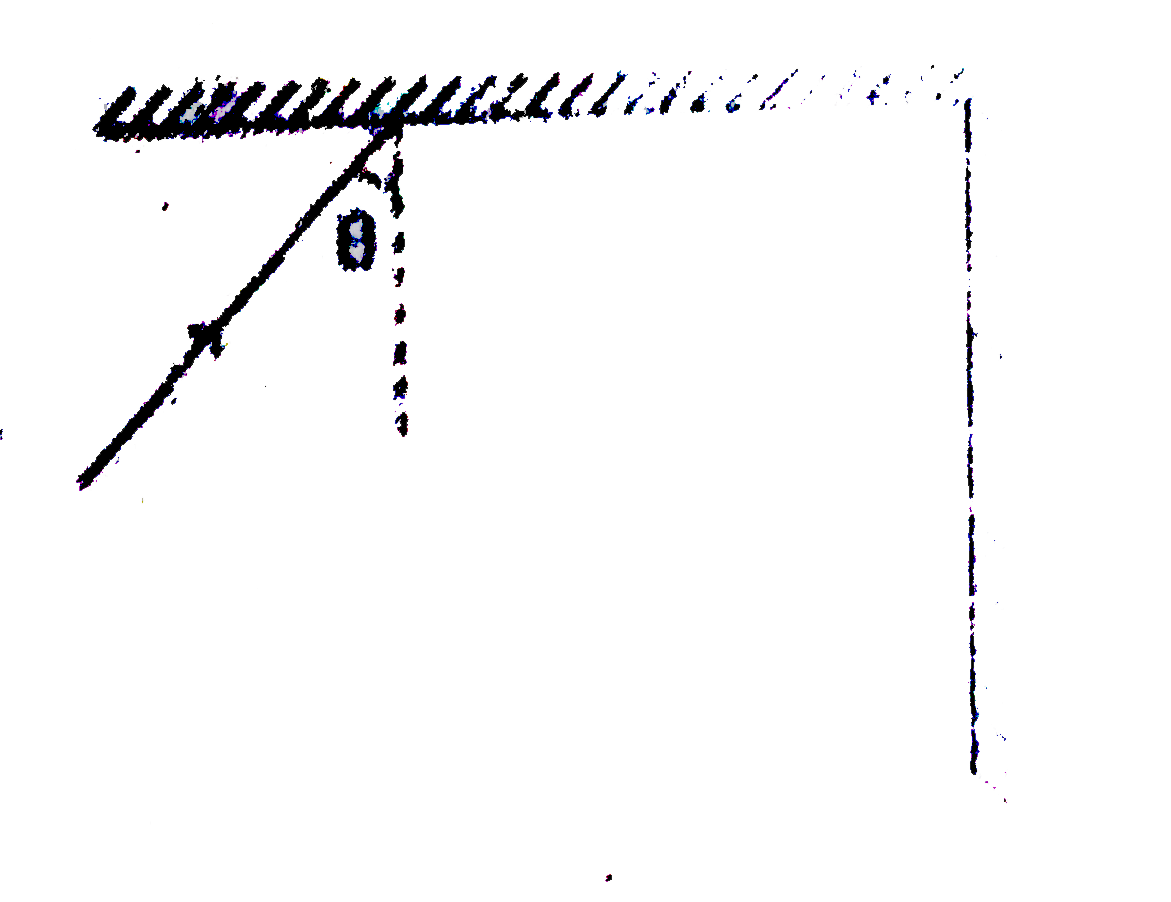
View SolutionAssertion : Two plane mirrors kept at right angles deviate any ray of light by 180∘ after two reflection.
Reason : The above condition is satisfied only for angle of incidence i=45∘.
View Solution