The velocity of image object and mirror both are moving towards each other with velocities 4ms−1and5ms−1 respectively, is
−14ms−1
15ms−1
−9ms−1
14ms−1
The correct Answer is:A
To solve the problem of finding the velocity of the image when both the object and the mirror are moving towards each other, we can follow these steps:
Step 1: Understand the velocities
- The object is moving towards the mirror with a velocity of vo=4m/s.
- The mirror is moving towards the object with a velocity of vm=5m/s.
Step 2: Set up the relationship
When both the object and the mirror are moving towards each other, the velocity of the object with respect to the mirror can be expressed as:
vom=vo+vm
where vom is the velocity of the object with respect to the mirror.
Step 3: Calculate the velocity of the object with respect to the mirror
Substituting the values:
vom=4m/s+5m/s=9m/s
Step 4: Relate the velocities of the object and the image
According to the mirror formula, the velocity of the object with respect to the mirror is equal and opposite to the velocity of the image with respect to the mirror:
vom=−vim
where vim is the velocity of the image with respect to the mirror.
Step 5: Solve for the velocity of the image
From the previous relationship:
9m/s=−vim
Thus,
vim=−9m/s
Conclusion
The velocity of the image is −9m/s. The negative sign indicates that the image is moving in the opposite direction to the defined positive direction (which is towards the mirror).
Final Answer
The velocity of the image is −9m/s.
---
- The object is moving towards the mirror with a velocity of vo=4m/s.
- The mirror is moving towards the object with a velocity of vm=5m/s.
When both the object and the mirror are moving towards each other, the velocity of the object with respect to the mirror can be expressed as:
vom=vo+vm
where vom is the velocity of the object with respect to the mirror.
Substituting the values:
vom=4m/s+5m/s=9m/s
According to the mirror formula, the velocity of the object with respect to the mirror is equal and opposite to the velocity of the image with respect to the mirror:
vom=−vim
where vim is the velocity of the image with respect to the mirror.
From the previous relationship:
9m/s=−vim
Thus,
vim=−9m/s
The velocity of the image is −9m/s. The negative sign indicates that the image is moving in the opposite direction to the defined positive direction (which is towards the mirror).
The velocity of the image is −9m/s.
Topper's Solved these Questions
Similar Questions
Find the velocity of the image when the object and mirror both are moving towards each other with velocities 2 and 3ms−1. How are they moving?
View SolutionTwo objects of masses 100g and 200g are moving along the same line in the same direction with velocities of 2m/s and 1m/s, respectively. They collide and after the collison, the first object moves at a velocity of 1.67m/s in the same direction. Determine the velocity of the second object.
View Solution
Knowledge Check
Two bodies of masses 5kg and 3kg are moving towards each other with 2ms−1 and 4ms−1 respectively. Then velocity of centre of mass is
A0.25ms−1 towards 3kgB0.5ms−1 towards 5kgC0.25ms−1 towards5kgD0.5ms−1 towards 3kgTwo trains are each 50 m long moving parallel towards each other at speeds 10ms−1 and 15ms−1 respectively, at what time will they pass each other ?
A8 sB4 sC2 sD6 sA plane mirror and a person are moving towards each other with same velocity v. Then the velocity of the image is
AvB2vC3vD4v
Figure shows a plane mirror and an object that are moving towards each other. Find the velocity of image.

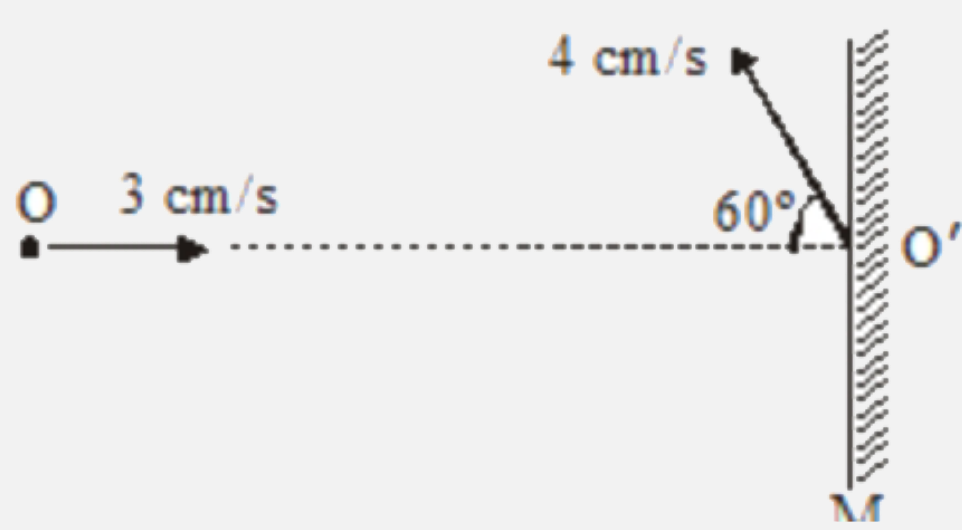
View SolutionA point object O and a mirror M move with velocities of 3cms−1 and 4cms−1 , respectively as shown in the figure. OO' is the normal to the mirror. What is the velocity of the image?
View SolutionTwo car travelling towards each other on a straith road at velocity 10ms−1 and 12ms−1 respectively. When they are 150 m apart, both the drivers apply their brakes and each car decelerates at 2ms−2 until it stops. How far apart will they be when both of them come to a halt ?
View SolutionA 10 kg ball and 20 kg ball approach each other with velocities 20ms−1 and 10ms−1 respectively . What are their velocities after collision if the collision is perfectly elastic ?
View SolutionTwo particles are thrown horizontally in opposite directions from the same point from a height h with velocities 4ms−1and3ms−1 . What is the separation between them when their velocities are perpendicular to each other?
View Solution
