Which of the following represents uniformly accelerated motion ?
x=√t+ab
x=t+ab
t=√x+ab
x=√t+a
The correct Answer is:C
To determine which of the given options represents uniformly accelerated motion, we can follow these steps:
1. Understand the Equation of Motion:
The general equation for uniformly accelerated motion is given by:
x=xi+ut+12at2
where:
- x is the final position,
- xi is the initial position,
- u is the initial velocity,
- a is the constant acceleration,
- t is the time.
2. Analyze Each Option:
We need to compare each option with the standard equation of motion to see if it matches.
- Option A: x=√t+ab
- This does not have a t2 term, hence it does not represent uniformly accelerated motion.
- Option B: x=b+a
- This is a constant equation with no time variable, thus it does not represent motion, let alone uniformly accelerated motion.
- Option C: t=√x+ab
- Rearranging this gives us:
t2=x+ab
- Now, if we express x:
x=t2−ab
- This can be rewritten as:
x=−ab+t2
- This matches the form of the equation of motion where xi=−ab, u=0, and a=b.
- Option D: x=−b+bt2
- This can be rearranged to:
x=−b+bt2
- This does not match the standard form of uniformly accelerated motion.
3. Conclusion:
After analyzing all options, we find that Option C is the only one that can be rearranged to fit the equation of uniformly accelerated motion.
Final Answer:
Option C represents uniformly accelerated motion.
The general equation for uniformly accelerated motion is given by:
x=xi+ut+12at2
where:
- x is the final position,
- xi is the initial position,
- u is the initial velocity,
- a is the constant acceleration,
- t is the time.
We need to compare each option with the standard equation of motion to see if it matches.
- This does not have a t2 term, hence it does not represent uniformly accelerated motion.
- This is a constant equation with no time variable, thus it does not represent motion, let alone uniformly accelerated motion.
- Rearranging this gives us:
t2=x+ab
- Now, if we express x:
x=t2−ab
- This can be rewritten as:
x=−ab+t2
- This matches the form of the equation of motion where xi=−ab, u=0, and a=b.
- This can be rearranged to:
x=−b+bt2
- This does not match the standard form of uniformly accelerated motion.
After analyzing all options, we find that Option C is the only one that can be rearranged to fit the equation of uniformly accelerated motion.
Option C represents uniformly accelerated motion.
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - DC PANDEYCHAPTER - MOTION IN A PLANEEXERCISE - (B) Meical entrance special format questions (Assertion and reason)
19 Videos
- MOTION IN A PLANEBOOK - DC PANDEYCHAPTER - MOTION IN A PLANEEXERCISE - (B) Meical entrance special format questions (Mathch the columns)
6 Videos
- MOTION IN A PLANEBOOK - DC PANDEYCHAPTER - MOTION IN A PLANEEXERCISE - Check point 3.7
15 Videos
- MOTIONBOOK - DC PANDEYCHAPTER - MOTIONEXERCISE - Medical entrances gallery
19 Videos
- PROJECTILE MOTIONBOOK - DC PANDEYCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 Subjective
10 Videos
Similar Questions
Knowledge Check
The relation between position (x) and time (t) are given below for a particle moving along a straight line. Which of the following equation represents uniformly accelerated motion? [where αandβ are positive constants]
Aβx=αt+αβBαx=β+tCxt=αβDαt=√β+xWhich of the following graphs represent a uniformaly accelerated motion ?
A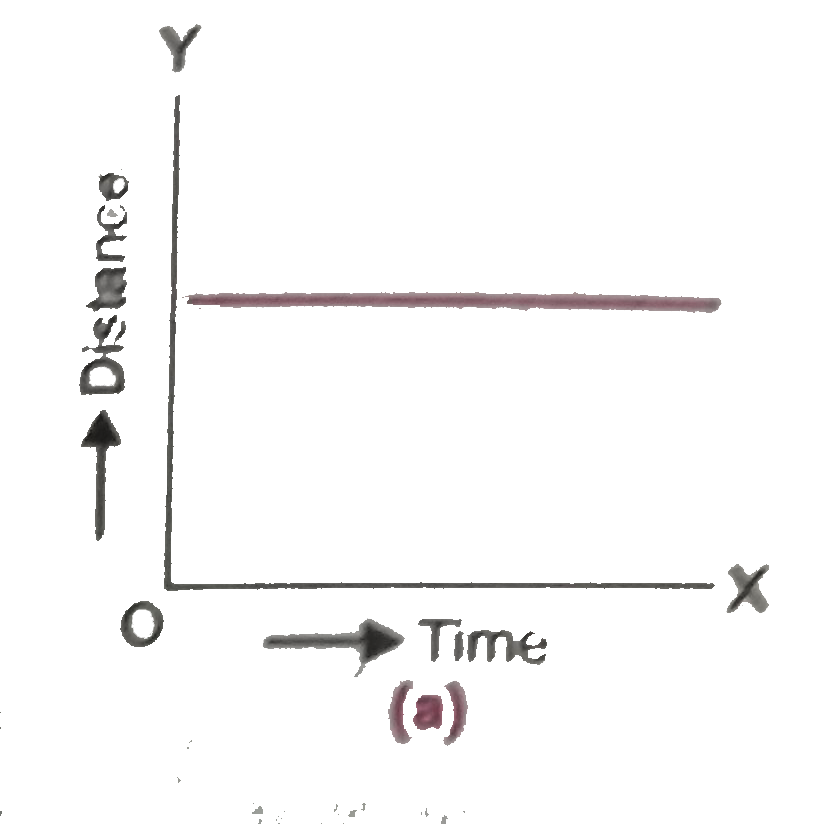 B
B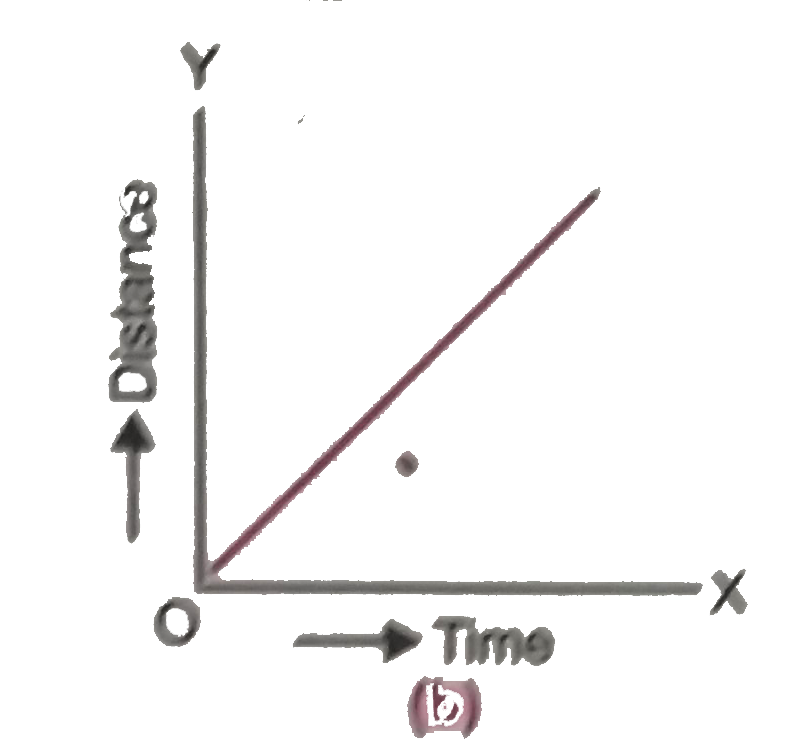 C
C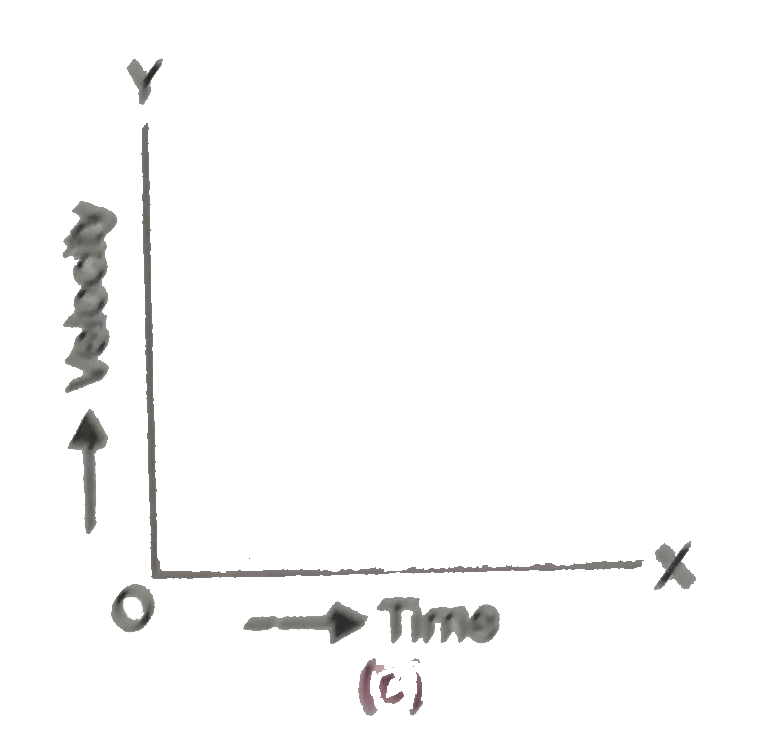 D
D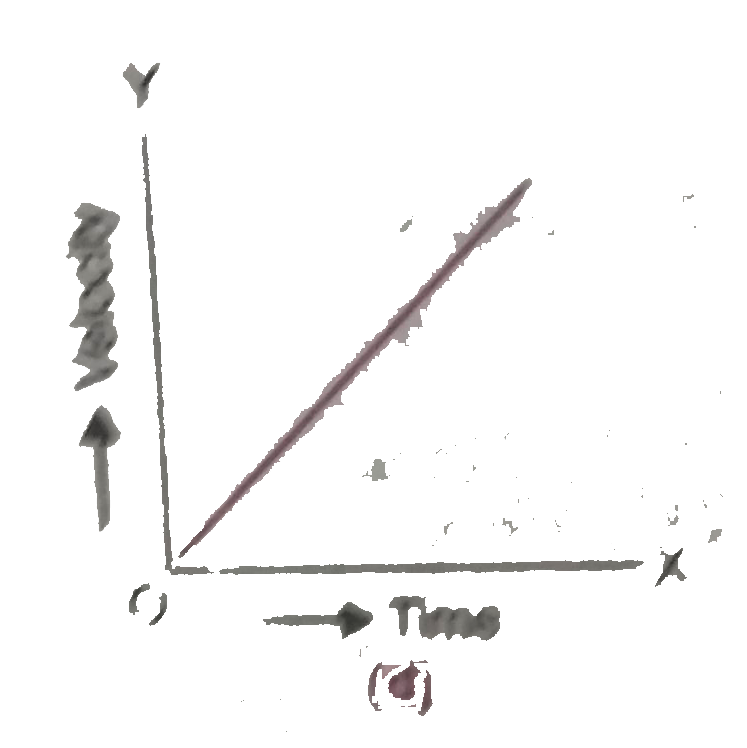
Which of the following graph represents uniform motion
A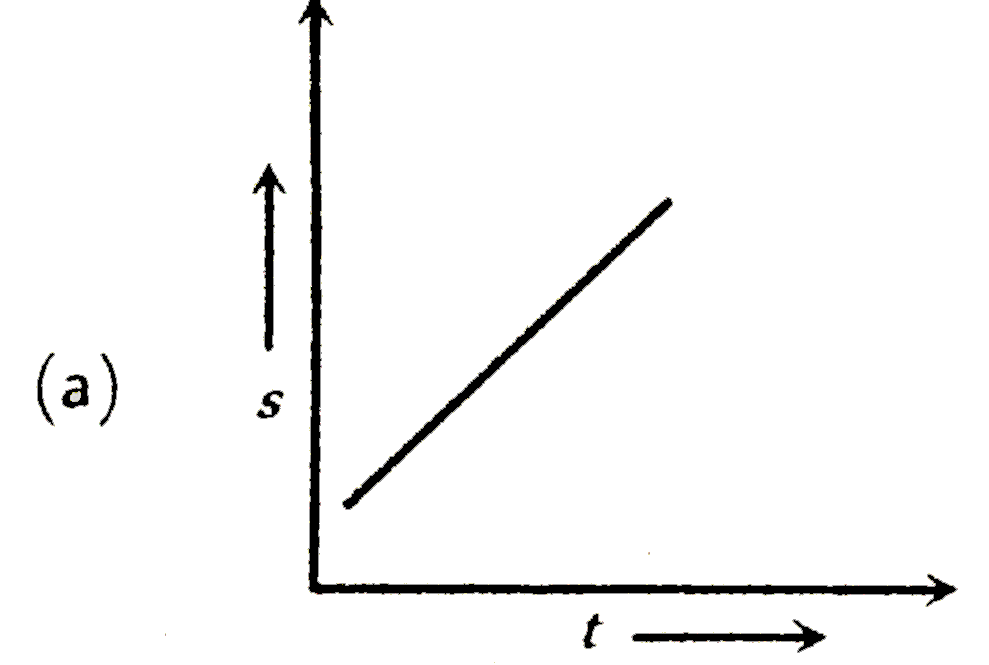 B
B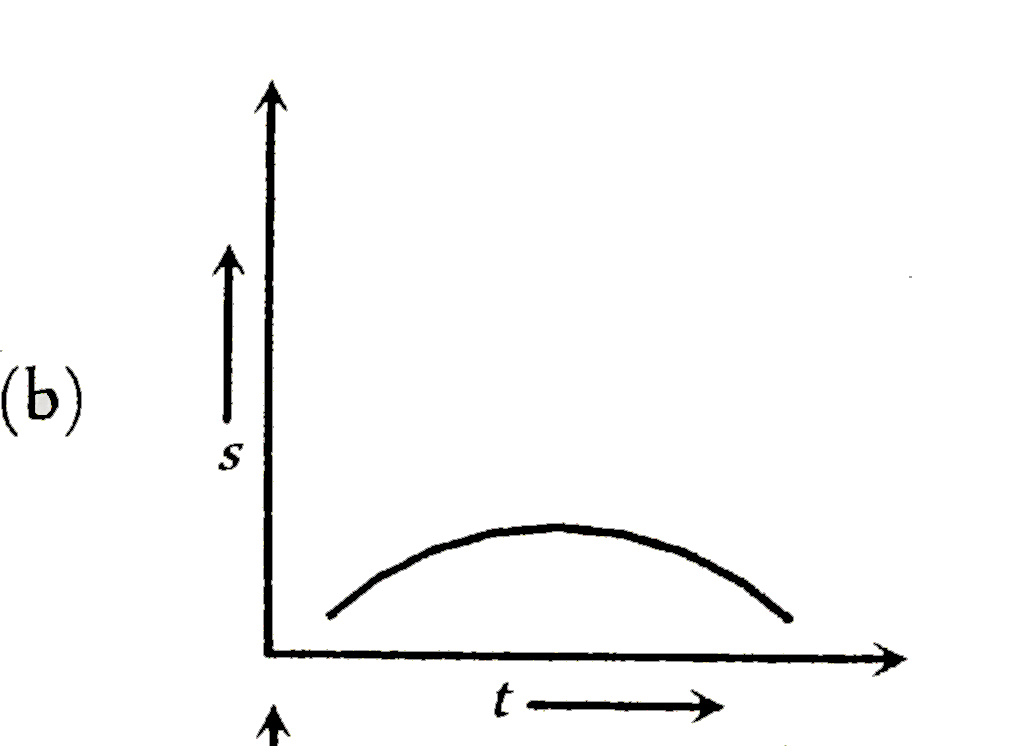 C
C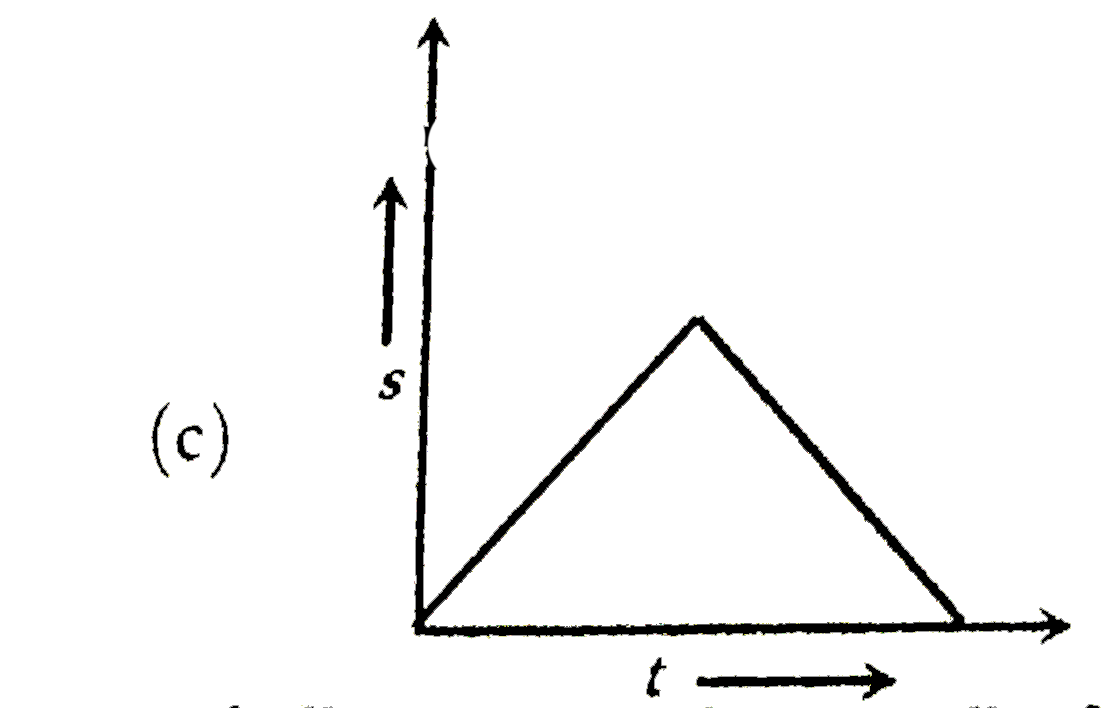 D
D
Deduce the following equations for uniformly accelerated motion by using integration technique : s=ut+12at2
View SolutionDeduce the following equations for uniformly accelerated motion by using integration technique : v = u + at
View SolutionNon uniformly accelerated motion using integration
View SolutionWhich of the following figures represents uniform motion of a moving object correctly ?
View SolutionWhich of following graphs represent accelerated motion ?
View Solution
