A bullet emerges from a barrel of length 1.2m with a speed of 640ms1. Assuming constant acceleration, after the gun is fired is
4m
40m
400us
1s
The correct Answer is:B
To solve the problem of a bullet emerging from a barrel with a given length and speed, we will use the equations of motion under constant acceleration. Here’s a step-by-step solution:
Step 1: Identify the known values
- Length of the barrel (s) = 1.2 m
- Final speed (v) = 640 m/s
- Initial speed (u) = 0 m/s (since the bullet starts from rest)
Step 2: Use the third equation of motion
The third equation of motion relates the final velocity, initial velocity, acceleration, and displacement:
v2=u2+2as
Where:
- v = final velocity
- u = initial velocity
- a = acceleration
- s = displacement (length of the barrel)
Step 3: Substitute the known values into the equation
Substituting the known values into the equation:
(640)2=(0)2+2a(1.2)
Step 4: Simplify the equation
This simplifies to:
409600=2a(1.2)
409600=2.4a
Step 5: Solve for acceleration (a)
Now, solve for a:
a=4096002.4
a=170666.67m/s2
Step 6: Use the first equation of motion to find time (t)
Now we can use the first equation of motion to find the time taken for the bullet to travel through the barrel:
v=u+at
Since u=0:
640=0+(170666.67)t
t=640170666.67
t≈0.00374s
Step 7: Convert time to milliseconds
To convert seconds to milliseconds:
t≈0.00374s×1000ms/s
t≈3.74ms
Final Answer
The time taken for the bullet to travel through the barrel is approximately 3.74 milliseconds.
---
- Length of the barrel (s) = 1.2 m
- Final speed (v) = 640 m/s
- Initial speed (u) = 0 m/s (since the bullet starts from rest)
The third equation of motion relates the final velocity, initial velocity, acceleration, and displacement:
v2=u2+2as
Where:
- v = final velocity
- u = initial velocity
- a = acceleration
- s = displacement (length of the barrel)
Substituting the known values into the equation:
(640)2=(0)2+2a(1.2)
This simplifies to:
409600=2a(1.2)
409600=2.4a
Now, solve for a:
a=4096002.4
a=170666.67m/s2
Now we can use the first equation of motion to find the time taken for the bullet to travel through the barrel:
v=u+at
Since u=0:
640=0+(170666.67)t
t=640170666.67
t≈0.00374s
To convert seconds to milliseconds:
t≈0.00374s×1000ms/s
t≈3.74ms
The time taken for the bullet to travel through the barrel is approximately 3.74 milliseconds.
Topper's Solved these Questions
- MOTION IN A PLANEBOOK - DC PANDEYCHAPTER - MOTION IN A PLANEEXERCISE - (B) Meical entrance special format questions (Assertion and reason)
19 Videos
- MOTION IN A PLANEBOOK - DC PANDEYCHAPTER - MOTION IN A PLANEEXERCISE - (B) Meical entrance special format questions (Mathch the columns)
6 Videos
- MOTION IN A PLANEBOOK - DC PANDEYCHAPTER - MOTION IN A PLANEEXERCISE - Check point 3.7
15 Videos
- MOTIONBOOK - DC PANDEYCHAPTER - MOTIONEXERCISE - Medical entrances gallery
19 Videos
- PROJECTILE MOTIONBOOK - DC PANDEYCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 Subjective
10 Videos
Similar Questions
A machine gun has a mass of 20kg If fires 25g bullets at the rate of 600 bullets per minute with a speed of 200ms−1 Calculate (i) recoil velocity of the gun (ii) force required to keep the gun in position .
View SolutionA shell leaves the barrel of a gun at a speed of 800 m/s. The barrel is 2.0 m long. Find the average acceleration.
View Solution
Knowledge Check
A machine gun has a mass 4 kg. It fires 50 gram bullets at the rate of 45 bullets per minute at a speed of 400ms−1. What force is required to keep the gun in position ?
A10NB13.3NC15ND26NA machine gun has a mass 5 kg. It fires 50 gram bullets at the rate of 30 bullets per minute at a speed of 400 m s−1. What force is required to keep the gun in positon ?
A10 NB5 NC15 ND30 NA machine gun is mounted on an armored car moving with a speed of 20ms−1. The gun point against the direction of motion of car. The muzzle speed of bullet is equal to speed of sound in air i.e., 340ms−1. The time difference between bullet actually reaching and sound of firing reaching at a target 544m away from car at the instant of firing is reaching at a target 544m away from car at the instant of firing is
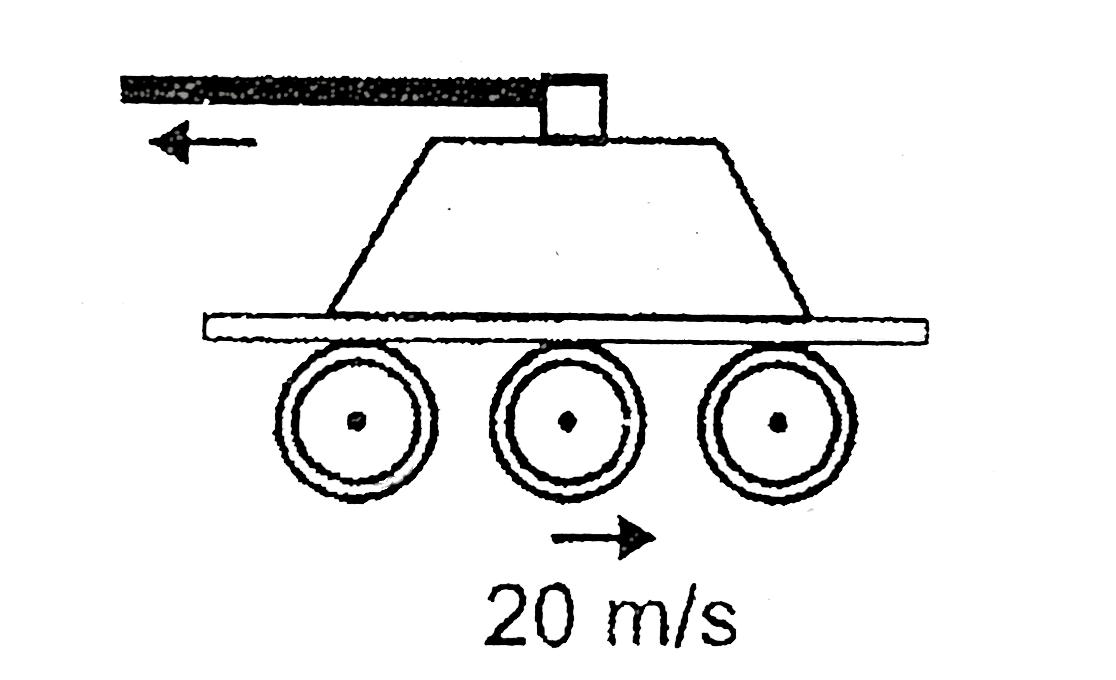 A1.2sB0.1sC1sD10s
A1.2sB0.1sC1sD10s
A machine gun of mass 10 kg fires 20 g bullets at the rate of 10 bullets per second with a speed of 500 ms−1. What force is required to hold the gun in position ?
View SolutionA bullet fired from a gun with a velocity of 140ms−1 strikes the ground at the same level as the gun at a distance of 1 km. Find the angle of inclination with the horizontal at which the bullet is fixed. Take g=9.8ms−2.
View SolutionA 50 g bullet is fired from a 10 kg gun with a speed of 500 ms−1. What is the speed of the recoil of the gun.
View SolutionA gun fire bullets each of mass 1 g with velocity of 10ms−1 by exerting a constant force of 5 g weight. Then , the number of bullets fired per second is (take g = 10ms−2 )
View SolutionA pendulum consists of a wooden bob of mass m and length l. A bullet of mass m1 is fired towards the pendulum with a speed v1. The bullet emerges out of the bob with a speed of (v1)/3 and the bob just completes motion along a vertical circle, then v1 is
View Solution