Given that →A+→B+→C=0, out of three vectors two are equal in magnitude and the magnitude of third vector is √2 times that of either of two having equal magnitude. Then angle between vectors are given by
30∘,60∘,90∘
45∘,45∘,90∘
90∘,135∘,45∘
90∘,135∘,135∘
The correct Answer is:D
(d) Angle between A and B Is 90∘
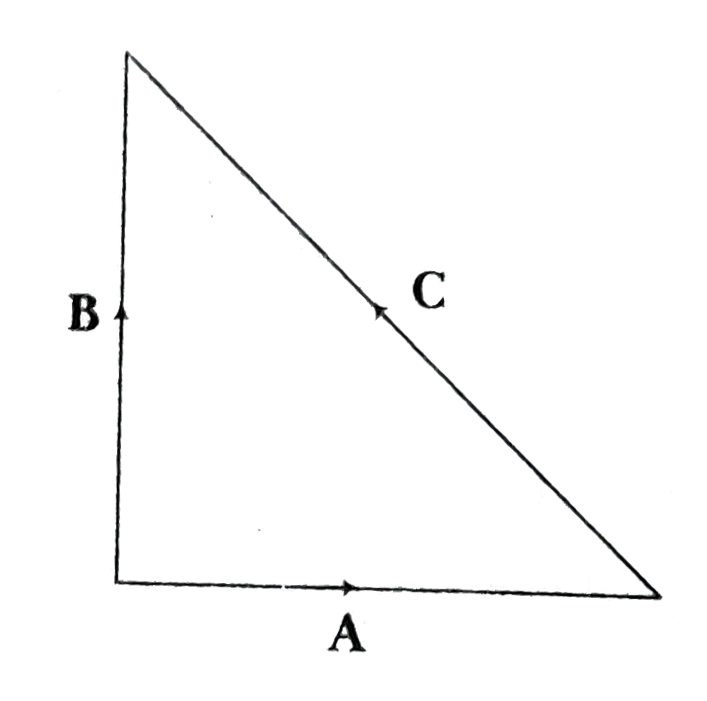
Between B and C 135∘and that betweeen A and C is 135∘
Topper's Solved these Questions
Similar Questions
Given that →A+→B+→C=→0. Out of three vectors,two are equal in magnitude and the magnitude of the third vectors is √2 times that of either of the two having equal magnitude. Find the angles between the vectors.
View SolutionGiven: →a+→b+→c=0. Out off three vectors →a,→band→c two are equal in magnitude. The magnitude of third vector is √2 times that of either of the two having equal magnitude. The angle between vectors is
View Solution
Knowledge Check
given that →A+→B+→C=0 out of three vectors two are equal in magnitude and the magnitude of third vector is √2 times that of either of the two having equal magnitude. Then the angles between vectors are given by
A30∘,60∘,90∘B40∘,45∘,90^@C45∘,60∘,90∘D90∘,135∘,135∘Given that P+Q+R= 0. Two out of the three vectors are equal in magnitude. The magnitude fo the third vector is s √2 times that of the other two. Which of the following can be the angles between these vectors?
A90∘B45∘,45∘,90∘C30∘,60∘,90∘D45∘,90∘,135∘If A and B are two non- zero vectors having equal magnitude, then angle between the vectors A and A−B is
A0∘B90∘C180∘Ddependent on the orientation of A and B
There vectors →P,→Qand→R are such that →P+→Q+→R=0 Vectors →P and →Q are equal in , magnitude . The magnitude of vector →R is √2 times the magnitude of either →Por→Q . Calculate the angle between these vectors .
View SolutionThe magnitude of the sum of two vectors is equal to the difference in their magnitudes. What is the angle between vectors?
View SolutionTwo vectors of equal magnitude are acting through a point. The magnitude of resultant is equal to the magnitude of either vectoe. Find the angle between the vectors.
View SolutionThe magnitude of the vector product of two vectors is √3 times their scalar product. The angle between the two vectors is
View SolutionIf A and B are Two non -zero vector having equal magnitude , the angle between the vector A and A-B is
View Solution
