A small block starts sliding down an inclined plane forming an angle $45^{\circ}$ horizontal. The coefficient of friction $μ$ , varies with distence s as $μ = c s^{2}$ wherem, c is a constant of appropriate dimension, then distence covered by the block before it stops is Solution in Telugu

A

$\sqrt{\frac{3}{c}}$

B

$\sqrt{3 C}$

C

$\sqrt{C}$

D

$\sqrt{\frac{1}{C}}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Given that,

$μ = C s^{2} {∴ s = distance and C = constant)$
Net force o9n the block is

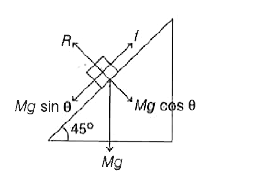
$M g sin θ 0 f = M a$
$M g sin θ - μ M g cos θ = m a$
$g sin θ - μ g cos θ = a$
$g [sin θ - C s^{2} cos θ] = a$
$∴ a = \frac{d v}{d t} = v \frac{d v}{d s}$
$a d s = v d v$
From Eqs. (i) and (ii), we get
$\Rightarrow g [sin θ - C s^{2} cos θ] d s = v d v$
Integrating both sides, we get
$\Rightarrow (g sin θ) s - \frac{g (C s^{3})}{3} cos θ = \frac{v^{2}}{2} + K ... (i i i)$
For $θ = 45^{\circ}$ , we have,
$(\frac{g}{\sqrt{2}}) s - (\frac{C s^{2}}{3}) \frac{g}{\sqrt{2}} = \frac{v^{2}}{2} + K$
Initially $t = 0, s = 0, u = 0$ , substituting these, we get k = 0
So, $\frac{g}{\sqrt{2}} (s - \frac{C s^{2}}{3} = \frac{v^{2}}{2} \times \frac{\sqrt{2}}{g})$
$\Rightarrow s = \frac{C s^{3}}{3} = \frac{v^{2}}{2} \times \frac{\sqrt{2}}{g}$
The body stops, when v = 0 $\Rightarrow s - \frac{C s^{3}}{3} = 0$
$\Rightarrow s = \frac{C s^{3}}{3} \Rightarrow s = \sqrt{\frac{3}{C}}$

|

Updated on:21/07/2023

Class 12 PHYSICS ONLINE QUESTION PAPER 2018

Topper's Solved these Questions

Similar Questions

TS EAMCET PREVIOUS YEAR PAPERS-ONLINE QUESTION PAPER 2018-PHYSICS

Home
Ask Doubt
Profile