A :
R : Solution in Telugu
Both A and R are true and R is the correct explanation of A
Both A and R are true but R is not correct explanation of A
A is true but R is false
A is false but R is true
The correct Answer is:A
Step by step video, text & image solution for A : (1)/(2)-(1)/(2).(1)/(2^(2))+(1)/(3).(1)/(2^(3))-(1)/(4).(1)/(2^(4))+....=log_(e)((3)/(2)) R : log_(e)(1+x)=x-(x^(2))/(2)+(x^(3))/(3)-(x^(4))/(4)+... by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- EXPONENTIAL SERIES & LOGARITHMIC SERIES (APPENDIX-1)BOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - EXPONENTIAL SERIES & LOGARITHMIC SERIES (APPENDIX-1)EXERCISE - EXERCISE 2 SET - 3
5 Videos
- ELLIPSEBOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - ELLIPSEEXERCISE - EXERCISE 2 SET - 2
1 Videos
- FUNCTIONSBOOK - DIPTI PUBLICATION ( AP EAMET)CHAPTER - FUNCTIONSEXERCISE - EXERCISE 2 (SPECIAL TYPE QUESTIONS)
7 Videos
Similar Questions
Match the following
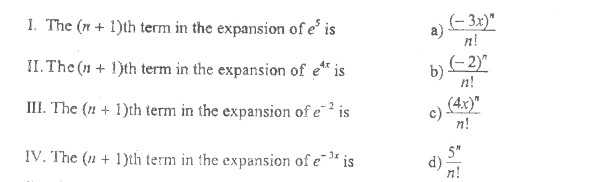
View SolutionThe equation of the chord of contact of the point (1, -2) w.r.t the ellipse 4x2+5y2=20 is
View SolutionI : Ever function must be either even or odd function
The function f(x)=log(x+√x2+1) is an odd function.
View Solutionlog_((3)/(4))log_(8)(x^(2)+7)+log_((1)/(2))log_((1)/(4))(x^(2)+7)^(-1)=-2
View Solutionlog_(2)(4^(x)+4)=log_(2)2^(x)+log_(2)(2^(x+1)-3)
View SolutionIf 1,log_(2)(3^(1-x)+2),log_(2)(4*3^(3)-1) are in A.P then x equals to
View Solutionlim_(x rarr a){[(a^((1)/(2))+x^((1)/(2)))/(a^((1)/(4))-x^((1)/(4))))^(-1)-(2(ax)^((1)/(4)))/(x^((3)/(4))-a^((1)/(4))x^((1)/(2))+a^((1)/(2))x^((1)/(4))-a^((3)/(4)))]^(-1)-sqrt(2)^(log_(4)a)}^(8)
View Solutionlog_((3)/(4))log_(8)(x^(2)+7)+log_((1)/(2))log_((1)/(4))(x^(2)+7)^(-1)=-2
View SolutionThe expression: (((x^(2)+3x+2)/(x+2))+3x-(x(x^(3)+1))/((x+1)(x^(2)+1))-log_(2)8)/((x-1)(log_(2)3)(log_(3)4)(log_(4)5)(log_(5)2)) reduces to
View SolutionIf xy2=4andlog3(log2x)+log1/3(log1/2y)=1 , then x equals
View SolutionIf x=log12.43.log2.13.log230.8, then ______.
View SolutionSolve the equation log1/3[2(12)x−1]=log1/3[(14)x−4]
View Solution1+x(loge2)+x22!(loge2)2+x33!(loge2)3+....=
View SolutionA : a−ba+12(a−ba)2+13(a−ba)3+....=loge(ab)
R : loge(1−x)=−x−x22−x33−x44−....
View SolutionIf xy2=4andlog3(log2x)+log1/3(log1/2y)=1 , then x equals
View SolutionIf 3x=4x−1 , then x = a. 2log322log32−1 b. 22−log23 c. 11−log43 d. 2log232log23−1
View Solution
