A steel rod of length 100 cm is clamped at the middle. The frequency of the fundamental mode for the longitudinal vibrations of the rod is
(Speed of sound in steel = 5 km s−1)
1.5 KHz
2 KHz
2.5 KHz
3 KHz
The correct Answer is:C
Here,
L=100 cm=1 m,
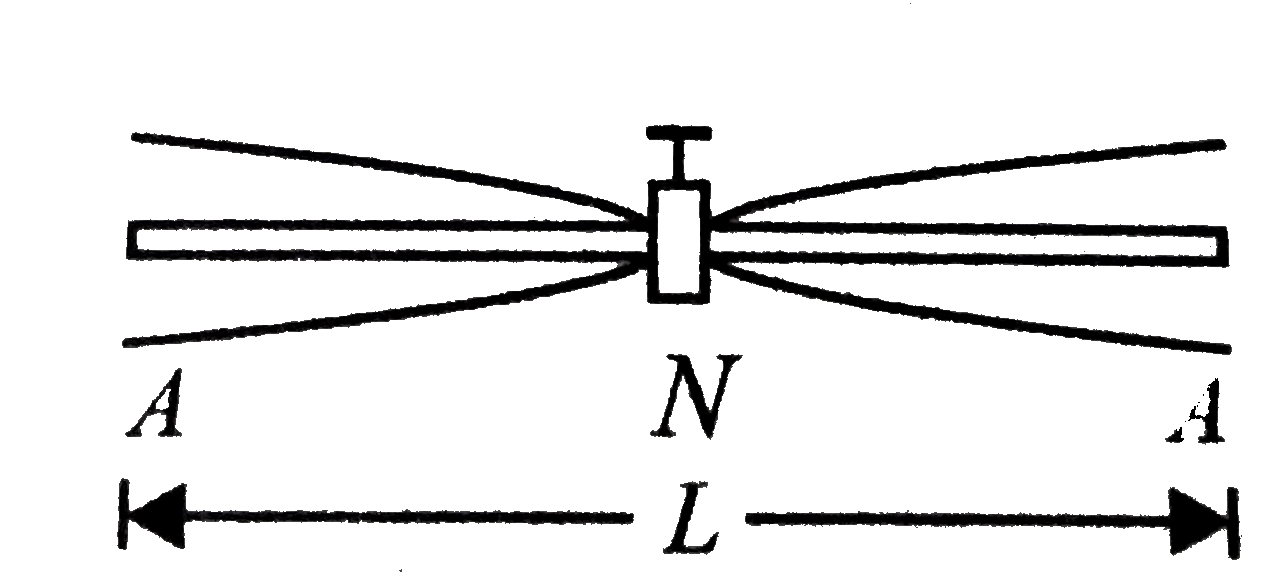
As the rod is clamped at the middle, therefore the middle point is a node. In the fundamental mode, the antinode is formed at each end as shown in figure.
Therefore, the distance two consecutive antinodes =L=1m But the distance between two consecutive antinodes is
λ2.
∴λ2=1morλ=2m
The frequency of the fundamental mode is
υ=vλ=5×103ms−12m=2.5×103Hz=2.5kHz
Topper's Solved these Questions
- WAVESBOOK - NCERT FINGERTIPSCHAPTER - WAVESEXERCISE - Beats
12 Videos
- WAVESBOOK - NCERT FINGERTIPSCHAPTER - WAVESEXERCISE - Doppler Effect
10 Videos
- WAVESBOOK - NCERT FINGERTIPSCHAPTER - WAVESEXERCISE - The Principle Of Superposition Of Waves
2 Videos
- UNITS AND MEASUREMENTSBOOK - NCERT FINGERTIPSCHAPTER - UNITS AND MEASUREMENTSEXERCISE - Assertion And Reason
15 Videos
- WORK , ENERGY AND POWERBOOK - NCERT FINGERTIPSCHAPTER - WORK , ENERGY AND POWEREXERCISE - Assertion And Reason
15 Videos
Similar Questions
A steel rod 100 cm long is clamped at its middle. The fundamental frequency of longitudinal vibrations of the rod is given to be 2.53k Hz. What is the speed of sound in steel?
View SolutionA steel rod 100 cm long is clamped at its middle. The fundamental frequency of longitudinal vibrations of the rod is given to be 2.53k Hz. What is the speed of sound in steel?
View Solution
Knowledge Check
A steel rod 100 cm long is clamped at its midpoint. The fundamental frequency of longitudinal vibrtions of the rod is 3 kHz. What is the speed of the sound in the rod?
A3×103ms−1B4×103ms−1C5×103ms−1D6×103ms−1A steel rod 100 cm long is clamped at its middle. The fundamental frequency of loungitudinal vibrations of the fundamental frequency of longitudinal vibrations of the rod are given to be 2.53 KHz. What is the speed of soind in steel?
A6.2 km/sB5.06 km/sC7.23 km/sD7.45 km/sAn aluminium rod having a length 100 cm is clamped at its middle point and set into longitudinal vibrations. Let the rod vibrate in its fundamental mode. The density of aluminium is 2600 kg/m3 and its Young's modulus is 7.8×1010N/m2. The frequency of the sound produced is :-
A1250 HzB2740 HzC2350 HzD1685 Hz
A steel rod 100 cm long is clamped at its centre. The fundamental frequency of longitudinal vibrations of the rod are given to be 2.53kHz. What is the speed of sound in steel?
View SolutionA copper rod of length l=50cm is clamped at its midpoint. Find the number of natural longitudinal oscillations of the rod in the frequency range from 20 to 50kHz. What are those frequencies equal to ?
View SolutionA copper rod of length 1.0 m is clamped at its middle point. Find the frequencies between 20 Hz and 20,000 Hz at which standing longitudinal waves can be set up in the rod. The speed of sound in copper is 3.8kms−1.
View SolutionA Kundt's tube apparatus has a steel rod of length 1.0 m 66. clamped at the centre. It is vibrated in its fundamental mode at a frequency of 2600 Hz. The lycopodium powder dispersed in the tube collects into heaps separated by 6.5 cm. Calculate the speed of sound in steel and in air.
View SolutionAn aluminium rod having a length 100 cm is clamped at its middle point and set into longitudinal vibrations. Let the rod vibrate in its fundamental mode. The density of aluminium is 2600 kg/m3 and its Young's modulus is 7.8×1010N/m2. The frequency of the sound produced is :-
View Solution
