Two cylinder having m1g and m2g of a gas at pressure P1 and P2 respectively are put in communication with each other, temperature remaining constant. The common pressure reached will be
P1P2(m1+m2)P2m1+P1m2
P1P2m1P2m1+P1m2
m1m2(P1+P2)P2m1(P1+P2)
m1m2P2P2m1+P1m2
The correct Answer is:A
According to Boyle's law, PV=kT (a constnt)
or PmρkTorρ=pmkT
orρ=PK (wherekTm=Kaconstant)
So, ρ1=P1KandV1m1ρ1=m1P1/K=km1P1
Similarly, V2=Km2P2
Total volume =V1+V2=K(m1P1+m2P2)
Let P be the common pressure and ρ be the common density of mixture. Then.
ρ=m1+m2V1+V2=m1+m2K(m1P1+m2P2)
∴P=Kρ=m1+m2m1P1+m2P2=P1P2(m1+m2)(m1P2+m2P1)
Topper's Solved these Questions
- KINETIC THEORYBOOK - NCERT FINGERTIPSCHAPTER - KINETIC THEORYEXERCISE - NCERT Exemplar
8 Videos
- KINETIC THEORYBOOK - NCERT FINGERTIPSCHAPTER - KINETIC THEORYEXERCISE - Assertion And Reason
10 Videos
- KINETIC THEORYBOOK - NCERT FINGERTIPSCHAPTER - KINETIC THEORYEXERCISE - Specific Heat Capacity
13 Videos
- GRAVITATIONBOOK - NCERT FINGERTIPSCHAPTER - GRAVITATIONEXERCISE - Assertion And Reason
15 Videos
- LAWS OF MOTIONBOOK - NCERT FINGERTIPSCHAPTER - LAWS OF MOTIONEXERCISE - Assertion And Reason
15 Videos
Similar Questions
Two chambers containing m1 and m2 kg of gas at pressure p1 and p2 are later connected. What will be pressure of the mixture.
View SolutionTwo containers of equal volume contain the same gas at pressure P1 and P2 and absolute temperature T1 and T2, respectively. On joining the vessels, the gas reaches a common pressure P and common temperature T. The ratio P/T is equal to
View Solution
Knowledge Check
Two cylinder having m1g and m2g of a gas at pressure P1 and P2 respectively are put in cummunication with each other, temperature remaining constant. The common pressure reached will be
Am1m2P2P2m1+P1m2BP1P2m1P2m1+P1m2Cm1m2(P1+P2)P2m1+P1m2DP1P2(m1+m2)P2m1+P1m20.1 M NaCl and 0.1 M CH3COOH are found to have osmotic pressures of P1 and P2 respectively then what is the correct ststement ?
AP1>P2BP1ifP2CP1ltP2DP1=P2=0 atm.Two containers of equal volume contain the same gas at pressure P1 and P2 and absolute temperature T1 and T2, respectively. On joining the vessels, the gas reaches a common pressure P and common temperature T. The ratio P/T is equal to
AP1T1+P2T2BP1T1+P2T2(T1+T2)2CP1T2+P2T2(T1+T1)2DP12T1+P22T2
Three containes of the same volume contain three different gases. The masses of the molecules are m1,m2 and m3 and the number of molecules in their respective containers are N1,N2 and N3. The gas pressure in the containers are P1,P2 and P3 respectively. All the gases are now mixed and put in one of the containers. The pressure P of mixture will be
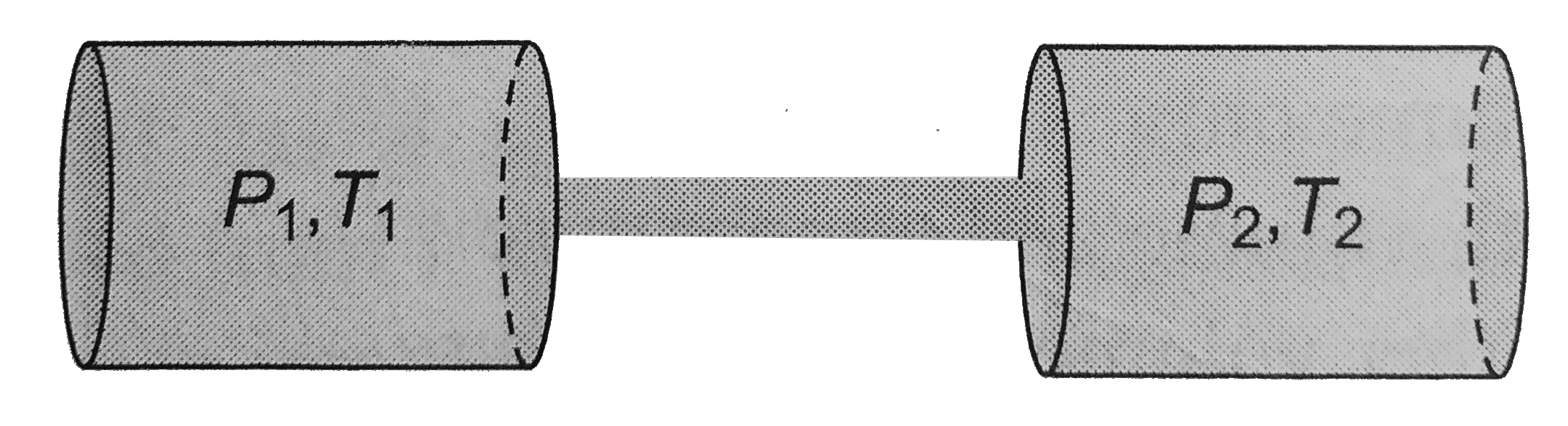
View SolutionTwo identical vessels contain the same gas at pressure P1 and P2 at absolute temperature T1andT2, respectively. On joining the vessels with a small tube as shown in the figure. The gas reaches a common temperature T and a common pressure P. Determine the ratio P/T
View SolutionV vs T curves at constant pressure P1 and P2 for an ideal gas are shown below
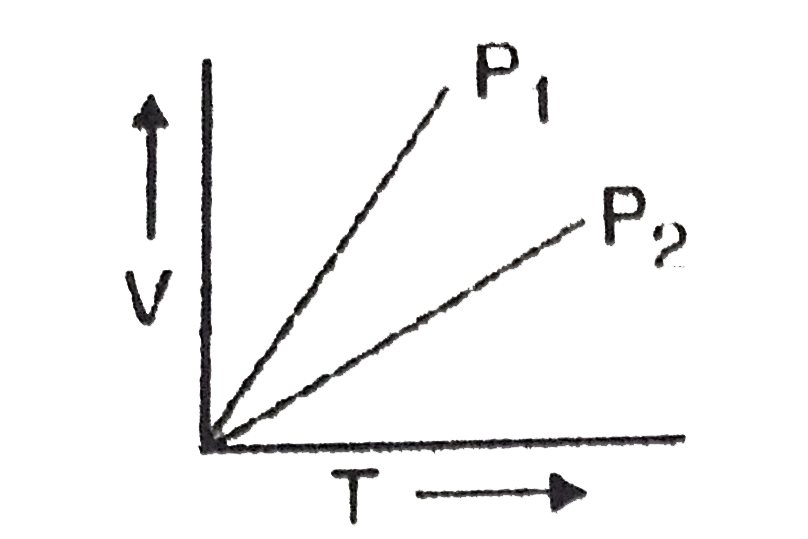
Which is correct ?
View SolutionGaseous system is placed with pressure P1, volume V1 and temperature T1, it has undergone thermodynamic changes where temperature is remaining constant, it is
View SolutionTwo bodies of masses m1andm2 have equal kinetic energies. If P1 and P2 are their respective momentum, then ratio P1:P2 is equal to
View Solution
