The temperature (T) of one mole of an ideal gas varies with its volume (V) as , where are positive constants. The maximum pressure of gas during this process is
The correct Answer is:B
To find the maximum pressure of the gas during the process described by the temperature equation T=−αV3+βV2, we can follow these steps:
Step 1: Relate Pressure, Volume, and Temperature
We know from the ideal gas law that:
PV=nRT
For one mole of gas (n=1), this simplifies to:
P=RTV
Substituting the given temperature equation into this, we have:
P=R(−αV3+βV2)V
This simplifies to:
P=R(−αV2+βV)
Step 2: Differentiate Pressure with Respect to Volume
To find the maximum pressure, we need to differentiate P with respect to V and set the derivative equal to zero:
dPdV=R(−2αV+β)
Setting this equal to zero gives us:
−2αV+β=0
Solving for V yields:
V=β2α
Step 3: Substitute Back to Find Maximum Pressure
Now, we substitute this value of V back into the pressure equation to find the maximum pressure:
P=R(−α(β2α)2+β(β2α))
Calculating each term:
P=R(−αβ24α2+β22α)
This simplifies to:
P=R(−β24α+2β24α)
Combining the terms gives:
P=R(β24α)
Final Result
Thus, the maximum pressure of the gas is:
Pmax=Rβ24α
We know from the ideal gas law that:
PV=nRT
For one mole of gas (n=1), this simplifies to:
P=RTV
Substituting the given temperature equation into this, we have:
P=R(−αV3+βV2)V
This simplifies to:
P=R(−αV2+βV)
To find the maximum pressure, we need to differentiate P with respect to V and set the derivative equal to zero:
dPdV=R(−2αV+β)
Setting this equal to zero gives us:
−2αV+β=0
Solving for V yields:
V=β2α
Now, we substitute this value of V back into the pressure equation to find the maximum pressure:
P=R(−α(β2α)2+β(β2α))
Calculating each term:
P=R(−αβ24α2+β22α)
This simplifies to:
P=R(−β24α+2β24α)
Combining the terms gives:
P=R(β24α)
Thus, the maximum pressure of the gas is:
Pmax=Rβ24α
Topper's Solved these Questions
- KINETIC THEORYBook:AAKASH INSTITUTEChapter:KINETIC THEORYExercise:EXERCISE (ASSIGNMENT) SECTION - C Previous Years Questions
Explore 21 Videos
- KINETIC THEORYBook:AAKASH INSTITUTEChapter:KINETIC THEORYExercise:EXERCISE (ASSIGNMENT) SECTION - D Assertion - Reason Type Questions
Explore 10 Videos
- KINETIC THEORYBook:AAKASH INSTITUTEChapter:KINETIC THEORYExercise:EXERCISE (ASSIGNMENT) SECTION - A Objective Type Questions
Explore 30 Videos
- GRAVITATION Book:AAKASH INSTITUTEChapter:GRAVITATION Exercise:ASSIGNMENT SECTION - D (ASSERTION-REASON TYPE QUESTIONS)
Explore 16 Videos
- LAWS OF MOTIONBook:AAKASH INSTITUTEChapter:LAWS OF MOTIONExercise:Assignment (SECTION-D) (Assertion-Reason Type Questions)
Explore 15 Videos
Similar Questions
Find the maximum attainable temperature of ideal gas in each of the following process :
(a) p=p0−αV2 ,
(b) p=p0e−βv,
where p0,α and β are positive constants, and V is the volume of one mole of gas.
View SolutionThe relation between the internal energy (U) versus temeperature (T) for a 3 mole ideal gas in an isochoric process is given as U=α+βT where α and β are constant. If the specific heat capacity of the gas for above process is βm find the value of m.
View Solution
Knowledge Check
One mole of an ideal gas undergoes a process
P=P0−αV2
where α and P0 are positive constant and V is the volume of one mole of gas
Q. When temperature is maximum, volume isA23P0BP0CP03D32P0One mole of an ideal gas undergoes a process
P=P0−αV2
where α and P0 are positive constant and V is the volume of one mole of gas
Q. When temperature is maximum, volume isA√P03αB√P0αC√P02αD√P0αOne mole of an ideal gas undergoes a process
P=P0−αV2
where α and P0 are positive constant and V is the volume of one mole of gas
Q. The maximum attainable temperature isAP0R√P03αB23P0R√P03αC√P03αD√P0R
Find the minimum attainable pressure of an ideal gas in the process T=T0+αV2, Where T0 and α are positive constant and V is the volume of one mole of gas. Draw the approximate T−V plot of this process.
View SolutionThe displacement x of a particle varies with time t as x=ae−αt+beβt. Where a,b,α and β positive constant.
The velocity of the particle will.
View SolutionOne mole of an ideal gas undergoes a process in which T=T0+aV3, where T0 and a are positive constants and V is molar volume. The volume for which pressure with be minimum is
View SolutionOne mole of an ideal monotomic gas undergoes a linear process from A to B in which its pressure p and its volume V change as shown in figure.
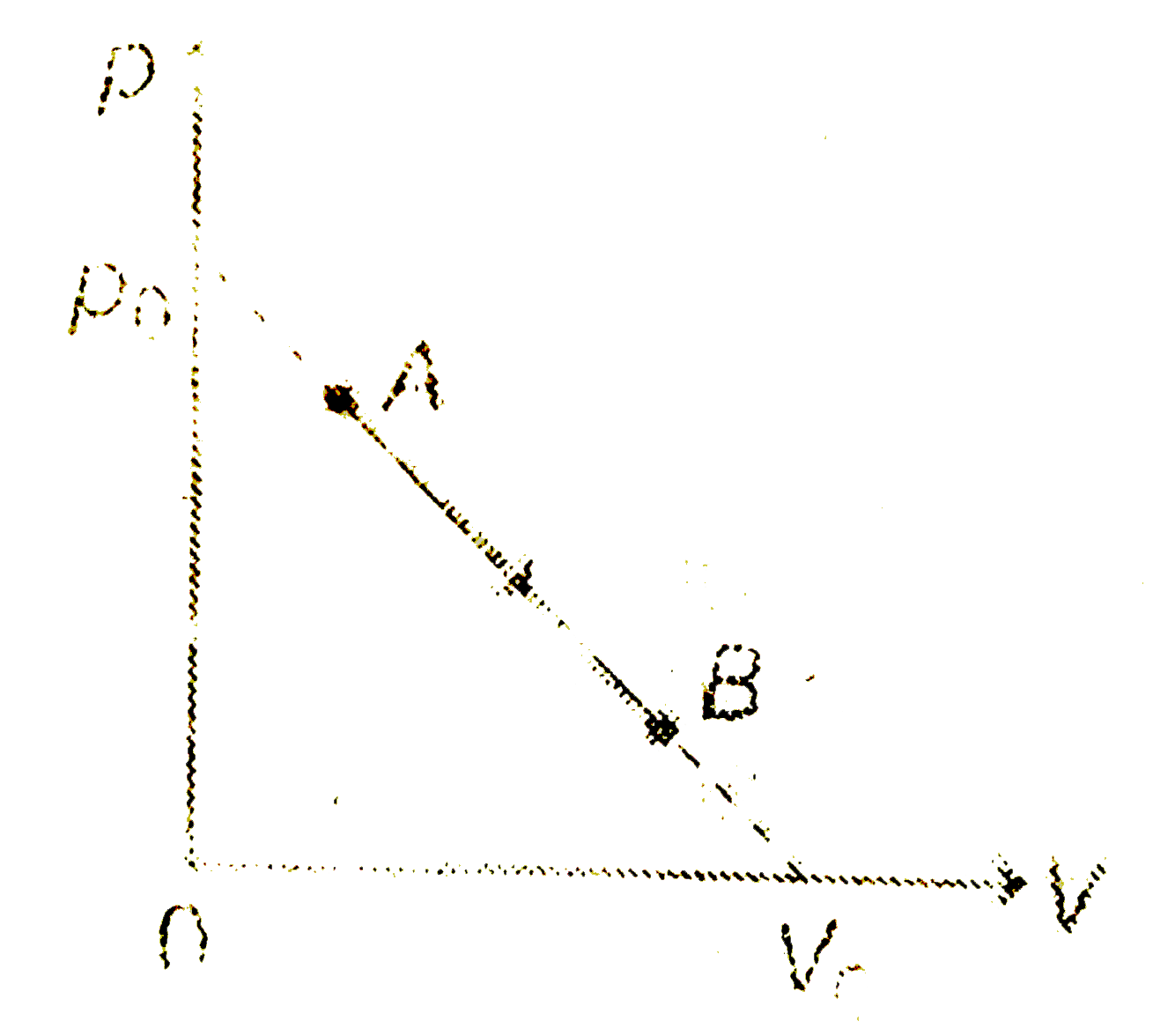
The maximum temperature of the gas during this process is
View SolutionFind the minimum attainabel pressure of one mole of an ideal gas. If during its expansion its temprature and volume are related as T=T0+αV2 where T0&α are positive constants
View Solution