From a uniform circular disc of mass M and radius R a small circular disc of radius R/2 is removed in such a way that both have a common tangent. Find the distance of centre of mass of remaining part from the centre of original disc.
To solve the problem, we need to find the distance of the center of mass of the remaining part of the disc after removing a smaller disc. Here’s a step-by-step solution:
Step 1: Understand the Geometry
We have a large disc of radius R and mass M. A smaller disc of radius R2 is removed from it. The center of the smaller disc is located at a distance R from the center of the larger disc along the radius.
Step 2: Calculate the Mass of the Smaller Disc
The mass of the smaller disc can be calculated using the area ratio since both discs are uniform. The area of the larger disc is πR2 and the area of the smaller disc is π(R2)2=πR24.
Thus, the mass of the smaller disc m is:
m=M⋅Area of smaller discArea of larger disc=M⋅πR24πR2=M4
Step 3: Determine the Center of Mass of the Remaining Part
After removing the smaller disc, the mass of the remaining part is:
M′=M−m=M−M4=3M4
Step 4: Set Up the Coordinate System
Let’s set the center of the larger disc as the origin (0,0). The center of the smaller disc, after being removed, is at the point (R,0).
Step 5: Calculate the Center of Mass of the Remaining Part
The center of mass xcm of the remaining part can be calculated using the formula:
xcm=M⋅0−m⋅RM−m
Substituting the values we have:
xcm=M⋅0−M4⋅RM−M4=−M4⋅R3M4=−R3
Step 6: Interpret the Result
The negative sign indicates that the center of mass of the remaining part is located to the left of the center of the original disc. Therefore, the distance of the center of mass of the remaining part from the center of the original disc is:
Distance=R3
Final Answer
The distance of the center of mass of the remaining part from the center of the original disc is R3.
---
We have a large disc of radius R and mass M. A smaller disc of radius R2 is removed from it. The center of the smaller disc is located at a distance R from the center of the larger disc along the radius.
The mass of the smaller disc can be calculated using the area ratio since both discs are uniform. The area of the larger disc is πR2 and the area of the smaller disc is π(R2)2=πR24.
m=M⋅Area of smaller discArea of larger disc=M⋅πR24πR2=M4
After removing the smaller disc, the mass of the remaining part is:
M′=M−m=M−M4=3M4
Let’s set the center of the larger disc as the origin (0,0). The center of the smaller disc, after being removed, is at the point (R,0).
The center of mass xcm of the remaining part can be calculated using the formula:
xcm=M⋅0−m⋅RM−m
Substituting the values we have:
xcm=M⋅0−M4⋅RM−M4=−M4⋅R3M4=−R3
The negative sign indicates that the center of mass of the remaining part is located to the left of the center of the original disc. Therefore, the distance of the center of mass of the remaining part from the center of the original disc is:
Distance=R3
The distance of the center of mass of the remaining part from the center of the original disc is R3.
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTECHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - J) Aakash Challengers Questions
13 Videos
- SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSBOOK - AAKASH INSTITUTECHAPTER - SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSEXERCISE - Assignment (Section-D (Assertion and reason))
5 Videos
- TEST 1BOOK - AAKASH INSTITUTECHAPTER - TEST 1EXERCISE - EXERCISE
9 Videos
Similar Questions
From a uniform circular disc of radius R, a circular disc of radius R/6 and having centre at a distance R/2 from the centre of the disc is removed. Determine the centre of mass of remaining portion of the disc.
View SolutionA disc of radius a2 is cut out from a uniform disc of radius a as shown in figure . Find the X Coordinate of centre of mass of remaining portion
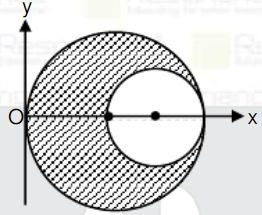
View Solution
Knowledge Check
A circular hole is cut from a disc of radius 6 cm in such a way that the radius of the hole is 1 cm and the centre of 3 cm from the centre of the disc. The distance of the centre of mass of the remaining part from the centre of the original disc is
A3/35 cmB1/35 cmC3/10 cmD7/35 cmFrom a circular disc of radius R, a triangular portion is cut (sec figure). The distance of the centre of mass of the remainder from the centre of the disc is −
 A4R3(π−2)B2R3(π−2)C5R7(π−2)DR3(π−1)
A4R3(π−2)B2R3(π−2)C5R7(π−2)DR3(π−1)From a circular disc of radius R, a square is cut out with a radius as its diagonal. The center of mass of remaining portion is at a distance from the center)
AR4π−2BR2πCRπ−2DR2π−2
From a semicircular disc of radius r1, another semicircular disc of radius r2 is removed. Find the c.m. of the remaining position.
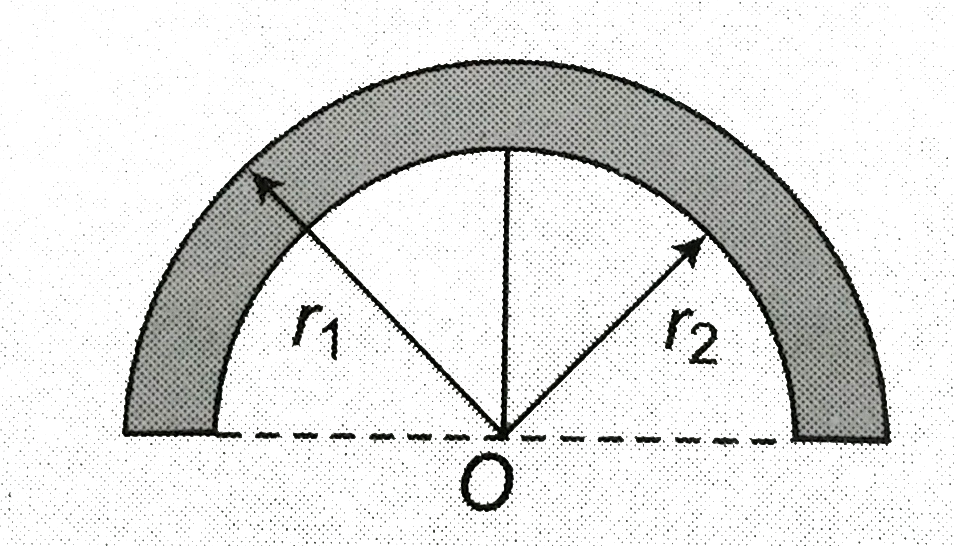
View SolutionFron a sqaure plate of side a,a quarter circular disc of radius a is removed as shown in figure. Find the coordinates of centre of mass of the remaining part.
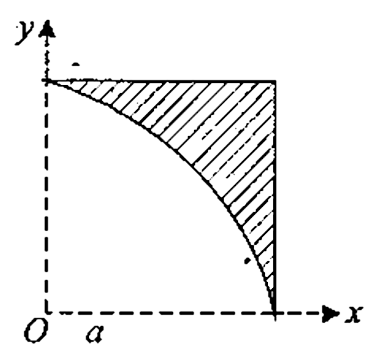
View SolutionGiven a uniform disc of mass M and radius R. A small disc of radius R/2 is cut from this disc in such a way that the distance between the centres of the two discs is R/2. Find the moment of inertia of the remaining disc about a diameter of the original disc perpendicular to the line connecting the centres of the two discs
View SolutionA circular disc of radius R is removed from a bigger circular disc of radius 2R such that the circumference of the discs coincoid . The centre of mass of the new disc is αR from the centre of the bigger disc . the value of α is
View SolutionA solid toy is in the form of a hemisphere surmounted by a right circular cone of same radius. The height of the cone is 10 Cm and the radius of the base is 7 cm. Determine the volume of the toy. Also find the area of the coloured sheet required to cover the toy. (Use π=22/7 and √149=12.2)
View Solution
