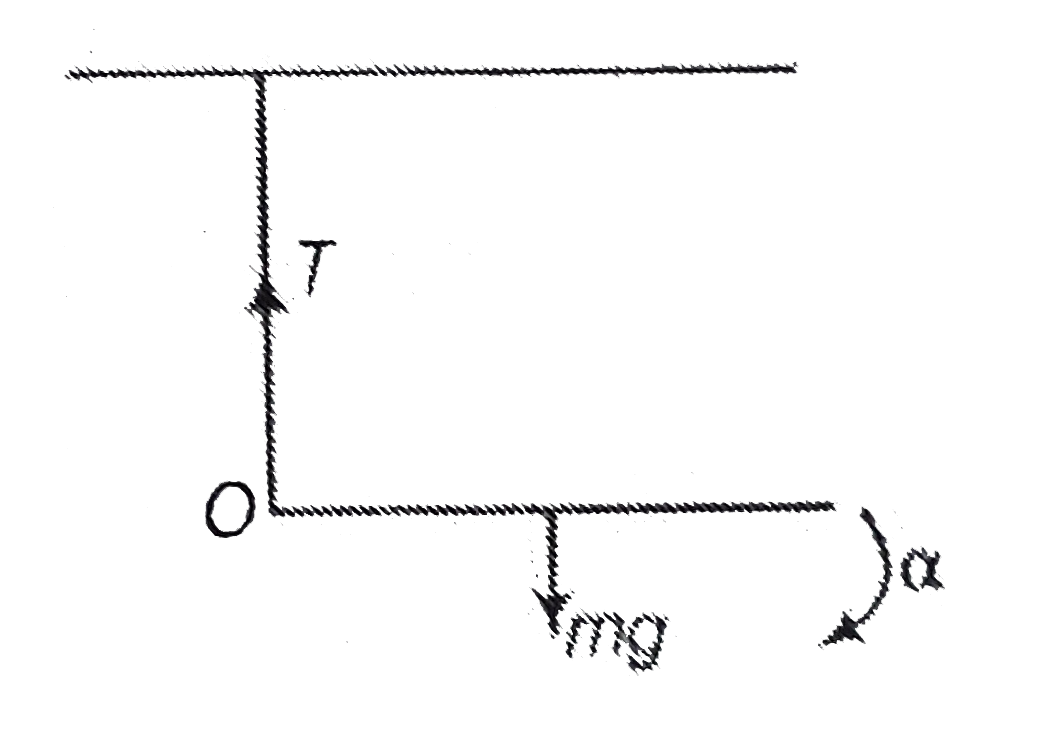
A cylinder of mass m is suspended through two strings wrapped around it as shwon in figure . Find (a). the tension T in the string and (b). the speed o the cylinder as it falls through a distance h.
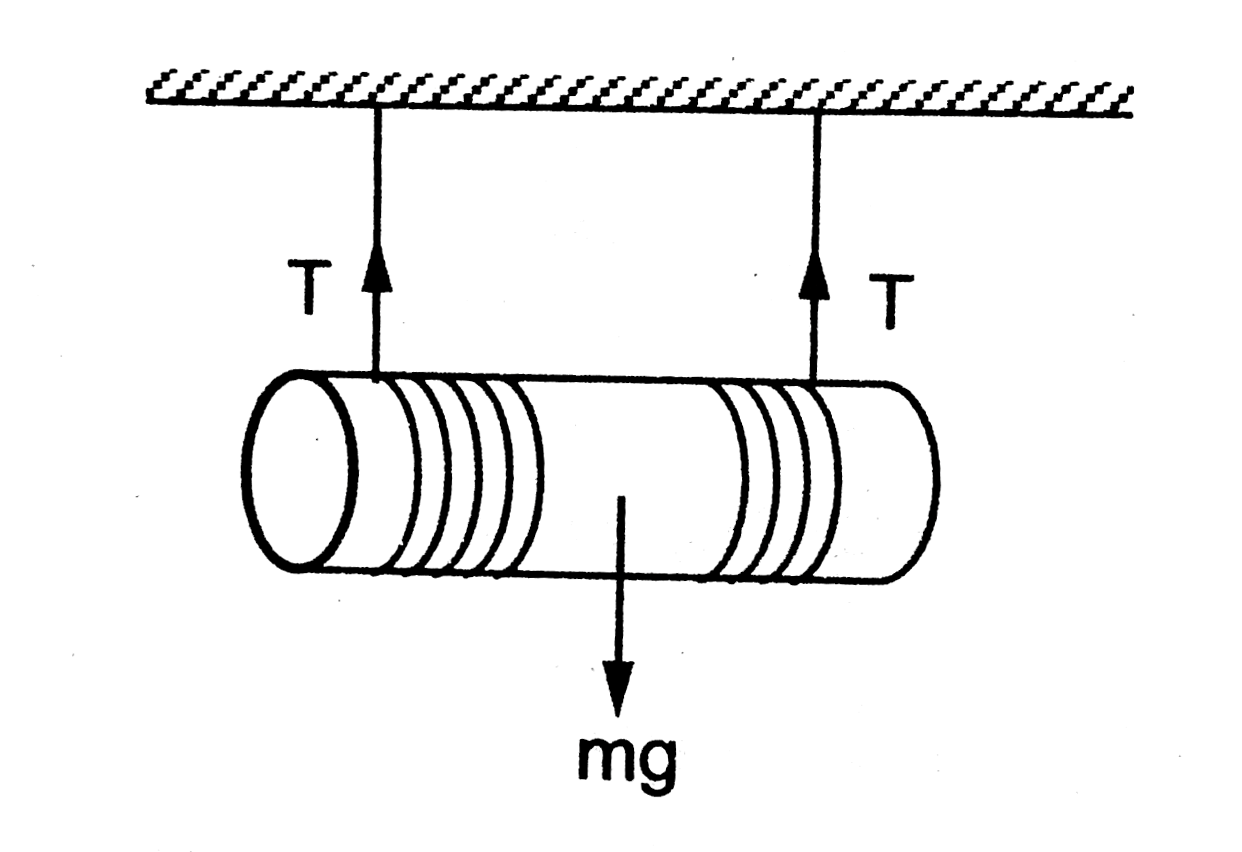
For the linear motion of the centre of mass.
Net force =mg−2T=ma,
[where a is the acceleration of the CM] ….(i)
For the rotational motion about the CM, Net torque =2Tr=lcmar
or 2T=lcmar2 ...(ii)
From (i) and (ii), we get
a=mgm+lcmr2 ....(A)
From (ii), T=lcm2r2⎛⎜⎝mgm+lcmr2⎞⎟⎠ ....(B)
Putting lcm=12mr2, we get a=23g and T=mg6
v2=2ah=2(23g)h or v=√4gh3
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTECHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Illustration
4 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTECHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - A) Objective Type Questions (One option is correct)
62 Videos
- SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSBOOK - AAKASH INSTITUTECHAPTER - SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSEXERCISE - Assignment (Section-D (Assertion and reason))
5 Videos
- TEST 1BOOK - AAKASH INSTITUTECHAPTER - TEST 1EXERCISE - EXERCISE
9 Videos
Similar Questions
Knowledge Check
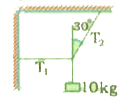
A block of mass 10 kg is suspended by three strings as shown in figure . Tension T2 is
 A100NB100√3NC√3×100ND50√3N
A100NB100√3NC√3×100ND50√3NA uniform rod of length l and mass M is suspended on two vertical inextensible string as shown in figure. Then tension T in the left string at the instant, when right string snaps is?
 AT=MgBT=Mg2CT=Mg4DT=zero
AT=MgBT=Mg2CT=Mg4DT=zeroA particle of mass m is tied to a string of length L and whirled into a horizontal plan. If tension in the string is T then the speed of the particle will be :
A√TlmB√2TlmC√3TlmD√Tml
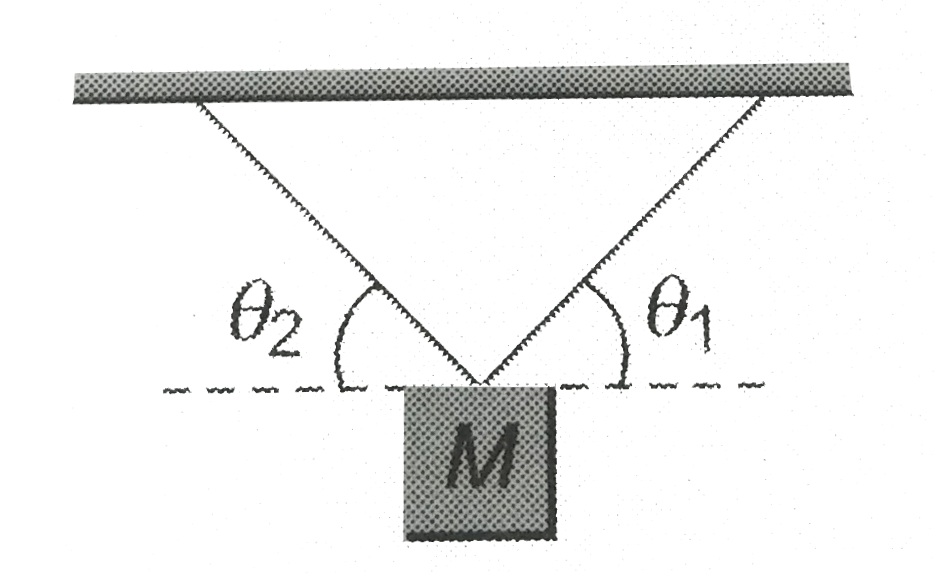
A block of mass M si suspended by two strings angles θ1 and θ2 with the horizontal. Find the tensions in the strings.
View SolutionA cylinder of mass m suspended by two strings wrapped around the cylinder one near each end, the free ends of the string being attached to hooks on the ceiling, such that the length of the cylinder is horizontal. From the position of rest , the cylinder is allowed to roll down as suspension strings unwind , calculate
(a) The downward linear acceleration of the cylinder
(b) The tension in the strings
(c) The time dependence of the instantaneous power developed by gravity
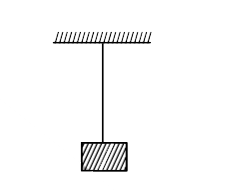
View SolutionA 'block' of mass 10 kg is suspended with string as shown in figure. Find tension in the string. (g=10m/s2)
View SolutionA block of mass 30 kg is suspended by three string as shown in fig, Find the tension in each string.

View SolutionA uniform rod of length l and mass m is suspended by two vertical inextensible strings as shwon in figure. Then, tension in the left string when right string snape, si
View Solution