A body a of mass m slides on plane inclined at angle θ0 to the horizontal and μ1 is the coefficient of friction between A and the plane A is connected by a light string passing over a frictionless pulley to another body B also of mass m slidding on a frictionless plane inclined at angle θ2 to the horizontal Which of the following statements are ture ? 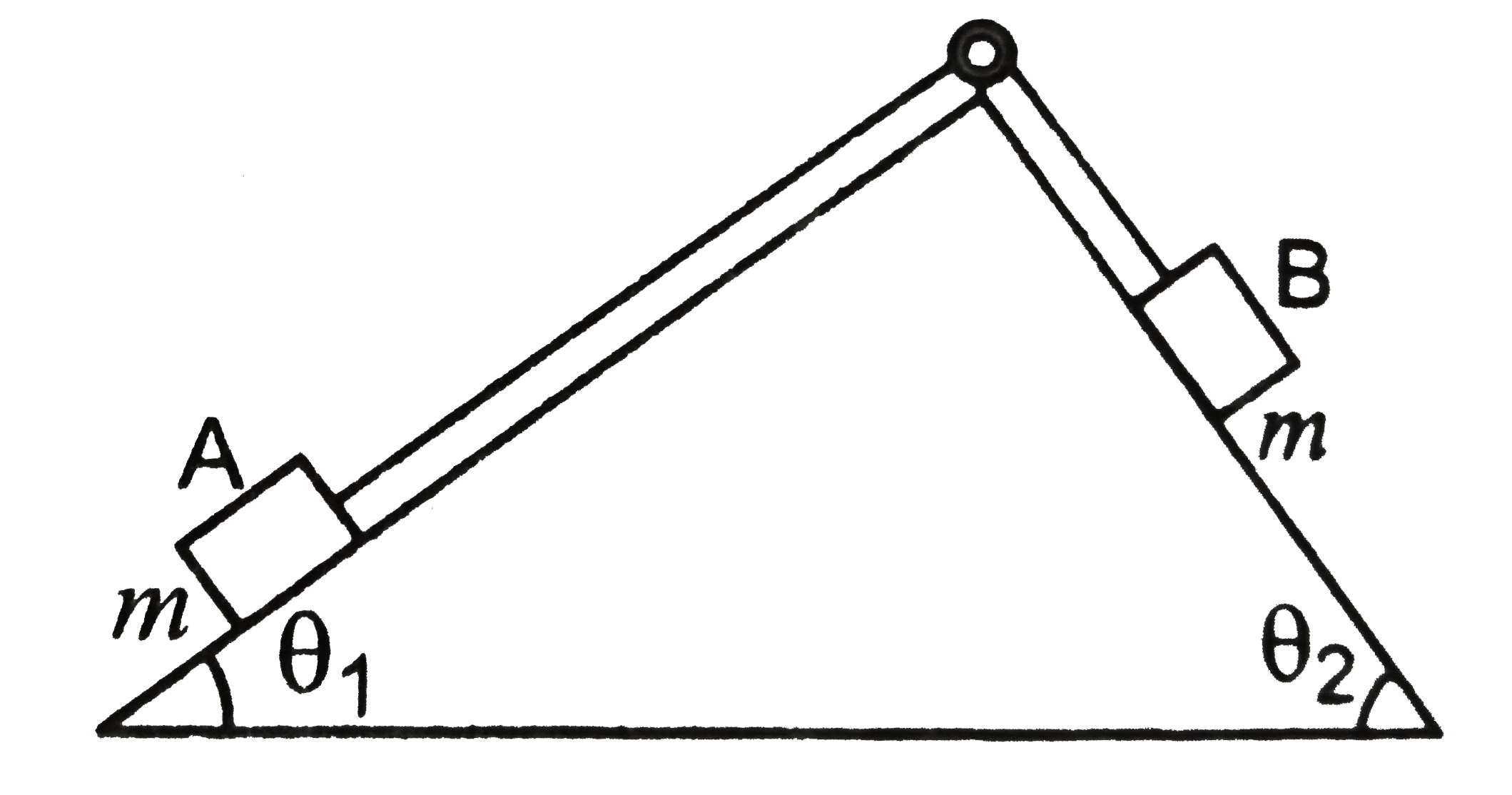 .
.
A will never move up the plane
A will just start moving up the plane when μ=sinθ2−sinθ1cosθ1
For A to move up the plane θ2 , must always be greater than θ1
B will always slide down with constant speed
The correct Answer is:B, C
Let A moves up the plane frictional force on A will be downward as shown .
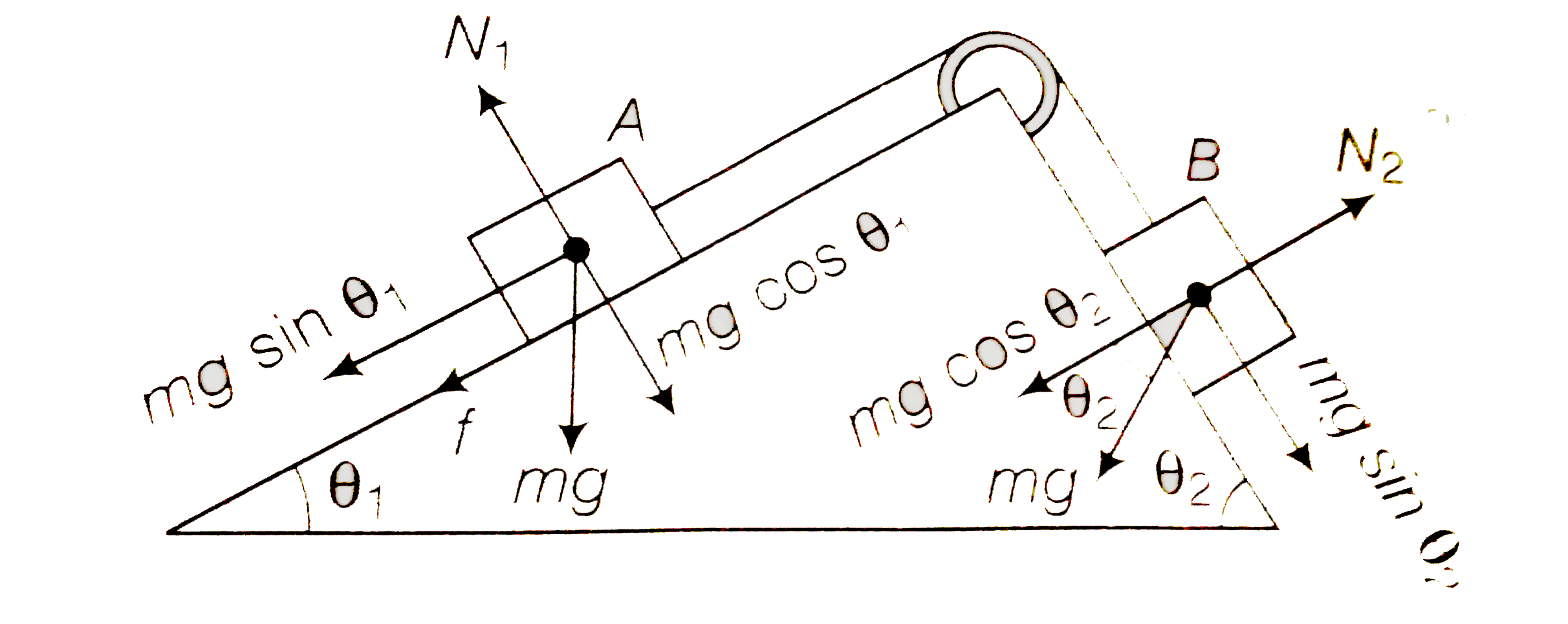
When A just starts moving up
mg sin θ1+f=mgsinθ2
⇒ mgsinθ1+μmgcosθ1=mgsinθ2
⇒ =sinθ2−sinθ1cosθ1
When A moves upwards
f = mg sin θ2−mgsinθ1>0
⇒ sinθ2>sinθ1⇒θ2>θ1
Related Playlists
- BOOK - NCERT EXEMPLARCHAPTER - LAWS OF MOTIONEXERCISE - Very short answer type Questions12videos
LAWS OF MOTION
- BOOK - NCERT EXEMPLARCHAPTER - LAWS OF MOTIONEXERCISE - Short answer type questions6videos
LAWS OF MOTION
- BOOK - NCERT EXEMPLARCHAPTER - LAWS OF MOTIONEXERCISE - Long answer Type Questions9videos
LAWS OF MOTION
- BOOK - NCERT EXEMPLARCHAPTER - KINETIC THEORYEXERCISE - Multiple Choice Questions (MCQs)31videos
KINETIC THEORY
- BOOK - NCERT EXEMPLARCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Long Answer Type Questions3videos
MECHANICAL PROPERTIES OF FLUIDS
Similar Questions
A block of mass m slides down an inclined plane which makes an angle θ with the horizontal. The coefficient of friction between the block and the plane is μ. The force exerted by the block on the plane is
View SolutionA body of mass m slides down an inclined plane making an angle of 45∘ with the horizontal. If the coefficient of friction between the body and the plane be 0.3, the acceleration of the body is approximately equal to :
View SolutionA block of mass m is placed at rest on an inclination θ to the horizontal.If the coefficient of friction between the block and the plane is μ, then the total force the inclined plane exerts on the block is
View SolutionA body is sliding down an inclined plane forming an angle 30∘ with the horizontal. If the coefficient of friction is 0.3 then acceleration of the body is
View SolutionA body of mass m slides down a rough plane of inclination α . If μ is the coefficient of friction, then acceleration of the body will be
View SolutionA body of mass m slides down a rough plane of inclination α . If μ is the coefficient of friction, then acceleration of the body will be
View SolutionA block of mass m is placed on a rough plane inclined at an angle θ with the horizontal. The coefficient of friction between the block and inclined plane is μ . Ifθ<tan−1(μ) , then net contact force exerted by the plane on the block is :
View SolutionA body takes just twice the time as long to slide down a plane inclined at 30∘ to the horizontal as if the plane were frictionless. The coefficient of friction between the body and the plane is
View SolutionA small mass slides down an inclined plane of inclination θ the horizontal the coefficient of friction is m=μ0x, where x is the distance by the mass before it stops is .
View SolutionA block of mass m slides down an inclined plane of inclination θ with uniform speed. The coefficient of friction between the block and the plane is μ. The contact force between the block and the plane is
View Solution


