In a square ABCD, AB=(2x+3) cm and BC=(3x-5) cm . Then , the value of x is
4
5
6
8
The correct Answer is:D
To solve the problem, we need to find the value of x in the given square ABCD where the lengths of sides AB and BC are given as AB=(2x+3) cm and BC=(3x−5) cm.
1. Understand the properties of a square: In a square, all sides are equal. Therefore, we can set the lengths of sides AB and BC equal to each other.
AB=BC
2. Set up the equation: Substitute the expressions for AB and BC into the equation.
2x+3=3x−5
3. Rearrange the equation: To isolate x, we will first move all terms involving x to one side and constant terms to the other side.
2x+3+5=3x
This simplifies to:
2x+8=3x
4. Isolate x: Now, subtract 2x from both sides of the equation.
8=3x−2x
This simplifies to:
8=x
5. Conclusion: Thus, the value of x is:
x=8
Final Answer:
The value of x is 8.
---
The value of x is 8.
Topper's Solved these Questions
- BOOK - RS AGGARWALCHAPTER - PARALLELOGRAMSEXERCISE - Exercise 16A28videos
PARALLELOGRAMS
- BOOK - RS AGGARWALCHAPTER - OPERATIONS ON ALGEBRAIC EXPRESSIONSEXERCISE - Exercise 6E (Tick the correct answer in each of the following:)19videos
OPERATIONS ON ALGEBRAIC EXPRESSIONS
- BOOK - RS AGGARWALCHAPTER - PERCENTAGEEXERCISE - TEST PAPER -9 ( TRUE AND FALSE )4videos
PERCENTAGE
Similar Questions
Knowledge Check
- Question 1 - Select One
ABCD is a square with AB =(x = 16) cm and BC = (3x) cm . The perimeter (in cm ) is
A16B24C32D96 - Question 2 - Select One
ABCD is a square with AB = (x + 6) cm and BC= (3x) cm. The perimeter (in cm) is
A16B24C32D36 - Question 3 - Select One
ABCD is a trapezium in which AB parallel to DC and its diagonals intersect at P. If AP = (3x - 1) cm, PC = (5x - 3) cm, BP = (2x + 1) cm and PD = (6x - 5) cm, then the length of DB is:
ABCD एक समलम्ब है जिसमें AB, DC के समानांतर है तथा इसके विकर्ण P पर एक दूसरे को काठते हैं | यदि AP = (3x - 1) सेमी, PC = (5x - 3) सेमी, BP = (2x + 1) सेमी तथा PD = (6x - 5) सेमी है, तो DB की लंबाई क्या होगी ?A14 cmB12 cmC10 cmD16 cm
ABCD is a trapezium in which AB parallel to DC and its diagonals intersect at P. If AP = (3x - 1) cm, PC = (5x - 3) cm, BP = (2x + 1) cm and PD = (6x - 5) cm, then the length of DB is:
ABCD एक समलम्ब है जिसमें AB, DC के समानांतर है तथा इसके विकर्ण P पर एक दूसरे को काठते हैं | यदि AP = (3x - 1) सेमी, PC = (5x - 3) सेमी, BP = (2x + 1) सेमी तथा PD = (6x - 5) सेमी है, तो DB की लंबाई क्या होगी ?View SolutionIn ΔABC, right angled at B, if AB=12cm, BC= x and AC=13cm, then the value of x is
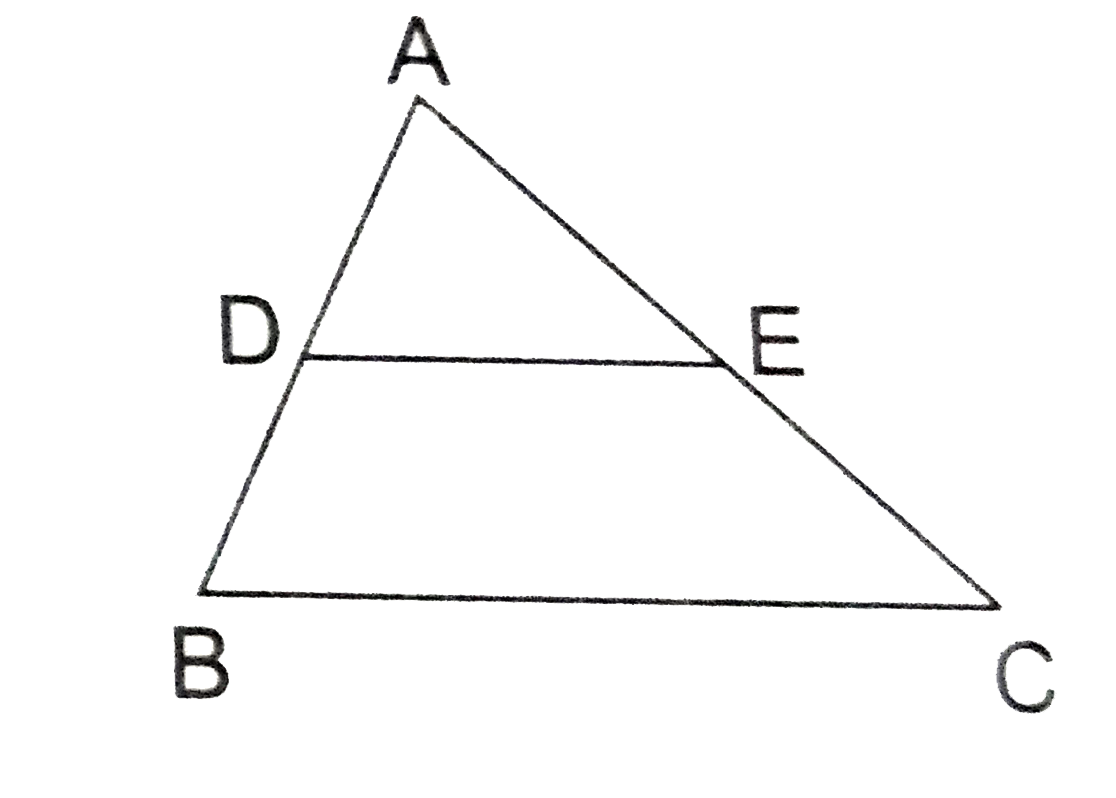
View SolutionIn the given figure, in ΔABC,DE∣∣BC so that AD=(4x−3)cm,AE=(8x−7)cm,BD=(3x−1)cmandCE=(5x−3)cm . Find the value of x.
View SolutionABCD is a square with AB = (x + 16) cm and BC = ( 3 x) cm. The perimeter (in cm) of the square is
View SolutionIf D and E are points on the sides AB and AC respectively of a triangle ABC such that DE || BC. If AD =x cm, DB =(x-3) cm, AE = ( x+ 3 ) cm and EC = ( x -2) cm, what is the value (in cm. ) of x ?
View Solution