A diagonal of a quadrilateral is 26 cm and the perpendiculars drawn to it from the opposite vertices are 12.8 cm and 11.2 cm. Find the area of the quadrilateral.
The correct Answer is:312cm2
To find the area of the quadrilateral, we can use the formula for the area of a quadrilateral when the lengths of the diagonals and the perpendiculars from the opposite vertices to the diagonal are known. The area A can be calculated using the formula:
A=12×d×(h1+h2)
where:
- d is the length of the diagonal,
- h1 and h2 are the lengths of the perpendiculars from the opposite vertices to the diagonal.
1. Identify the given values:
- Length of the diagonal d=26 cm
- Length of the first perpendicular h1=12.8 cm
- Length of the second perpendicular h2=11.2 cm
2. Substitute the values into the area formula:
A=12×26×(12.8+11.2)
3. Calculate the sum of the perpendiculars:
h1+h2=12.8+11.2=24 cm
4. Now substitute this sum back into the area formula:
A=12×26×24
5. Calculate 12×26:
12×26=13
6. Now multiply by 24:
A=13×24=312 cm2
Final Answer:
The area of the quadrilateral is 312 cm2.
- d is the length of the diagonal,
- h1 and h2 are the lengths of the perpendiculars from the opposite vertices to the diagonal.
- Length of the diagonal d=26 cm
- Length of the first perpendicular h1=12.8 cm
- Length of the second perpendicular h2=11.2 cm
A=12×26×(12.8+11.2)
h1+h2=12.8+11.2=24 cm
A=12×26×24
12×26=13
A=13×24=312 cm2
The area of the quadrilateral is 312 cm2.
Topper's Solved these Questions
- MENSURATIONBOOK - RS AGGARWALCHAPTER - MENSURATIONEXERCISE - EXERCISE 20E
21 Videos
- MENSURATIONBOOK - RS AGGARWALCHAPTER - MENSURATIONEXERCISE - EXERCISE 20F
16 Videos
- MENSURATIONBOOK - RS AGGARWALCHAPTER - MENSURATIONEXERCISE - EXERCISE 20C
16 Videos
- LINES AND ANGLESBOOK - RS AGGARWALCHAPTER - LINES AND ANGLESEXERCISE - Exercise 13
11 Videos
- PERCENTAGE BOOK - RS AGGARWALCHAPTER - PERCENTAGE EXERCISE - TEST PAPER
16 Videos
Similar Questions
One diagonal of a quadrilateral is 20 m long and the perpendiculars to it from the opposite vertices are 8.5 m and 11 m. Find the areaof the quadrilateral.
View SolutionA diagonal of a quadrilateral is 30 cm in length and the lengths of perpendiculars to it from the opposite vertices are 6.8 cm and 9.6 cm. Find the area of the quadrilateral.
View Solution
Knowledge Check
The diagonal of a quadrilateral is 20 cm in length and the lengths of perpendiculars on it from the opposite vertices are 8.5 cm and 11.5 cm. The area of the quadrilateral is
A400cm2B200cm2C300cm2D240cm2The diagonal of a quadrilateral shaped field is 24 m and the perpendiculars dropped on its from the remaining opposite vertices are 8m and 13m. The area of the field is
A252m2B156m2C96m2D1152m2ABCD is a quadrilateral in which BD = 40 cm. The lengths of the perpendiculars drawn from the opposite vertices on BD are 16 cm and 12 cm. The area of the quadrilateral (in cm2) is
A560B530C800D500
The diagonal of a quadrilateral shaped field is 24 m and the perpendiculars dropped on it from the remaining opposite vertices are 8 m and 13 m. Find the area of the field.
View SolutionThe diagonal of a quadrilateral shaped field is 24 m and the perpendiculars dropped on it from the remaining opposite vertices are 8 m and 13 m. Find the area of the field.
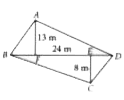
View SolutionThe area of a quadrilateral is 360m2 and the perpendiculars drawn to one of the diagonal from the opposite vertices are 10m and 8m. Find the length of the diagonal.
View SolutionA diagonal of a quadrilateral is 30.5m long. The perpendiculars to the diagonal from the opposite vertices are of lengths 8.5m and 7.5m.Find the area of the quadrilateral
View SolutionABCD is a quadrilateral in which BD = 40 cm. The lengths of the perpendiculars drawn from the opposite vertices on BD are 16 cm and 12 cm . The area of the quadrilateral (in cm ) is
View Solution
