A square has
one line of symmetry
two lines of symmetry
three lines of symmetry
four lines of symmetry
The correct Answer is:D
To determine how many lines of symmetry a square has, we can follow these steps:
1. Understand the concept of lines of symmetry: A line of symmetry is a line that divides a shape into two identical halves. Each half is a mirror image of the other.
2. Draw a square: Start by sketching a square. A square has four equal sides and four right angles.
3. Identify the lines of symmetry:
- Vertical line: Draw a vertical line down the center of the square. This line divides the square into two equal rectangles.
- Horizontal line: Draw a horizontal line across the center of the square. This line also divides the square into two equal rectangles.
- Diagonal line (top left to bottom right): Draw a diagonal line from the top left corner to the bottom right corner. This line divides the square into two equal triangles.
- Diagonal line (top right to bottom left): Draw a diagonal line from the top right corner to the bottom left corner. This line also divides the square into two equal triangles.
4. Count the lines of symmetry: After drawing the lines, you will see that there are four distinct lines of symmetry in total.
5. Choose the correct option: Since we have identified four lines of symmetry, we can conclude that the answer corresponds to option 4.
Final Answer:
Therefore, a square has 4 lines of symmetry, which corresponds to option 4.
---
- Vertical line: Draw a vertical line down the center of the square. This line divides the square into two equal rectangles.
- Horizontal line: Draw a horizontal line across the center of the square. This line also divides the square into two equal rectangles.
- Diagonal line (top left to bottom right): Draw a diagonal line from the top left corner to the bottom right corner. This line divides the square into two equal triangles.
- Diagonal line (top right to bottom left): Draw a diagonal line from the top right corner to the bottom left corner. This line also divides the square into two equal triangles.
Therefore, a square has 4 lines of symmetry, which corresponds to option 4.
Topper's Solved these Questions
- REFLECTION AND ROTATIONAL SYMMETRYBook:RS AGGARWALChapter:REFLECTION AND ROTATIONAL SYMMETRYExercise:Exercise A(True / False)
Explore 7 Videos
- REFLECTION AND ROTATIONAL SYMMETRYBook:RS AGGARWALChapter:REFLECTION AND ROTATIONAL SYMMETRYExercise:Exercise B
Explore 12 Videos
- REFLECTION AND ROTATIONAL SYMMETRYBook:RS AGGARWALChapter:REFLECTION AND ROTATIONAL SYMMETRYExercise:Exercise B
Explore 12 Videos
- RATIONAL NUMBERSBook:RS AGGARWALChapter:RATIONAL NUMBERSExercise:TEST PAPER -(4)
Explore 17 Videos
- SIMPLE INTEREST Book:RS AGGARWALChapter:SIMPLE INTEREST Exercise:test perper 12 D
Explore 1 Video
Similar Questions
Knowledge Check
A regular octagon is formed by cutting congruent isosceles right-angled triangles from the corners of a square. If the square has side-length 1, the side-length of the octagon is-
A√2−12B√2−1C√5−14D√5−13In Fig. there is a uniform conducting structure in which each small square has side a. The structure is kept in a uniform magnetic field B. Then the magnetic force on the structure will be
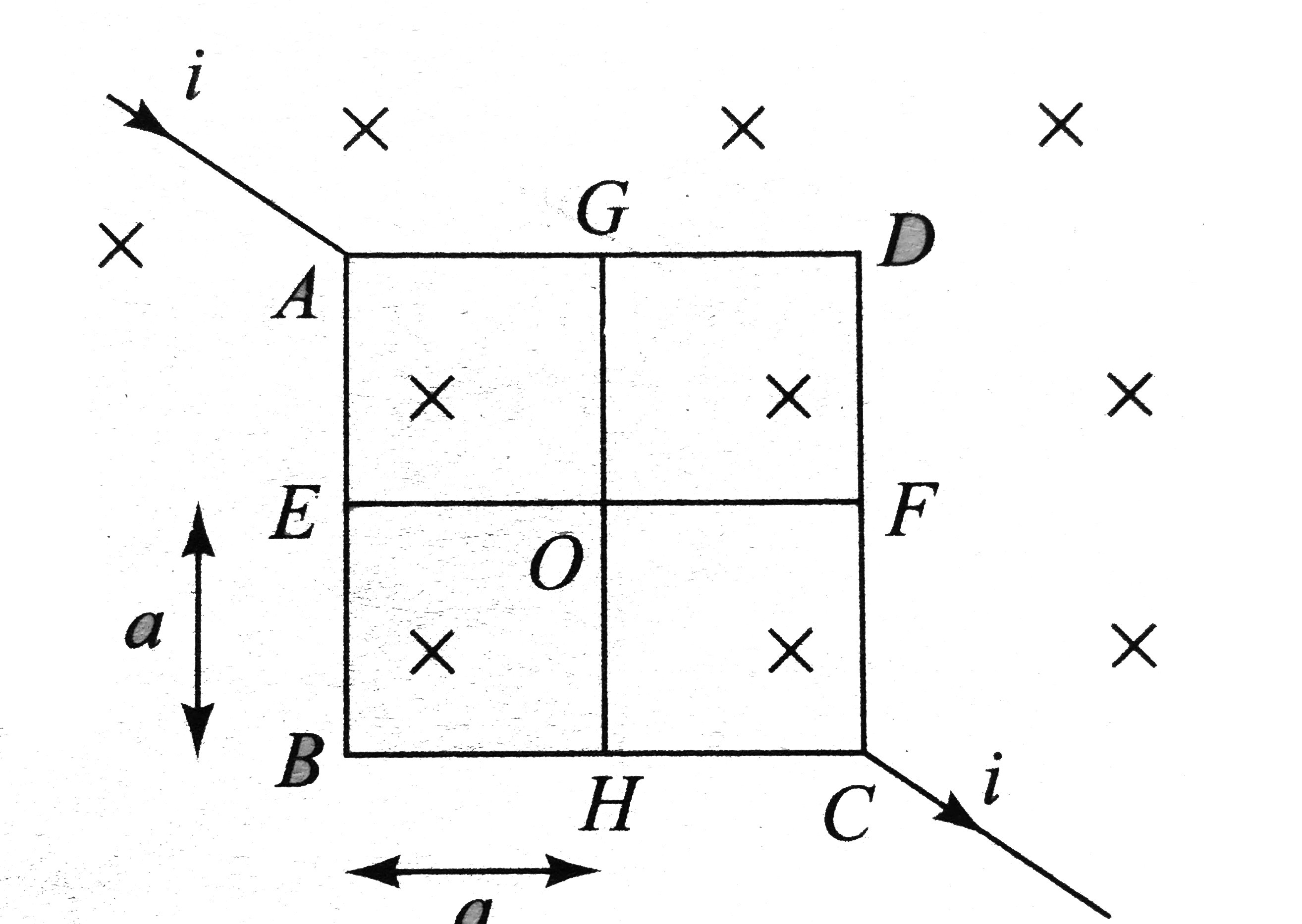 A2√2iBaB√2iBaC2iBaDiBa
A2√2iBaB√2iBaC2iBaDiBa
Show that of all the rectangles of given area, the square has the smallest perimeter.
View SolutionA 45∘−45∘−90∘ set - square has _________line / lines of symmetry
View SolutionShow that all the rectangles with a given perimeter, the square has the largest area.
View SolutionShow that all the rectangles with a given perimeter, the square has the largest area.
View SolutionShow that of all the rectangles inscribed in a given fixed circle, the square has the maximum area.
View SolutionLet N be a natural number which contains no prime factors except 2 and 3. N has exactly 24 different divisors and its square has 77 different divisors. The sum of all possible values of N is.
View Solution
